
Question Number 52683 by maxmathsup by imad last updated on 11/Jan/19
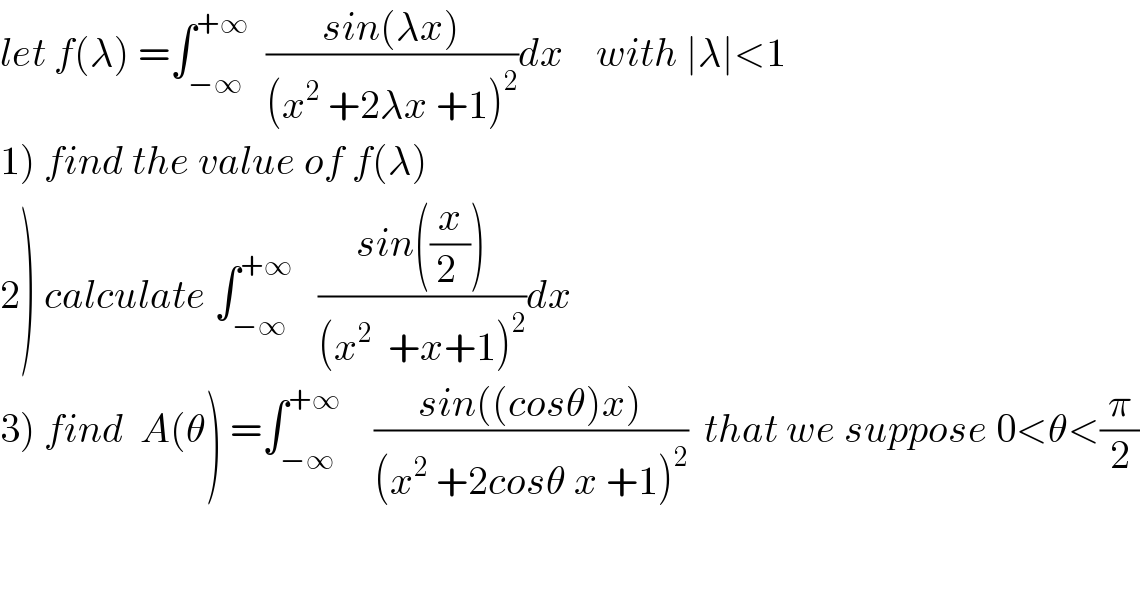
$${let}\:{f}\left(\lambda\right)\:=\int_{−\infty} ^{+\infty} \:\:\frac{{sin}\left(\lambda{x}\right)}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\lambda{x}\:+\mathrm{1}\right)^{\mathrm{2}} }{dx}\:\:\:\:{with}\:\mid\lambda\mid<\mathrm{1} \\ $$ $$\left.\mathrm{1}\right)\:{find}\:{the}\:{value}\:{of}\:{f}\left(\lambda\right) \\ $$ $$\left.\mathrm{2}\right)\:{calculate}\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{sin}\left(\frac{{x}}{\mathrm{2}\:}\right)}{\left({x}^{\mathrm{2}} \:\:+{x}+\mathrm{1}\right)^{\mathrm{2}} }{dx} \\ $$ $$\left.\mathrm{3}\right)\:{find}\:\:{A}\left(\theta\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\:\frac{{sin}\left(\left({cos}\theta\right){x}\right)}{\left({x}^{\mathrm{2}} \:+\mathrm{2}{cos}\theta\:{x}\:+\mathrm{1}\right)^{\mathrm{2}} }\:\:{that}\:{we}\:{suppose}\:\mathrm{0}<\theta<\frac{\pi}{\mathrm{2}} \\ $$
Commented bymaxmathsup by imad last updated on 13/Jan/19

$$\left.\mathrm{1}\right)\:{f}\left(\lambda\right)={Im}\left(\int_{−\infty} ^{+\infty} \:\:\:\frac{{e}^{{i}\lambda{x}} }{\left({x}^{\mathrm{2}} \:+\mathrm{2}\lambda{x}\:+\mathrm{1}\right)^{\mathrm{2}} }{dx}\right)\:{let}\:{consder}\:{the}\:{complex}\:{function} \\ $$ $$\varphi\left({z}\right)=\:\frac{{e}^{{i}\lambda{z}} }{\left({x}^{\mathrm{2}} +\mathrm{2}\lambda{x}\:+\mathrm{1}\right)^{\mathrm{2}} }\:{poles}\:{of}\:\varphi?\:\:{let}\:{determine}\:{roots}\:{of}\:{x}^{\mathrm{2}} \:+\mathrm{2}\lambda{x}\:+\mathrm{1} \\ $$ $$\Delta^{'} =\lambda^{\mathrm{2}} −\mathrm{1}<\mathrm{0}\:\Rightarrow\Delta^{'} \:=−\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\:=\left({i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right)^{\mathrm{2}} \:\Rightarrow{z}_{\mathrm{1}} =−\lambda\:+{i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} } \\ $$ $${and}\:{z}_{\mathrm{2}} =−\lambda−{i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\:\:\:\:\Rightarrow\:\varphi\:{have}\:{double}\:{poles}\:{z}_{\mathrm{1}} \:{and}\:{z}_{\mathrm{2}} \\ $$ $$\varphi\left({z}\right)\:=\frac{{e}^{{i}\lambda{z}} }{\left({z}−{z}_{\mathrm{1}} \right)^{\mathrm{2}} \left({z}−{z}_{\mathrm{2}} \right)^{\mathrm{2}} }\:\:{residus}\:{theorem}\:{give} \\ $$ $$\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left(\varphi,{z}_{\mathrm{1}} \right)\:{but} \\ $$ $${Res}\left(\varphi,{z}_{\mathrm{1}} \right)={lim}_{{z}\rightarrow{z}_{\mathrm{1}} } \:\frac{\mathrm{1}}{\left(\mathrm{2}−\mathrm{1}\right)!}\:\left\{\left({z}−{z}_{\mathrm{1}} \right)^{\mathrm{2}} \varphi\left({z}\right)\right\}^{\left(\mathrm{1}\right)} \\ $$ $$={lim}_{{z}\rightarrow{z}_{\mathrm{1}} } \:\:\:\left\{\:\frac{{e}^{{i}\lambda{z}} }{\left({z}−{z}_{\mathrm{2}} \right)^{\mathrm{2}} }\right\}^{\left(\mathrm{1}\right)} \\ $$ $$={lim}_{{z}\rightarrow{z}_{\mathrm{1}} } \:\frac{{i}\lambda\:{e}^{{i}\lambda{z}} \left({z}−{z}_{\mathrm{2}} \right)^{\mathrm{2}} \:−\mathrm{2}\left({z}−{z}_{\mathrm{2}} \right){e}^{{i}\lambda{z}} }{\left({z}−{z}_{\mathrm{2}} \right)^{\mathrm{4}} }\:={lim}_{{z}\rightarrow{z}_{\mathrm{1}} } \:\frac{\left({i}\lambda\left({z}−{z}_{\mathrm{2}} \right)−\mathrm{2}\right){e}^{{i}\lambda{z}} }{\left({z}−{z}_{\mathrm{2}} \right)^{\mathrm{3}} } \\ $$ $$=\frac{\left({i}\lambda\left({z}_{\mathrm{1}} −{z}_{\mathrm{2}} \right)−\mathrm{2}\right){e}^{{iz}_{\mathrm{1}} } }{\left({z}_{\mathrm{1}} −{z}_{\mathrm{2}} \right)^{\mathrm{3}} }\:=\frac{\left({i}\lambda\left(\mathrm{2}{i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right)−\mathrm{2}\right){e}^{{i}\lambda{z}_{\mathrm{1}} } }{\left(\mathrm{2}{i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right)^{\mathrm{3}} } \\ $$ $$=\frac{\left(−\mathrm{2}\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }−\mathrm{2}\right){e}^{{i}\lambda\left(−\lambda+{i}\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right)} }{−\mathrm{8}{i}\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:=\frac{\left(\mathrm{1}+\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right){e}^{−{i}\lambda^{\mathrm{2}} } \:{e}^{−\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }} }{\mathrm{4}{i}\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }} \\ $$ $$\Rightarrow\int_{−\infty} ^{+\infty} \varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\frac{\left(\mathrm{1}+\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right){e}^{−\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }} }{\mathrm{4}{i}\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:{e}^{−{i}\lambda^{\mathrm{2}} } \: \\ $$ $$=\frac{\pi}{\mathrm{2}}\:\frac{\left(\mathrm{1}+\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right){e}^{−\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }} }{\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:\left({cos}\left(\lambda^{\mathrm{2}} \right)−{isin}\left(\lambda^{\mathrm{2}} \right)\right)\:\Rightarrow \\ $$ $${f}\left(\lambda\right)\:=−\frac{\pi}{\mathrm{2}}\:{sin}\left(\lambda^{\mathrm{2}} \right)\:\frac{\left(\mathrm{1}+\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }\right){e}^{−\lambda\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }} }{\left(\mathrm{1}−\lambda^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:. \\ $$ $$ \\ $$
Commented bymaxmathsup by imad last updated on 13/Jan/19
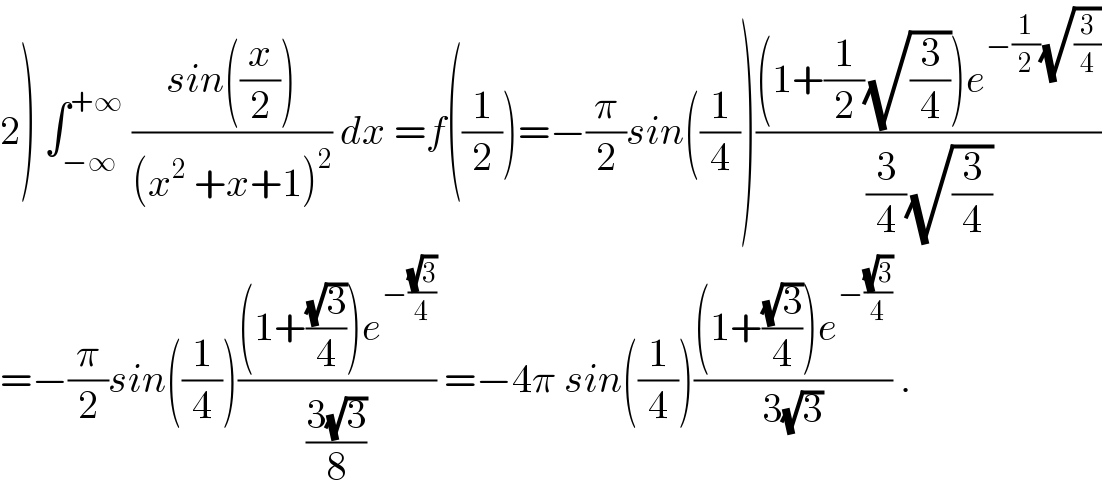
$$\left.\mathrm{2}\right)\:\int_{−\infty} ^{+\infty} \:\frac{{sin}\left(\frac{{x}}{\mathrm{2}}\right)}{\left({x}^{\mathrm{2}} \:+{x}+\mathrm{1}\right)^{\mathrm{2}} }\:{dx}\:={f}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=−\frac{\pi}{\mathrm{2}}{sin}\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\frac{\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}\right){e}^{−\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}} }{\frac{\mathrm{3}}{\mathrm{4}}\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$ $$=−\frac{\pi}{\mathrm{2}}{sin}\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\frac{\left(\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}\right){e}^{−\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}} }{\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{8}}}\:=−\mathrm{4}\pi\:{sin}\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\frac{\left(\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}\right){e}^{−\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}} }{\mathrm{3}\sqrt{\mathrm{3}}}\:. \\ $$
Commented byAbdo msup. last updated on 13/Jan/19
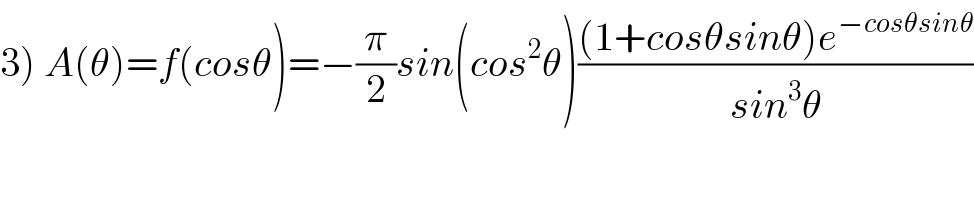
$$\left.\mathrm{3}\right)\:{A}\left(\theta\right)={f}\left({cos}\theta\right)=−\frac{\pi}{\mathrm{2}}{sin}\left({cos}^{\mathrm{2}} \theta\right)\frac{\left(\mathrm{1}+{cos}\theta{sin}\theta\right){e}^{−{cos}\theta{sin}\theta} }{{sin}^{\mathrm{3}} \theta} \\ $$
