
Question Number 51122 by behi83417@gmail.com last updated on 24/Dec/18
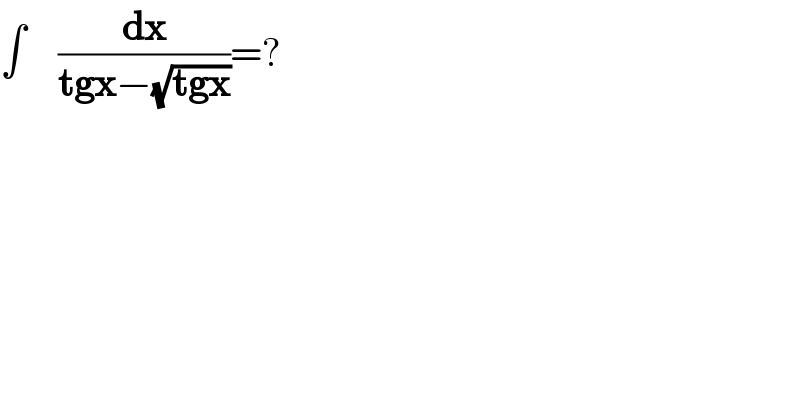
$$\int\:\:\:\:\frac{\boldsymbol{\mathrm{dx}}}{\boldsymbol{\mathrm{tgx}}−\sqrt{\boldsymbol{\mathrm{tgx}}}}=? \\ $$
Commented by MJS last updated on 24/Dec/18
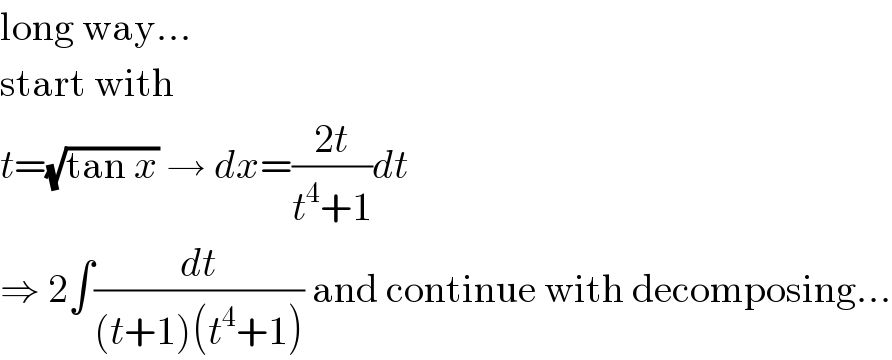
$$\mathrm{long}\:\mathrm{way}... \\ $$$$\mathrm{start}\:\mathrm{with} \\ $$$${t}=\sqrt{\mathrm{tan}\:{x}}\:\rightarrow\:{dx}=\frac{\mathrm{2}{t}}{{t}^{\mathrm{4}} +\mathrm{1}}{dt} \\ $$$$\Rightarrow\:\mathrm{2}\int\frac{{dt}}{\left({t}+\mathrm{1}\right)\left({t}^{\mathrm{4}} +\mathrm{1}\right)}\:\mathrm{and}\:\mathrm{continue}\:\mathrm{with}\:\mathrm{decomposing}... \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 24/Dec/18
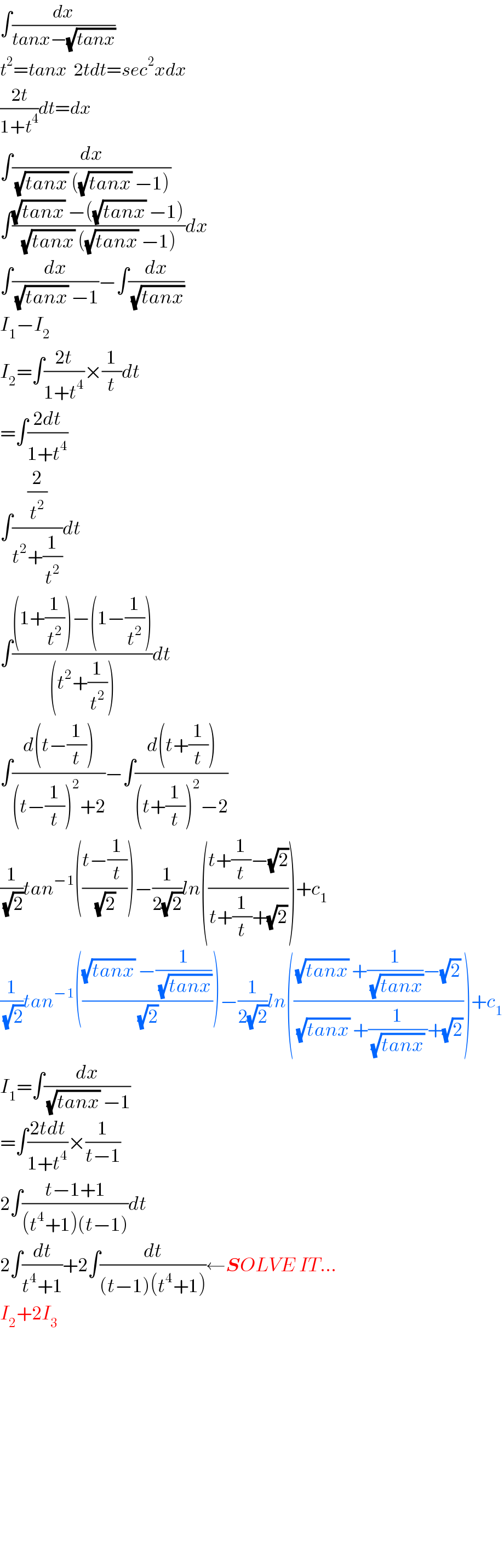
$$\int\frac{{dx}}{{tanx}−\sqrt{{tanx}}} \\ $$$${t}^{\mathrm{2}} ={tanx}\:\:\mathrm{2}{tdt}={sec}^{\mathrm{2}} {xdx} \\ $$$$\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}={dx} \\ $$$$\int\frac{{dx}}{\sqrt{{tanx}}\:\left(\sqrt{{tanx}}\:−\mathrm{1}\right)} \\ $$$$\int\frac{\sqrt{{tanx}}\:−\left(\sqrt{{tanx}}\:−\mathrm{1}\right)}{\sqrt{{tanx}}\:\left(\sqrt{{tanx}}\:−\mathrm{1}\right)}{dx} \\ $$$$\int\frac{{dx}}{\sqrt{{tanx}}\:−\mathrm{1}}−\int\frac{{dx}}{\sqrt{{tanx}}} \\ $$$${I}_{\mathrm{1}} −{I}_{\mathrm{2}} \\ $$$${I}_{\mathrm{2}} =\int\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{4}} }×\frac{\mathrm{1}}{{t}}{dt} \\ $$$$=\int\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{4}} } \\ $$$$\int\frac{\frac{\mathrm{2}}{{t}^{\mathrm{2}} }}{{t}^{\mathrm{2}} +\frac{\mathrm{1}}{{t}^{\mathrm{2}} }}{dt} \\ $$$$\int\frac{\left(\mathrm{1}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} }\right)−\left(\mathrm{1}−\frac{\mathrm{1}}{{t}^{\mathrm{2}} }\right)}{\left({t}^{\mathrm{2}} +\frac{\mathrm{1}}{{t}^{\mathrm{2}} }\right)}{dt} \\ $$$$\int\frac{{d}\left({t}−\frac{\mathrm{1}}{{t}}\right)}{\left({t}−\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} +\mathrm{2}}−\int\frac{{d}\left({t}+\frac{\mathrm{1}}{{t}}\right)}{\left({t}+\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} −\mathrm{2}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{tan}^{−\mathrm{1}} \left(\frac{{t}−\frac{\mathrm{1}}{{t}}}{\sqrt{\mathrm{2}}}\right)−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{ln}\left(\frac{{t}+\frac{\mathrm{1}}{{t}}−\sqrt{\mathrm{2}}}{{t}+\frac{\mathrm{1}}{{t}}+\sqrt{\mathrm{2}}}\right)+{c}_{\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{tan}^{−\mathrm{1}} \left(\frac{\sqrt{{tanx}}\:−\frac{\mathrm{1}}{\sqrt{{tanx}}}}{\sqrt{\mathrm{2}}}\right)−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{ln}\left(\frac{\sqrt{{tanx}}\:+\frac{\mathrm{1}}{\sqrt{{tanx}}}−\sqrt{\mathrm{2}}}{\sqrt{{tanx}}\:+\frac{\mathrm{1}}{\sqrt{{tanx}}\:}+\sqrt{\mathrm{2}}}\right)+{c}_{\mathrm{1}} \\ $$$${I}_{\mathrm{1}} =\int\frac{{dx}}{\sqrt{{tanx}}\:−\mathrm{1}} \\ $$$$=\int\frac{\mathrm{2}{tdt}}{\mathrm{1}+{t}^{\mathrm{4}} }×\frac{\mathrm{1}}{{t}−\mathrm{1}} \\ $$$$\mathrm{2}\int\frac{{t}−\mathrm{1}+\mathrm{1}}{\left({t}^{\mathrm{4}} +\mathrm{1}\right)\left({t}−\mathrm{1}\right)}{dt} \\ $$$$\mathrm{2}\int\frac{{dt}}{{t}^{\mathrm{4}} +\mathrm{1}}+\mathrm{2}\int\frac{{dt}}{\left({t}−\mathrm{1}\right)\left({t}^{\mathrm{4}} +\mathrm{1}\right)}\leftarrow\boldsymbol{{S}}{OLVE}\:{IT}... \\ $$$${I}_{\mathrm{2}} +\mathrm{2}{I}_{\mathrm{3}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by behi83417@gmail.com last updated on 25/Dec/18

$${thank}\:{you}\:{sir}\:{tanmay}. \\ $$
