
Question Number 4889 by 123456 last updated on 19/Mar/16
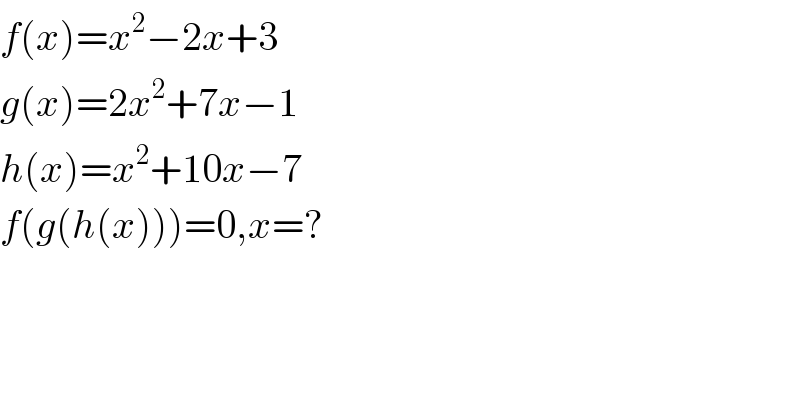
$${f}\left({x}\right)={x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{3} \\ $$$${g}\left({x}\right)=\mathrm{2}{x}^{\mathrm{2}} +\mathrm{7}{x}−\mathrm{1} \\ $$$${h}\left({x}\right)={x}^{\mathrm{2}} +\mathrm{10}{x}−\mathrm{7} \\ $$$${f}\left({g}\left({h}\left({x}\right)\right)\right)=\mathrm{0},{x}=? \\ $$
Answered by Yozzii last updated on 19/Mar/16

$${f}\left({g}\left({h}\left({x}\right)\right)\right)=\mathrm{0}.\:{Let}\:{u}={g}\left({h}\left({x}\right)\right). \\ $$$$\therefore\:{f}\left({u}\right)=\mathrm{0}\Rightarrow{u}^{\mathrm{2}} −\mathrm{2}{u}+\mathrm{3}=\mathrm{0} \\ $$$$\therefore\:{u}=\frac{\mathrm{2}\pm\sqrt{\mathrm{2}^{\mathrm{2}} −\mathrm{4}×\mathrm{1}×\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{2}\pm\mathrm{2}\sqrt{−\mathrm{2}}}{\mathrm{2}} \\ $$$${u}=\mathrm{1}\pm{i}\sqrt{\mathrm{2}}\:,\:{u}_{\mathrm{1}} =\mathrm{1}+{i}\sqrt{\mathrm{2}},{u}_{\mathrm{2}} =\mathrm{1}−{i}\sqrt{\mathrm{2}}. \\ $$$$\therefore{g}\left({h}\left({x}\right)\right)={u}_{{r}} \:\:\:\:\:\left({r}=\mathrm{1},\mathrm{2}\right) \\ $$$${Let}\:{n}={h}\left({x}\right).\therefore\:{g}\left({n}\right)={u}_{{r}} . \\ $$$$\mathrm{2}{n}^{\mathrm{2}} +\mathrm{7}{n}−\mathrm{1}={u}_{{r}} \\ $$$$\mathrm{2}{n}^{\mathrm{2}} +\mathrm{7}{n}−\mathrm{1}−{u}_{{r}} =\mathrm{0} \\ $$$${n}=\frac{−\mathrm{7}\pm\sqrt{\mathrm{49}−\mathrm{4}×\mathrm{2}\left(−\mathrm{1}−{u}_{{r}} \right)}}{\mathrm{4}} \\ $$$${n}=\frac{−\mathrm{7}\pm\sqrt{\mathrm{57}+\mathrm{8}{u}_{{r}} }}{\mathrm{4}} \\ $$$$\therefore\:{n}_{\mathrm{1}} =\frac{−\mathrm{7}+\sqrt{\mathrm{57}+\mathrm{8}{u}_{{r}} }}{\mathrm{4}},{n}_{\mathrm{2}} =\frac{−\mathrm{7}−\sqrt{\mathrm{57}+\mathrm{8}{u}_{{r}} }}{\mathrm{4}} \\ $$$${r}=\mathrm{1},\mathrm{2}.\:\left(\mathrm{4}\:{roots}\:{for}\:{n}\right) \\ $$$$\therefore\:{h}\left({x}\right)={n}_{{j}} \:\:\left({j}=\mathrm{1},\mathrm{2}\right) \\ $$$${x}^{\mathrm{2}} +\mathrm{10}{x}−\mathrm{7}={n}_{{j}} \\ $$$${x}=\frac{−\mathrm{10}\pm\sqrt{\mathrm{10}^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}\right)\left(−\mathrm{7}−{n}_{{j}} \right)}}{\mathrm{2}} \\ $$$${x}=\frac{−\mathrm{10}\pm\sqrt{\mathrm{4}×\mathrm{25}+\mathrm{4}\left(\mathrm{7}+{n}_{{j}} \right)}}{\mathrm{2}} \\ $$$${x}_{{j}} =−\mathrm{5}\pm\sqrt{\mathrm{32}+{n}_{{j}} } \\ $$$${x}_{\mathrm{1},{j}} =−\mathrm{5}+\sqrt{\mathrm{32}+{n}_{{j}} }\:,{x}_{\mathrm{2},{j}} =−\mathrm{5}−\sqrt{\mathrm{32}+{n}_{{j}} } \\ $$$${x}_{\mathrm{1},{r}} =−\mathrm{5}+\sqrt{\mathrm{32}+\frac{−\mathrm{7}\pm\sqrt{\mathrm{57}+\mathrm{8}{u}_{{r}} }}{\mathrm{4}}} \\ $$$${x}_{\mathrm{1}} =−\mathrm{5}+\sqrt{\mathrm{32}+\frac{−\mathrm{7}+\sqrt{\left.\mathrm{65}+{i}\mathrm{8}\sqrt{\mathrm{2}}\right)}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{2}} =−\mathrm{5}+\sqrt{\mathrm{32}+\frac{−\mathrm{7}+\sqrt{\mathrm{65}−\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{3}} =−\mathrm{5}+\sqrt{\mathrm{32}+\frac{−\mathrm{7}−\sqrt{\mathrm{65}−\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{4}} =−\mathrm{5}+\sqrt{\mathrm{32}+\frac{−\mathrm{7}−\sqrt{\mathrm{65}+\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$$−−−−−−−−−−−−−−−−−−− \\ $$$${x}_{\mathrm{2},{r}} =−\mathrm{5}−\sqrt{\mathrm{32}+\frac{−\mathrm{7}\pm\sqrt{\mathrm{57}+\mathrm{8}{u}_{{r}} }}{\mathrm{4}}} \\ $$$${x}_{\mathrm{5}} =−\mathrm{5}−\sqrt{\mathrm{32}+\frac{−\mathrm{7}+\sqrt{\mathrm{65}+\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{6}} =−\mathrm{5}−\sqrt{\mathrm{32}+\frac{−\mathrm{7}+\sqrt{\mathrm{65}−\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{7}} =−\mathrm{5}−\sqrt{\mathrm{32}+\frac{−\mathrm{7}−\sqrt{\mathrm{65}−\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}} \\ $$$${x}_{\mathrm{8}} =−\mathrm{5}−\sqrt{\mathrm{32}+\frac{−\mathrm{7}−\sqrt{\mathrm{65}+\mathrm{8}{i}\sqrt{\mathrm{2}}}}{\mathrm{4}}}. \\ $$$$ \\ $$$$ \\ $$
