
Question Number 48553 by Cheyboy last updated on 25/Nov/18

$$\mathrm{In}\:\mathrm{a}\:\mathrm{hospital}\:\mathrm{unit},\mathrm{there}\:\mathrm{are}\:\mathrm{8}\:\mathrm{nurses} \\ $$$$\mathrm{and}\:\mathrm{5}\:\mathrm{physicians}.\:\mathrm{7}\:\mathrm{of}\:\mathrm{them}\:\mathrm{are} \\ $$$$\mathrm{female}\:\mathrm{nurses}\:\mathrm{and}\:\mathrm{3}\:\mathrm{of}\:\mathrm{them}\:\mathrm{are} \\ $$$$\mathrm{male}\:\mathrm{physicians}. \\ $$$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{of} \\ $$$$\mathrm{selecting}\:\mathrm{a}\:\mathrm{staff}\:\mathrm{who}\:\mathrm{is}\:\mathrm{Nurse}\:\mathrm{or} \\ $$$$\mathrm{Male}? \\ $$$$\mathrm{plzz}\:\mathrm{help} \\ $$$$ \\ $$$$ \\ $$
Commented by mr W last updated on 26/Nov/18
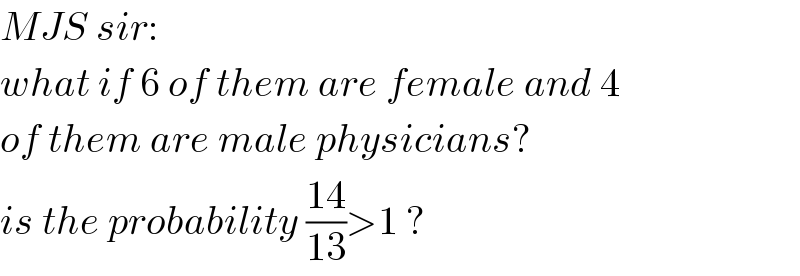
$${MJS}\:{sir}: \\ $$$${what}\:{if}\:\mathrm{6}\:{of}\:{them}\:{are}\:{female}\:{and}\:\mathrm{4} \\ $$$${of}\:{them}\:{are}\:{male}\:{physicians}? \\ $$$${is}\:{the}\:{probability}\:\frac{\mathrm{14}}{\mathrm{13}}>\mathrm{1}\:? \\ $$
Commented by MJS last updated on 26/Nov/18

$$\mathrm{8}\:\mathrm{nurses} \\ $$$$\:\:\:\:\:\mathrm{7}\:\mathrm{female}\:\Rightarrow\:\mathrm{1}\:\mathrm{male} \\ $$$$\mathrm{5}\:\mathrm{physicians} \\ $$$$\:\:\:\:\:\mathrm{3}\:\mathrm{male}\:\Rightarrow\:\mathrm{2}\:\mathrm{female} \\ $$$$\Rightarrow\:\mathrm{probabikity}\:\frac{\mathrm{11}}{\mathrm{12}} \\ $$$$\mathrm{sorry}\:\mathrm{for}\:\mathrm{mistake}\:\mathrm{yesterday} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{8}\:\mathrm{nurses}\:+\:\mathrm{5}\:\mathrm{physicians} \\ $$$$\:\:\:\:\:\mathrm{6}\:\mathrm{female}\:\Rightarrow\:\mathrm{7}\:\mathrm{male} \\ $$$$\mathrm{5}\:\mathrm{physicians} \\ $$$$\:\:\:\:\:\mathrm{4}\:\mathrm{male}\:\Rightarrow\:\mathrm{1}\:\mathrm{female} \\ $$$$\Rightarrow\:\mathrm{8}\:\mathrm{nurses} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{5}\:\mathrm{female}\:\Rightarrow\:\mathrm{3}\:\mathrm{male} \\ $$$$\mathrm{probability}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\frac{\mathrm{12}}{\mathrm{13}}\:\mathrm{because}\:\mathrm{we}\:\mathrm{must} \\ $$$$\mathrm{count}\:\mathrm{all}\:\mathrm{nurses}\:\mathrm{anyway},\:\mathrm{plus}\:\mathrm{only}\:\mathrm{male}\:\mathrm{physicians} \\ $$
Answered by MJS last updated on 26/Nov/18
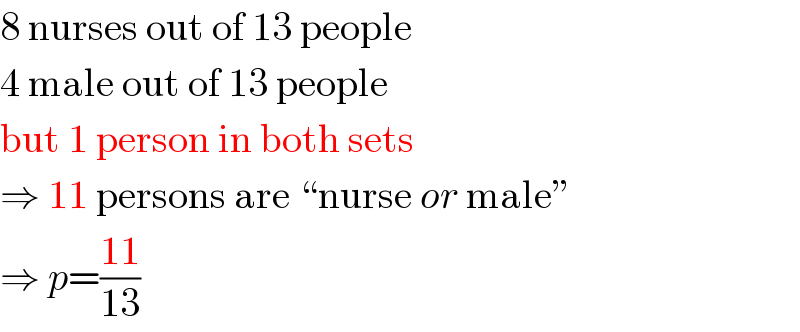
$$\mathrm{8}\:\mathrm{nurses}\:\mathrm{out}\:\mathrm{of}\:\mathrm{13}\:\mathrm{people} \\ $$$$\mathrm{4}\:\mathrm{male}\:\mathrm{out}\:\mathrm{of}\:\mathrm{13}\:\mathrm{people} \\ $$$$\mathrm{but}\:\mathrm{1}\:\mathrm{person}\:\mathrm{in}\:\mathrm{both}\:\mathrm{sets} \\ $$$$\Rightarrow\:\mathrm{11}\:\mathrm{persons}\:\mathrm{are}\:``\mathrm{nurse}\:{or}\:\mathrm{male}'' \\ $$$$\Rightarrow\:{p}=\frac{\mathrm{11}}{\mathrm{13}} \\ $$
Commented by Cheyboy last updated on 25/Nov/18

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
