
Question Number 48541 by behi83417@gmail.com last updated on 25/Nov/18
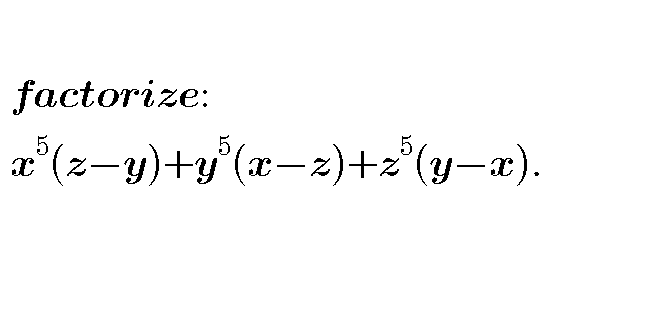
Commented by math1967 last updated on 25/Nov/18
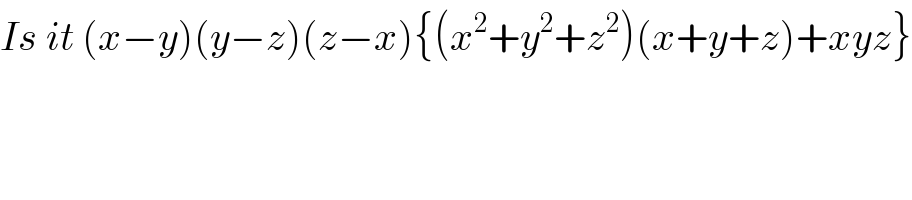
$${Is}\:{it}\:\left({x}−{y}\right)\left({y}−{z}\right)\left({z}−{x}\right)\left\{\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)\left({x}+{y}+{z}\right)+{xyz}\right\} \\ $$
Commented by math1967 last updated on 25/Nov/18
$${Apnake}\:{onek}\:{dhanyabad} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 25/Nov/18

$${excellent}...{sir}... \\ $$
Commented by math1967 last updated on 25/Nov/18
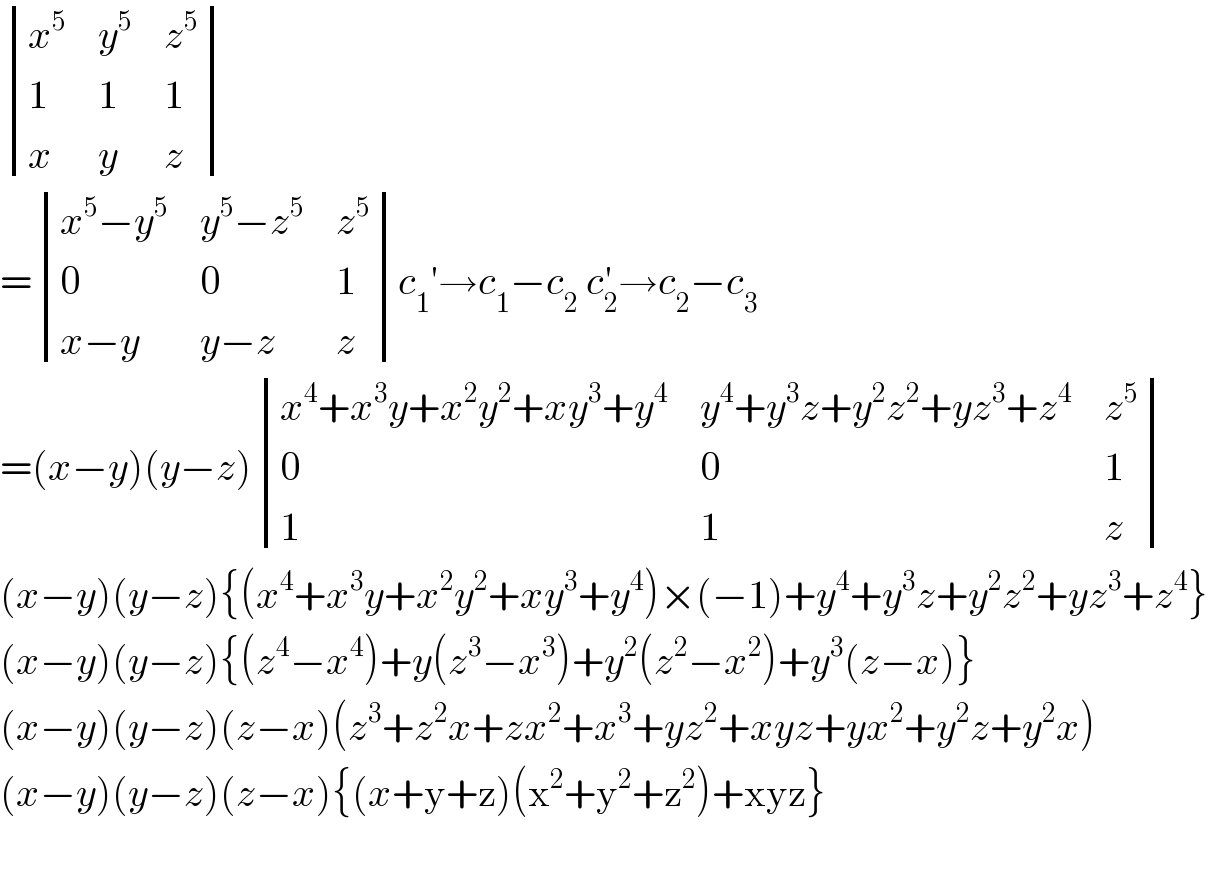
$$\begin{vmatrix}{{x}^{\mathrm{5}} }&{{y}^{\mathrm{5}} }&{{z}^{\mathrm{5}} }\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{1}}\\{{x}}&{{y}}&{{z}}\end{vmatrix} \\ $$$$=\begin{vmatrix}{{x}^{\mathrm{5}} −{y}^{\mathrm{5}} }&{{y}^{\mathrm{5}} −{z}^{\mathrm{5}} }&{{z}^{\mathrm{5}} }\\{\mathrm{0}}&{\mathrm{0}}&{\mathrm{1}}\\{{x}−{y}}&{{y}−{z}}&{{z}}\end{vmatrix}{c}_{\mathrm{1}} '\rightarrow{c}_{\mathrm{1}} −{c}_{\mathrm{2}} \:{c}_{\mathrm{2}} ^{'} \rightarrow{c}_{\mathrm{2}} −{c}_{\mathrm{3}} \\ $$$$=\left({x}−{y}\right)\left({y}−{z}\right)\begin{vmatrix}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} {y}+{x}^{\mathrm{2}} {y}^{\mathrm{2}} +{xy}^{\mathrm{3}} +{y}^{\mathrm{4}} }&{{y}^{\mathrm{4}} +{y}^{\mathrm{3}} {z}+{y}^{\mathrm{2}} {z}^{\mathrm{2}} +{yz}^{\mathrm{3}} +{z}^{\mathrm{4}} }&{{z}^{\mathrm{5}} }\\{\mathrm{0}}&{\mathrm{0}}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{1}}&{{z}}\end{vmatrix} \\ $$$$\left({x}−{y}\right)\left({y}−{z}\right)\left\{\left({x}^{\mathrm{4}} +{x}^{\mathrm{3}} {y}+{x}^{\mathrm{2}} {y}^{\mathrm{2}} +{xy}^{\mathrm{3}} +{y}^{\mathrm{4}} \right)×\left(−\mathrm{1}\right)+{y}^{\mathrm{4}} +{y}^{\mathrm{3}} {z}+{y}^{\mathrm{2}} {z}^{\mathrm{2}} +{yz}^{\mathrm{3}} +{z}^{\mathrm{4}} \right\} \\ $$$$\left({x}−{y}\right)\left({y}−{z}\right)\left\{\left({z}^{\mathrm{4}} −{x}^{\mathrm{4}} \right)+{y}\left({z}^{\mathrm{3}} −{x}^{\mathrm{3}} \right)+{y}^{\mathrm{2}} \left({z}^{\mathrm{2}} −{x}^{\mathrm{2}} \right)+{y}^{\mathrm{3}} \left({z}−{x}\right)\right\} \\ $$$$\left({x}−{y}\right)\left({y}−{z}\right)\left({z}−{x}\right)\left({z}^{\mathrm{3}} +{z}^{\mathrm{2}} {x}+{zx}^{\mathrm{2}} +{x}^{\mathrm{3}} +{yz}^{\mathrm{2}} +{xyz}+{yx}^{\mathrm{2}} +{y}^{\mathrm{2}} {z}+{y}^{\mathrm{2}} {x}\right) \\ $$$$\left({x}−{y}\right)\left({y}−{z}\right)\left({z}−{x}\right)\left\{\left({x}+\mathrm{y}+\mathrm{z}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)+\mathrm{xyz}\right\} \\ $$$$ \\ $$
Commented by math1967 last updated on 25/Nov/18

$${Thank}\:{yousir} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 25/Nov/18

$${Apni}\:{ki}\:{bangali}...{ami}\:{bangali}...{ki}\:{nam}\:{apnar}.. \\ $$
Commented by math1967 last updated on 25/Nov/18
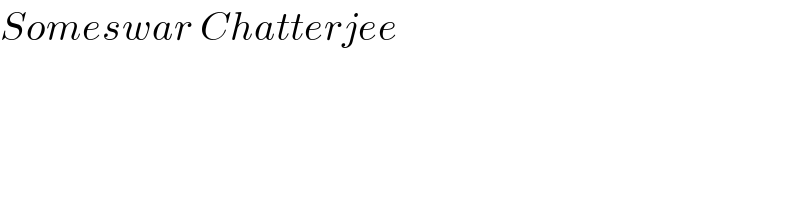
$${Someswar}\:{Chatterjee} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 25/Nov/18
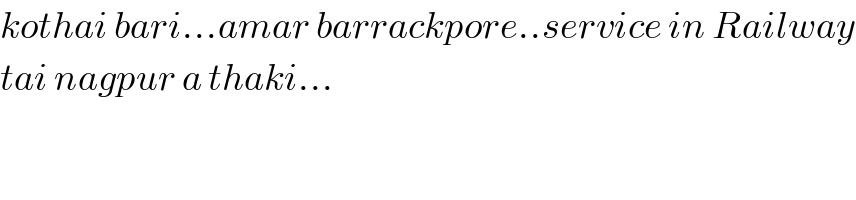
$${kothai}\:{bari}...{amar}\:{barrackpore}..{service}\:{in}\:{Railway} \\ $$$${tai}\:{nagpur}\:{a}\:{thaki}... \\ $$
Commented by behi83417@gmail.com last updated on 25/Nov/18
![let:f(x,y,z)=x^5 (z−y)+y^5 (x−z)+z^5 (y−x) when x=y or y=z or z=x⇒f(x,y,z)=0 so: z−y,y−x,x−z,are the factors of f(x,y,z). by dividing we have: f(x,y,z)=(y−x)(x−z)(z−y)[xyz+x^3 + +y^3 +z^3 +x^2 y+y^2 x+z^2 y+y^2 z+x^2 z+z^2 x]= =(y−x)(x−z)(z−y)[xyz+Σx^3 +Σx^2 y].](Q48600.png)
$${let}:{f}\left({x},{y},{z}\right)={x}^{\mathrm{5}} \left({z}−{y}\right)+{y}^{\mathrm{5}} \left({x}−{z}\right)+{z}^{\mathrm{5}} \left({y}−{x}\right) \\ $$$${when}\:{x}={y}\:{or}\:{y}={z}\:{or}\:{z}={x}\Rightarrow{f}\left({x},{y},{z}\right)=\mathrm{0} \\ $$$${so}:\:{z}−{y},{y}−{x},{x}−{z},{are}\:{the}\:{factors}\:{of}\: \\ $$$${f}\left({x},{y},{z}\right). \\ $$$${by}\:{dividing}\:{we}\:{have}: \\ $$$${f}\left({x},{y},{z}\right)=\left({y}−{x}\right)\left({x}−{z}\right)\left({z}−{y}\right)\left[{xyz}+{x}^{\mathrm{3}} +\right. \\ $$$$\left.+{y}^{\mathrm{3}} +{z}^{\mathrm{3}} +{x}^{\mathrm{2}} {y}+{y}^{\mathrm{2}} {x}+{z}^{\mathrm{2}} {y}+{y}^{\mathrm{2}} {z}+{x}^{\mathrm{2}} {z}+{z}^{\mathrm{2}} {x}\right]= \\ $$$$=\left({y}−{x}\right)\left({x}−{z}\right)\left({z}−{y}\right)\left[{xyz}+\Sigma{x}^{\mathrm{3}} +\Sigma{x}^{\mathrm{2}} {y}\right]. \\ $$
Commented by math1967 last updated on 26/Nov/18
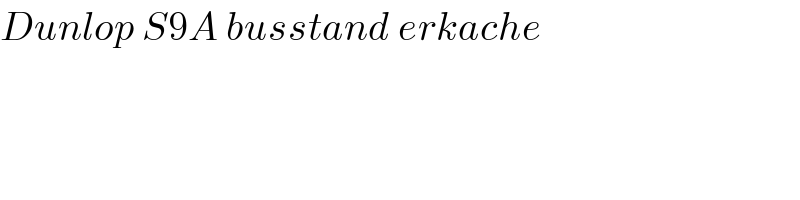
$${Dunlop}\:{S}\mathrm{9}{A}\:{busstand}\:{erkache} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 26/Nov/18

$${bah}\:{darun}\:{ar}\:{por}\:{zokhon}\:{barrackpore}\:{jabo}\:\:{contact}\:{korbo} \\ $$$${ar}\:{Dakhsinesswar}\:{mandir}\:{jabo}... \\ $$
Commented by math1967 last updated on 26/Nov/18

$${ok} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 25/Nov/18
![S=x^5 (z−y)+y^5 (x−z)+z^5 (y−x) put x=y S=0 similarly put y=z S=0 x=z S=0 (x−y)(y−z)(z−x)[f(x,y,z) of degree 3]=S S=(x−y)(y−z)(z−x)[a(x^3 +y^3 +z^3 )+b(xyz)+c{x^2 (y+z)+y^2 (x+z)+z^2 (x+y)}]](Q48544.png)
$${S}={x}^{\mathrm{5}} \left({z}−{y}\right)+{y}^{\mathrm{5}} \left({x}−{z}\right)+{z}^{\mathrm{5}} \left({y}−{x}\right) \\ $$$${put}\:{x}={y}\:\:{S}=\mathrm{0} \\ $$$${similarly}\:{put}\:{y}={z}\:\:\:{S}=\mathrm{0} \\ $$$${x}={z}\:\:\:{S}=\mathrm{0} \\ $$$$\left({x}−{y}\right)\left({y}−{z}\right)\left({z}−{x}\right)\left[{f}\left({x},{y},{z}\right)\:{of}\:{degree}\:\mathrm{3}\right]={S} \\ $$$${S}=\left({x}−{y}\right)\left({y}−{z}\right)\left({z}−{x}\right)\left[{a}\left({x}^{\mathrm{3}} +{y}^{\mathrm{3}} +{z}^{\mathrm{3}} \right)+{b}\left({xyz}\right)+{c}\left\{{x}^{\mathrm{2}} \left({y}+{z}\right)+{y}^{\mathrm{2}} \left({x}+{z}\right)+{z}^{\mathrm{2}} \left({x}+{y}\right)\right\}\right] \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by behi83417@gmail.com last updated on 25/Nov/18
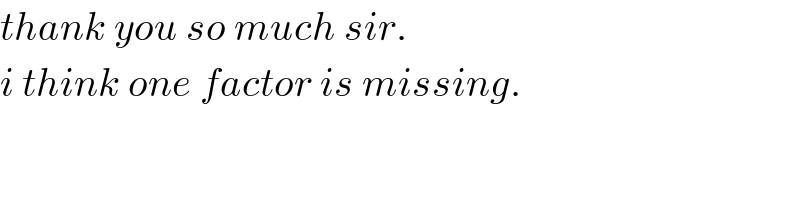
$${thank}\:{you}\:{so}\:{much}\:{sir}. \\ $$$${i}\:{think}\:{one}\:{factor}\:{is}\:{missing}. \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 25/Nov/18

$${yes}\:{sir}\:...{i}\:{have}\:{rectified}... \\ $$
