
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 47656 by Necxx last updated on 12/Nov/18

$${A}\:{square}\:{is}\:{divided}\:{into}\:\mathrm{9}\:{identical} \\ $$$${smaller}\:{squares}.{Six}\:{identical}\:{balls} \\ $$$${are}\:{to}\:{be}\:{placed}\:{in}\:{these}\:{smaller}\: \\ $$$${squares}\:{such}\:{that}\:{each}\:{of}\:{the}\:{three} \\ $$$${rows}\:{gets}\:{at}\:{least}\:{one}\:{ball}\left({one}\right. \\ $$$$\left.{ball}\:{in}\:{one}\:{square}\:{only}\right).{In}\:{how} \\ $$$${many}\:{different}\:{ways}\:{can}\:{this}\:{be} \\ $$$${done}? \\ $$$$\left.{a}\left.\right)\left.\mathrm{9}\left.\mathrm{1}\:{b}\right)\mathrm{51}\:{c}\right)\mathrm{81}\:{d}\right)\mathrm{41} \\ $$$$ \\ $$
Commented by Necxx last updated on 13/Nov/18

$${please}\:{help} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 13/Nov/18
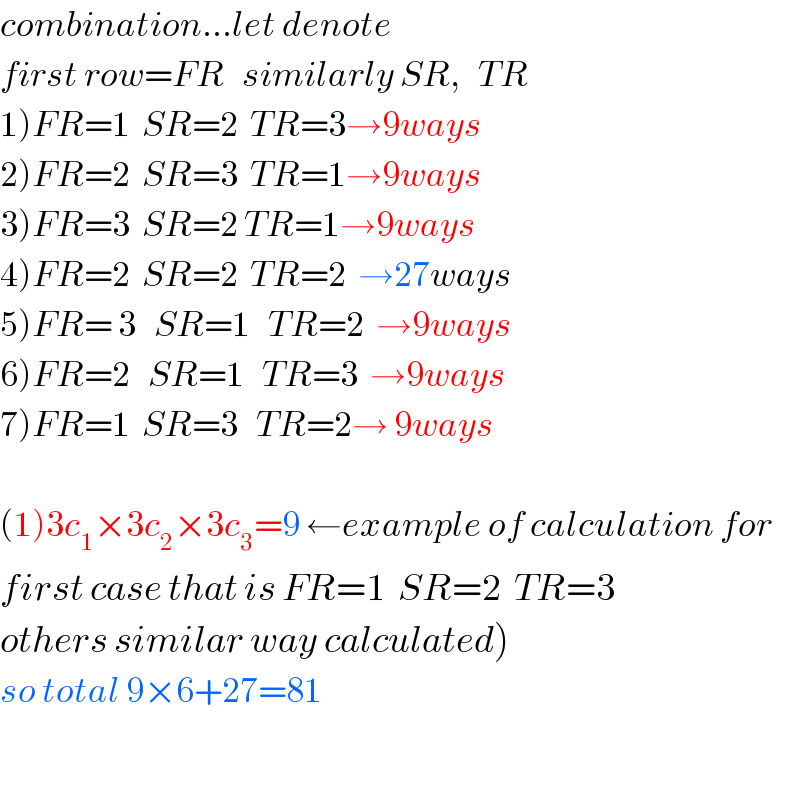
$${combination}...{let}\:{denote} \\ $$$${first}\:{row}={FR}\:\:\:{similarly}\:{SR},\:\:\:{TR} \\ $$$$\left.\mathrm{1}\right){FR}=\mathrm{1}\:\:{SR}=\mathrm{2}\:\:{TR}=\mathrm{3}\rightarrow\mathrm{9}{ways} \\ $$$$\left.\mathrm{2}\right){FR}=\mathrm{2}\:\:{SR}=\mathrm{3}\:\:{TR}=\mathrm{1}\rightarrow\mathrm{9}{ways} \\ $$$$\left.\mathrm{3}\right){FR}=\mathrm{3}\:\:{SR}=\mathrm{2}\:{TR}=\mathrm{1}\rightarrow\mathrm{9}{ways} \\ $$$$\left.\mathrm{4}\right){FR}=\mathrm{2}\:\:{SR}=\mathrm{2}\:\:{TR}=\mathrm{2}\:\:\rightarrow\mathrm{27}{ways} \\ $$$$\left.\mathrm{5}\right){FR}=\:\mathrm{3}\:\:\:{SR}=\mathrm{1}\:\:\:{TR}=\mathrm{2}\:\:\rightarrow\mathrm{9}{ways} \\ $$$$\left.\mathrm{6}\right){FR}=\mathrm{2}\:\:\:{SR}=\mathrm{1}\:\:\:{TR}=\mathrm{3}\:\:\rightarrow\mathrm{9}{ways} \\ $$$$\left.\mathrm{7}\right){FR}=\mathrm{1}\:\:{SR}=\mathrm{3}\:\:\:{TR}=\mathrm{2}\rightarrow\:\mathrm{9}{ways} \\ $$$$ \\ $$$$\left(\mathrm{1}\right)\mathrm{3}{c}_{\mathrm{1}} ×\mathrm{3}{c}_{\mathrm{2}} ×\mathrm{3}{c}_{\mathrm{3}} =\mathrm{9}\:\leftarrow{example}\:{of}\:{calculation}\:{for} \\ $$$${first}\:{case}\:{that}\:{is}\:{FR}=\mathrm{1}\:\:{SR}=\mathrm{2}\:\:{TR}=\mathrm{3} \\ $$$$\left.{others}\:{similar}\:{way}\:{calculated}\right) \\ $$$${so}\:{total}\:\mathrm{9}×\mathrm{6}+\mathrm{27}=\mathrm{81} \\ $$$$ \\ $$
Commented by Necxx last updated on 13/Nov/18
$${wow}.....{Thank}\:{you}\:{so}\:{much}. \\ $$
