
Question Number 47477 by naka3546 last updated on 10/Nov/18
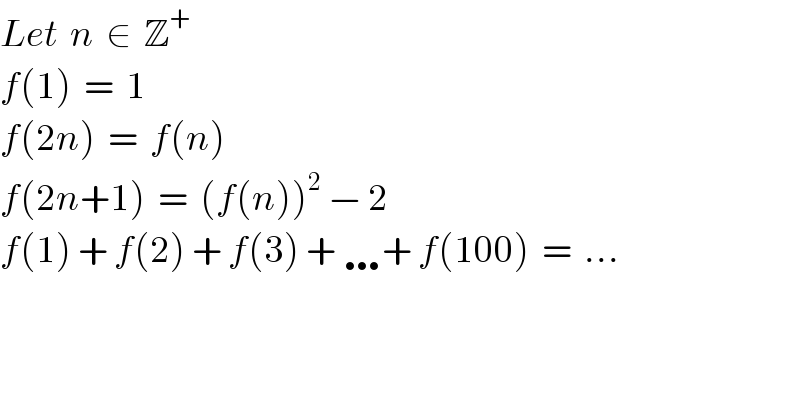
$${Let}\:\:{n}\:\:\in\:\:\mathbb{Z}^{+} \\ $$$${f}\left(\mathrm{1}\right)\:\:=\:\:\mathrm{1} \\ $$$${f}\left(\mathrm{2}{n}\right)\:\:=\:\:{f}\left({n}\right) \\ $$$${f}\left(\mathrm{2}{n}+\mathrm{1}\right)\:\:=\:\:\left({f}\left({n}\right)\right)^{\mathrm{2}} \:−\:\mathrm{2} \\ $$$${f}\left(\mathrm{1}\right)\:+\:{f}\left(\mathrm{2}\right)\:+\:{f}\left(\mathrm{3}\right)\:+\:\ldots+\:{f}\left(\mathrm{100}\right)\:\:=\:\:... \\ $$
Answered by MJS last updated on 11/Nov/18
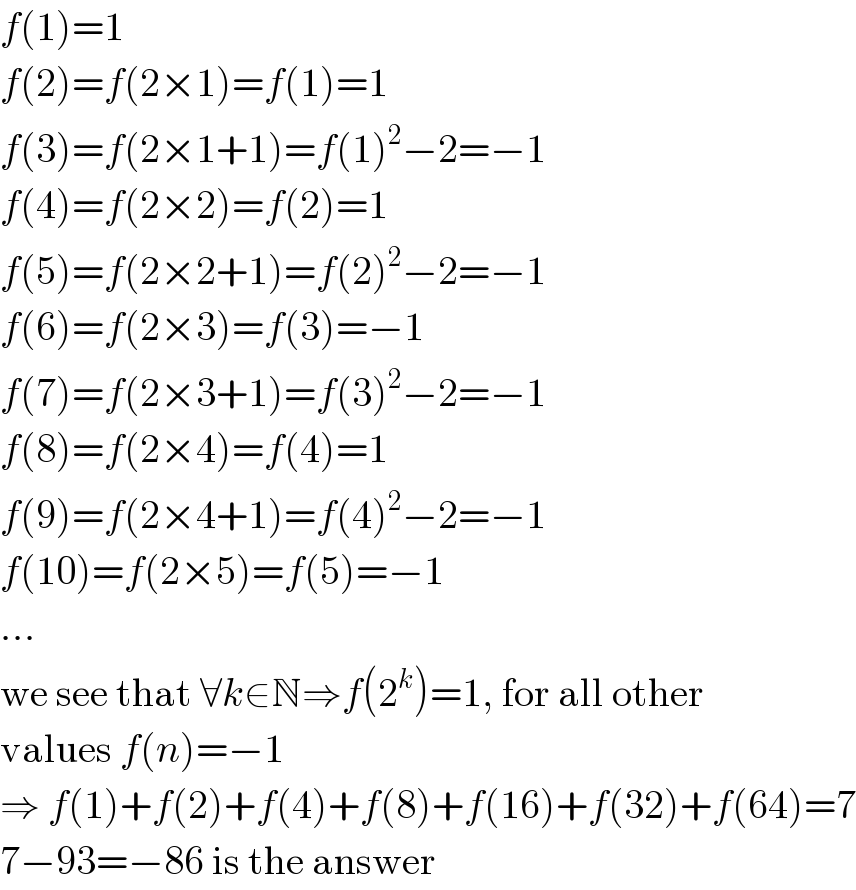
$${f}\left(\mathrm{1}\right)=\mathrm{1} \\ $$$${f}\left(\mathrm{2}\right)={f}\left(\mathrm{2}×\mathrm{1}\right)={f}\left(\mathrm{1}\right)=\mathrm{1} \\ $$$${f}\left(\mathrm{3}\right)={f}\left(\mathrm{2}×\mathrm{1}+\mathrm{1}\right)={f}\left(\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}=−\mathrm{1} \\ $$$${f}\left(\mathrm{4}\right)={f}\left(\mathrm{2}×\mathrm{2}\right)={f}\left(\mathrm{2}\right)=\mathrm{1} \\ $$$${f}\left(\mathrm{5}\right)={f}\left(\mathrm{2}×\mathrm{2}+\mathrm{1}\right)={f}\left(\mathrm{2}\right)^{\mathrm{2}} −\mathrm{2}=−\mathrm{1} \\ $$$${f}\left(\mathrm{6}\right)={f}\left(\mathrm{2}×\mathrm{3}\right)={f}\left(\mathrm{3}\right)=−\mathrm{1} \\ $$$${f}\left(\mathrm{7}\right)={f}\left(\mathrm{2}×\mathrm{3}+\mathrm{1}\right)={f}\left(\mathrm{3}\right)^{\mathrm{2}} −\mathrm{2}=−\mathrm{1} \\ $$$${f}\left(\mathrm{8}\right)={f}\left(\mathrm{2}×\mathrm{4}\right)={f}\left(\mathrm{4}\right)=\mathrm{1} \\ $$$${f}\left(\mathrm{9}\right)={f}\left(\mathrm{2}×\mathrm{4}+\mathrm{1}\right)={f}\left(\mathrm{4}\right)^{\mathrm{2}} −\mathrm{2}=−\mathrm{1} \\ $$$${f}\left(\mathrm{10}\right)={f}\left(\mathrm{2}×\mathrm{5}\right)={f}\left(\mathrm{5}\right)=−\mathrm{1} \\ $$$$... \\ $$$$\mathrm{we}\:\mathrm{see}\:\mathrm{that}\:\forall{k}\in\mathbb{N}\Rightarrow{f}\left(\mathrm{2}^{{k}} \right)=\mathrm{1},\:\mathrm{for}\:\mathrm{all}\:\mathrm{other} \\ $$$$\mathrm{values}\:{f}\left({n}\right)=−\mathrm{1} \\ $$$$\Rightarrow\:{f}\left(\mathrm{1}\right)+{f}\left(\mathrm{2}\right)+{f}\left(\mathrm{4}\right)+{f}\left(\mathrm{8}\right)+{f}\left(\mathrm{16}\right)+{f}\left(\mathrm{32}\right)+{f}\left(\mathrm{64}\right)=\mathrm{7} \\ $$$$\mathrm{7}−\mathrm{93}=−\mathrm{86}\:\mathrm{is}\:\mathrm{the}\:\mathrm{answer} \\ $$
