Question Number 47139 by Tawa1 last updated on 05/Nov/18
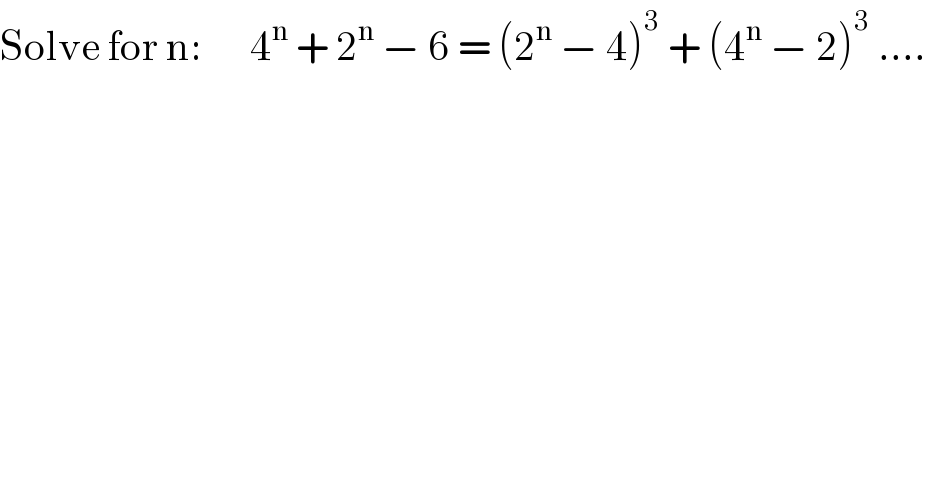
$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{n}:\:\:\:\:\:\:\mathrm{4}^{\mathrm{n}} \:+\:\mathrm{2}^{\mathrm{n}} \:−\:\mathrm{6}\:=\:\left(\mathrm{2}^{\mathrm{n}} \:−\:\mathrm{4}\right)^{\mathrm{3}} \:+\:\left(\mathrm{4}^{\mathrm{n}} \:−\:\mathrm{2}\right)^{\mathrm{3}} \:.... \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 05/Nov/18
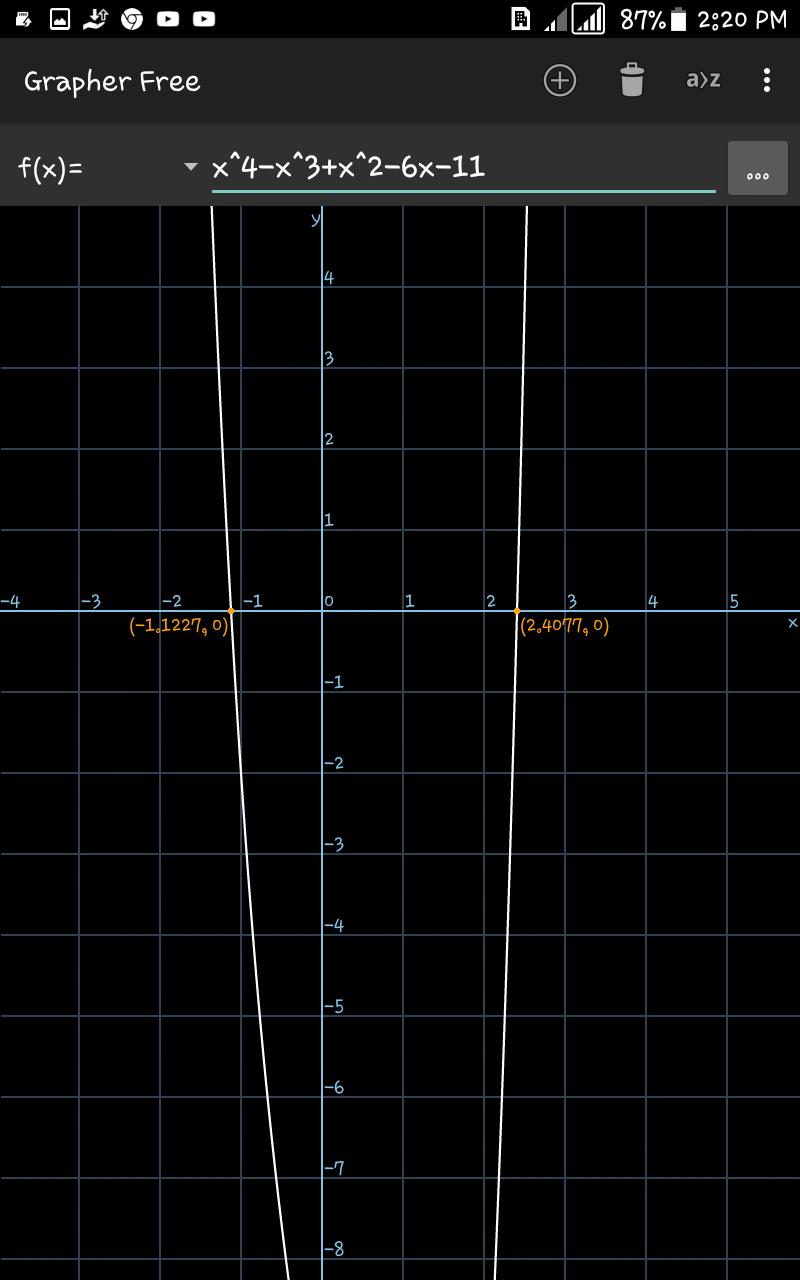
![2^n =x x^2 +x−6=(x−4)^3 +(x^2 −2)^3 (x^2 +x−6)=(x^2 +x−6){(x−4)^2 −(x−4)(x^2 −2)+(x^2 −2)^2 } (x^2 +x−6)[{x^2 −8x+16−x^3 +2x+4x^2 −8+x^4 −4x^2 +4}−1]=0 either x^2 +x−6=0 x^2 +3x−2x−6=0 x(x+3)−2(x+3)=0 (x+3)(x−2)=0 x=−3 x=2 so 2^n =2 n=1← n=1 2^n =−3 n=ln_2 (−3)←not feasible solution again... [{x^2 −8x+16−x^3 +2x+4x^2 −8+x^4 −4x^2 +4−1]=0 x^4 −x^3 +x^2 −6x−11=0 soljtion are 2.4077and −1.12227 2^n =2.4077 n=ln_2 (2.4077) 2^n =−1.2227 n=ln_2 (−1.1227) ←nog feasible soluution so n=(1 and ln_2 2.4077)](Q47141.png)
$$\mathrm{2}^{{n}} ={x} \\ $$$${x}^{\mathrm{2}} +{x}−\mathrm{6}=\left({x}−\mathrm{4}\right)^{\mathrm{3}} +\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{3}} \\ $$$$\left({x}^{\mathrm{2}} +{x}−\mathrm{6}\right)=\left({x}^{\mathrm{2}} +{x}−\mathrm{6}\right)\left\{\left({x}−\mathrm{4}\right)^{\mathrm{2}} −\left({x}−\mathrm{4}\right)\left({x}^{\mathrm{2}} −\mathrm{2}\right)+\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} \right\} \\ $$$$\left({x}^{\mathrm{2}} +{x}−\mathrm{6}\right)\left[\left\{{x}^{\mathrm{2}} −\mathrm{8}{x}+\mathrm{16}−{x}^{\mathrm{3}} +\mathrm{2}{x}+\mathrm{4}{x}^{\mathrm{2}} −\mathrm{8}+{x}^{\mathrm{4}} −\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}\right\}−\mathrm{1}\right]=\mathrm{0} \\ $$$${either} \\ $$$${x}^{\mathrm{2}} +{x}−\mathrm{6}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\mathrm{3}{x}−\mathrm{2}{x}−\mathrm{6}=\mathrm{0} \\ $$$${x}\left({x}+\mathrm{3}\right)−\mathrm{2}\left({x}+\mathrm{3}\right)=\mathrm{0} \\ $$$$\left({x}+\mathrm{3}\right)\left({x}−\mathrm{2}\right)=\mathrm{0} \\ $$$${x}=−\mathrm{3}\:\:\:\:{x}=\mathrm{2} \\ $$$${so}\:\mathrm{2}^{{n}} =\mathrm{2}\:\:\:{n}=\mathrm{1}\leftarrow\:\:{n}=\mathrm{1}\: \\ $$$$\mathrm{2}^{{n}} =−\mathrm{3} \\ $$$${n}={ln}_{\mathrm{2}} \left(−\mathrm{3}\right)\leftarrow{not}\:{feasible}\:{solution} \\ $$$${again}... \\ $$$$\left[\left\{{x}^{\mathrm{2}} −\mathrm{8}{x}+\mathrm{16}−{x}^{\mathrm{3}} +\mathrm{2}{x}+\mathrm{4}{x}^{\mathrm{2}} −\mathrm{8}+{x}^{\mathrm{4}} −\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}−\mathrm{1}\right]=\mathrm{0}\right. \\ $$$${x}^{\mathrm{4}} −{x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{6}{x}−\mathrm{11}=\mathrm{0} \\ $$$${soljtion}\:{are}\:\mathrm{2}.\mathrm{4077}{and}\:−\mathrm{1}.\mathrm{12227} \\ $$$$\mathrm{2}^{{n}} =\mathrm{2}.\mathrm{4077} \\ $$$${n}={ln}_{\mathrm{2}} \left(\mathrm{2}.\mathrm{4077}\right) \\ $$$$\mathrm{2}^{{n}} =−\mathrm{1}.\mathrm{2227} \\ $$$${n}={ln}_{\mathrm{2}} \left(−\mathrm{1}.\mathrm{1227}\right)\:\leftarrow{nog}\:{feasible}\:{soluution} \\ $$$${so}\:{n}=\left(\mathrm{1}\:{and}\:{ln}_{\mathrm{2}} \mathrm{2}.\mathrm{4077}\right) \\ $$$$ \\ $$$$ \\ $$
Commented by Tawa1 last updated on 05/Nov/18

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 05/Nov/18