
Question Number 4712 by paonky last updated on 28/Feb/16
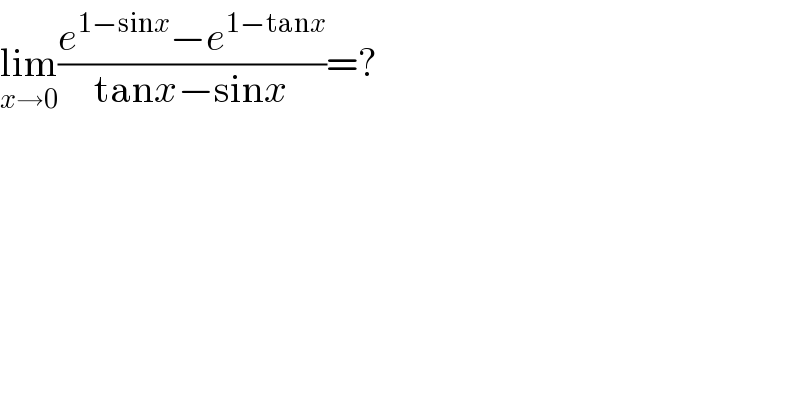
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{\mathrm{1}−\mathrm{sin}{x}} −{e}^{\mathrm{1}−\mathrm{tan}{x}} }{\mathrm{tan}{x}−\mathrm{sin}{x}}=? \\ $$
Answered by Yozzii last updated on 28/Feb/16
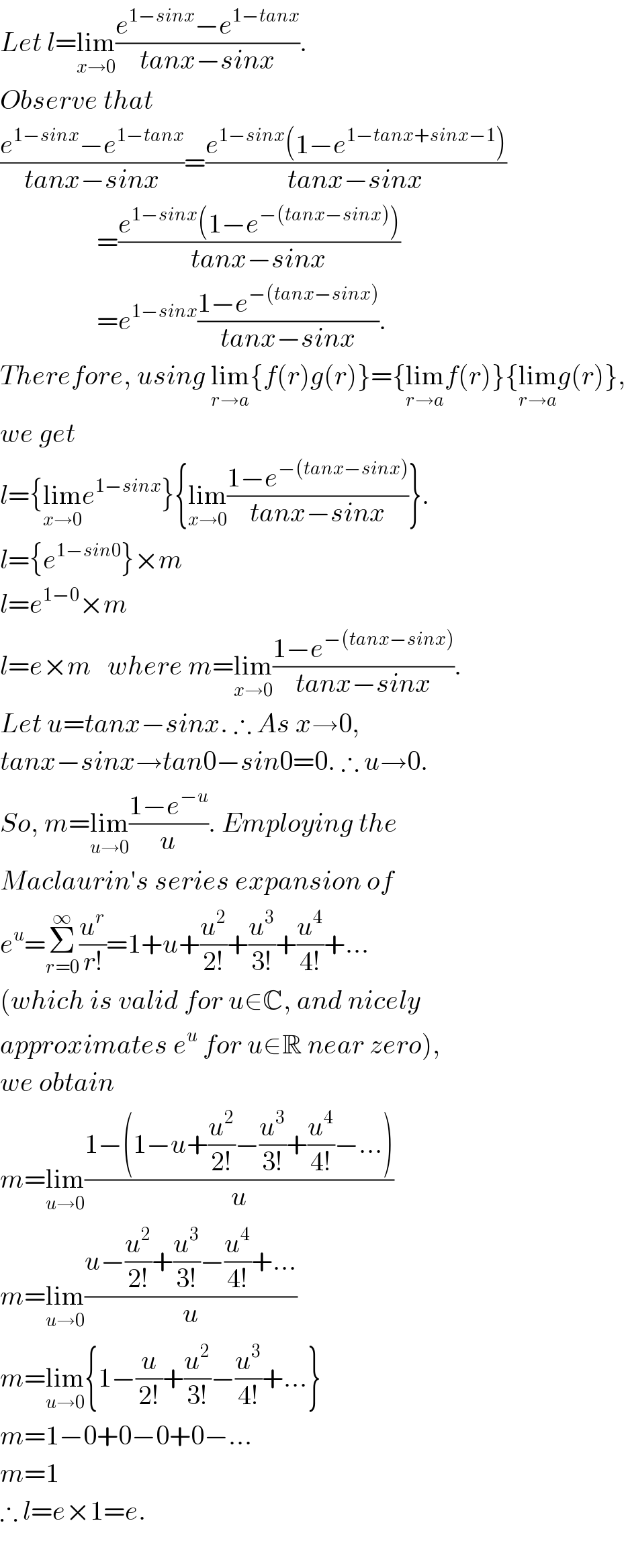
$${Let}\:{l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{\mathrm{1}−{sinx}} −{e}^{\mathrm{1}−{tanx}} }{{tanx}−{sinx}}. \\ $$$${Observe}\:{that} \\ $$$$\frac{{e}^{\mathrm{1}−{sinx}} −{e}^{\mathrm{1}−{tanx}} }{{tanx}−{sinx}}=\frac{{e}^{\mathrm{1}−{sinx}} \left(\mathrm{1}−{e}^{\mathrm{1}−{tanx}+{sinx}−\mathrm{1}} \right)}{{tanx}−{sinx}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{{e}^{\mathrm{1}−{sinx}} \left(\mathrm{1}−{e}^{−\left({tanx}−{sinx}\right)} \right)}{{tanx}−{sinx}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:={e}^{\mathrm{1}−{sinx}} \frac{\mathrm{1}−{e}^{−\left({tanx}−{sinx}\right)} }{{tanx}−{sinx}}. \\ $$$${Therefore},\:{using}\:\underset{{r}\rightarrow{a}} {\mathrm{lim}}\left\{{f}\left({r}\right){g}\left({r}\right)\right\}=\left\{\underset{{r}\rightarrow{a}} {\mathrm{lim}}{f}\left({r}\right)\right\}\left\{\underset{{r}\rightarrow{a}} {\mathrm{lim}}{g}\left({r}\right)\right\}, \\ $$$${we}\:{get}\: \\ $$$${l}=\left\{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}{e}^{\mathrm{1}−{sinx}} \right\}\left\{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−{e}^{−\left({tanx}−{sinx}\right)} }{{tanx}−{sinx}}\right\}. \\ $$$${l}=\left\{{e}^{\mathrm{1}−{sin}\mathrm{0}} \right\}×{m} \\ $$$${l}={e}^{\mathrm{1}−\mathrm{0}} ×{m} \\ $$$${l}={e}×{m}\:\:\:{where}\:{m}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−{e}^{−\left({tanx}−{sinx}\right)} }{{tanx}−{sinx}}. \\ $$$${Let}\:{u}={tanx}−{sinx}.\:\therefore\:{As}\:{x}\rightarrow\mathrm{0}, \\ $$$${tanx}−{sinx}\rightarrow{tan}\mathrm{0}−{sin}\mathrm{0}=\mathrm{0}.\:\therefore\:{u}\rightarrow\mathrm{0}. \\ $$$${So},\:{m}=\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−{e}^{−{u}} }{{u}}.\:{Employing}\:{the}\: \\ $$$${Maclaurin}'{s}\:{series}\:{expansion}\:{of}\: \\ $$$${e}^{{u}} =\underset{{r}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{{u}^{{r}} }{{r}!}=\mathrm{1}+{u}+\frac{{u}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{u}^{\mathrm{3}} }{\mathrm{3}!}+\frac{{u}^{\mathrm{4}} }{\mathrm{4}!}+... \\ $$$$\left({which}\:{is}\:{valid}\:{for}\:{u}\in\mathbb{C},\:{and}\:{nicely}\right. \\ $$$$\left.{approximates}\:{e}^{{u}} \:{for}\:{u}\in\mathbb{R}\:{near}\:{zero}\right), \\ $$$${we}\:{obtain} \\ $$$${m}=\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\left(\mathrm{1}−{u}+\frac{{u}^{\mathrm{2}} }{\mathrm{2}!}−\frac{{u}^{\mathrm{3}} }{\mathrm{3}!}+\frac{{u}^{\mathrm{4}} }{\mathrm{4}!}−...\right)}{{u}} \\ $$$${m}=\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{u}−\frac{{u}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{u}^{\mathrm{3}} }{\mathrm{3}!}−\frac{{u}^{\mathrm{4}} }{\mathrm{4}!}+...}{{u}} \\ $$$${m}=\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\mathrm{1}−\frac{{u}}{\mathrm{2}!}+\frac{{u}^{\mathrm{2}} }{\mathrm{3}!}−\frac{{u}^{\mathrm{3}} }{\mathrm{4}!}+...\right\} \\ $$$${m}=\mathrm{1}−\mathrm{0}+\mathrm{0}−\mathrm{0}+\mathrm{0}−... \\ $$$${m}=\mathrm{1} \\ $$$$\therefore\:{l}={e}×\mathrm{1}={e}.\: \\ $$$$ \\ $$
