
Question Number 47114 by maxmathsup by imad last updated on 04/Nov/18
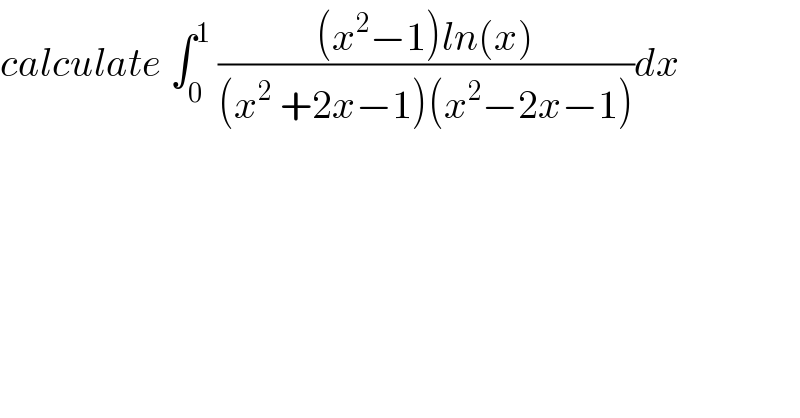
$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\left({x}^{\mathrm{2}} −\mathrm{1}\right){ln}\left({x}\right)}{\left({x}^{\mathrm{2}} \:+\mathrm{2}{x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}\right)}{dx} \\ $$
Commented by maxmathsup by imad last updated on 11/Nov/18
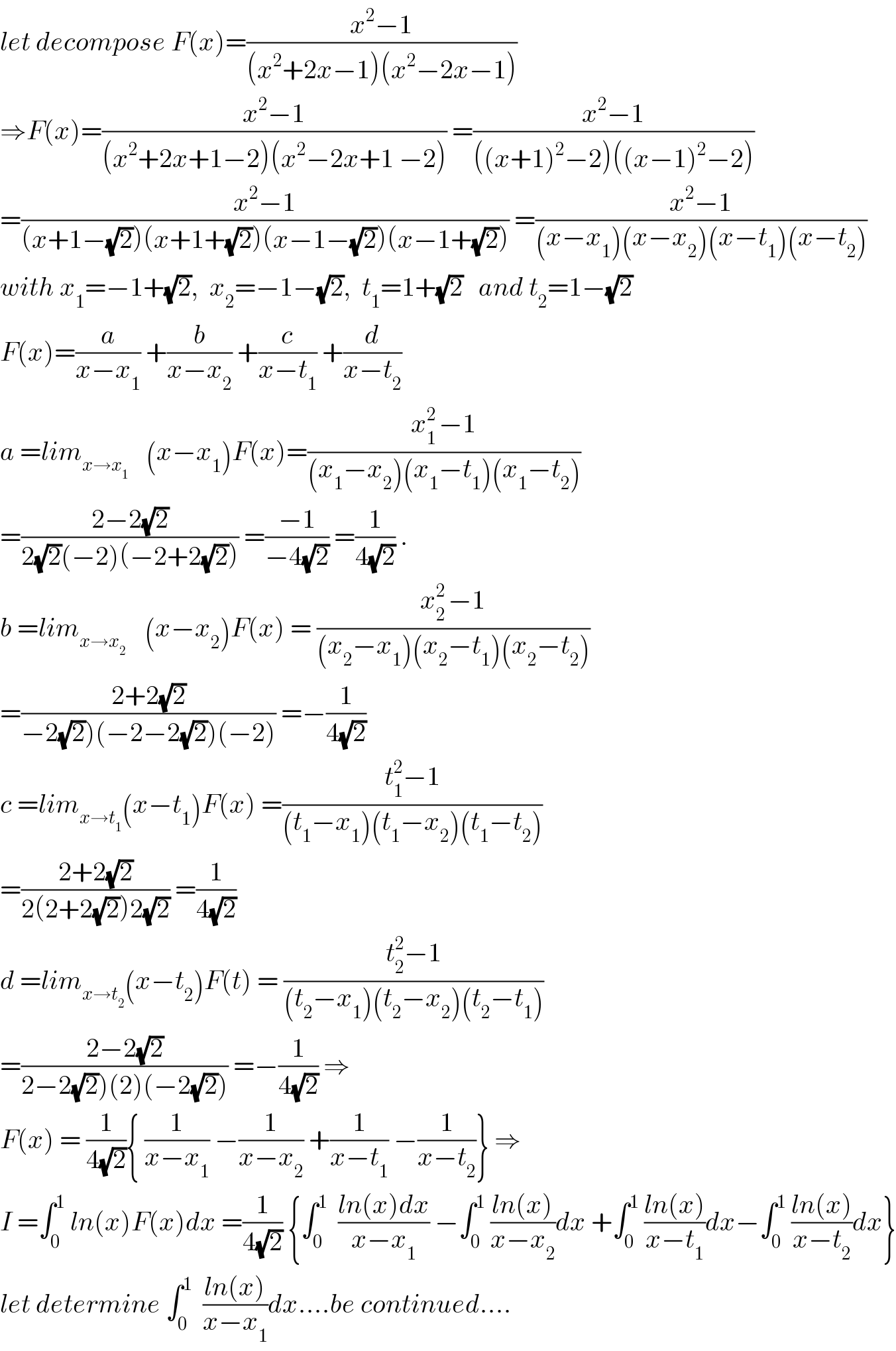
$${let}\:{decompose}\:{F}\left({x}\right)=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{1}\right)} \\ $$$$\Rightarrow{F}\left({x}\right)=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{1}−\mathrm{2}\right)\left({x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1}\:−\mathrm{2}\right)}\:=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left(\left({x}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}\right)\left(\left({x}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}\right)} \\ $$$$=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left({x}+\mathrm{1}−\sqrt{\mathrm{2}}\right)\left({x}+\mathrm{1}+\sqrt{\mathrm{2}}\right)\left({x}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\left({x}−\mathrm{1}+\sqrt{\mathrm{2}}\right)}\:=\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\left({x}−{x}_{\mathrm{1}} \right)\left({x}−{x}_{\mathrm{2}} \right)\left({x}−{t}_{\mathrm{1}} \right)\left({x}−{t}_{\mathrm{2}} \right)} \\ $$$${with}\:{x}_{\mathrm{1}} =−\mathrm{1}+\sqrt{\mathrm{2}},\:\:{x}_{\mathrm{2}} =−\mathrm{1}−\sqrt{\mathrm{2}},\:\:{t}_{\mathrm{1}} =\mathrm{1}+\sqrt{\mathrm{2}}\:\:\:{and}\:{t}_{\mathrm{2}} =\mathrm{1}−\sqrt{\mathrm{2}} \\ $$$${F}\left({x}\right)=\frac{{a}}{{x}−{x}_{\mathrm{1}} }\:+\frac{{b}}{{x}−{x}_{\mathrm{2}} }\:+\frac{{c}}{{x}−{t}_{\mathrm{1}} }\:+\frac{{d}}{{x}−{t}_{\mathrm{2}} } \\ $$$${a}\:={lim}_{{x}\rightarrow{x}_{\mathrm{1}\:} } \:\:\:\left({x}−{x}_{\mathrm{1}} \right){F}\left({x}\right)=\frac{{x}_{\mathrm{1}\:} ^{\mathrm{2}} −\mathrm{1}}{\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)\left({x}_{\mathrm{1}} −{t}_{\mathrm{1}} \right)\left({x}_{\mathrm{1}} −{t}_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}−\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}\sqrt{\mathrm{2}}\left(−\mathrm{2}\right)\left(−\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}\right)}\:=\frac{−\mathrm{1}}{−\mathrm{4}\sqrt{\mathrm{2}}}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:. \\ $$$${b}\:={lim}_{{x}\rightarrow{x}_{\mathrm{2}} \:} \:\:\:\left({x}−{x}_{\mathrm{2}} \right){F}\left({x}\right)\:=\:\frac{{x}_{\mathrm{2}\:} ^{\mathrm{2}} −\mathrm{1}}{\left({x}_{\mathrm{2}} −{x}_{\mathrm{1}} \right)\left({x}_{\mathrm{2}} −{t}_{\mathrm{1}} \right)\left({x}_{\mathrm{2}} −{t}_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}}{\left.−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(−\mathrm{2}−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(−\mathrm{2}\right)}\:=−\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}} \\ $$$${c}\:={lim}_{{x}\rightarrow{t}_{\mathrm{1}} } \left({x}−{t}_{\mathrm{1}} \right){F}\left({x}\right)\:=\frac{{t}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{1}}{\left({t}_{\mathrm{1}} −{x}_{\mathrm{1}} \right)\left({t}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)\left({t}_{\mathrm{1}} −{t}_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}\left(\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}\right)\mathrm{2}\sqrt{\mathrm{2}}}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}} \\ $$$${d}\:={lim}_{{x}\rightarrow{t}_{\mathrm{2}} } \left({x}−{t}_{\mathrm{2}} \right){F}\left({t}\right)\:=\:\frac{{t}_{\mathrm{2}} ^{\mathrm{2}} −\mathrm{1}}{\left({t}_{\mathrm{2}} −{x}_{\mathrm{1}} \right)\left({t}_{\mathrm{2}} −{x}_{\mathrm{2}} \right)\left({t}_{\mathrm{2}} −{t}_{\mathrm{1}} \right)} \\ $$$$=\frac{\mathrm{2}−\mathrm{2}\sqrt{\mathrm{2}}}{\left.\mathrm{2}−\mathrm{2}\sqrt{\mathrm{2}}\right)\left(\mathrm{2}\right)\left(−\mathrm{2}\sqrt{\mathrm{2}}\right)}\:=−\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\Rightarrow \\ $$$${F}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\left\{\:\frac{\mathrm{1}}{{x}−{x}_{\mathrm{1}} }\:−\frac{\mathrm{1}}{{x}−{x}_{\mathrm{2}} }\:+\frac{\mathrm{1}}{{x}−{t}_{\mathrm{1}} }\:−\frac{\mathrm{1}}{{x}−{t}_{\mathrm{2}} }\right\}\:\Rightarrow \\ $$$${I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:{ln}\left({x}\right){F}\left({x}\right){dx}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\left\{\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left({x}\right){dx}}{{x}−{x}_{\mathrm{1}} }\:−\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left({x}\right)}{{x}−{x}_{\mathrm{2}} }{dx}\:+\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left({x}\right)}{{x}−{t}_{\mathrm{1}} }{dx}−\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left({x}\right)}{{x}−{t}_{\mathrm{2}} }{dx}\right\} \\ $$$${let}\:{determine}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left({x}\right)}{{x}−{x}_{\mathrm{1}} }{dx}....{be}\:{continued}.... \\ $$
