
Question Number 46838 by peter frank last updated on 01/Nov/18
Answered by MrW3 last updated on 03/Nov/18
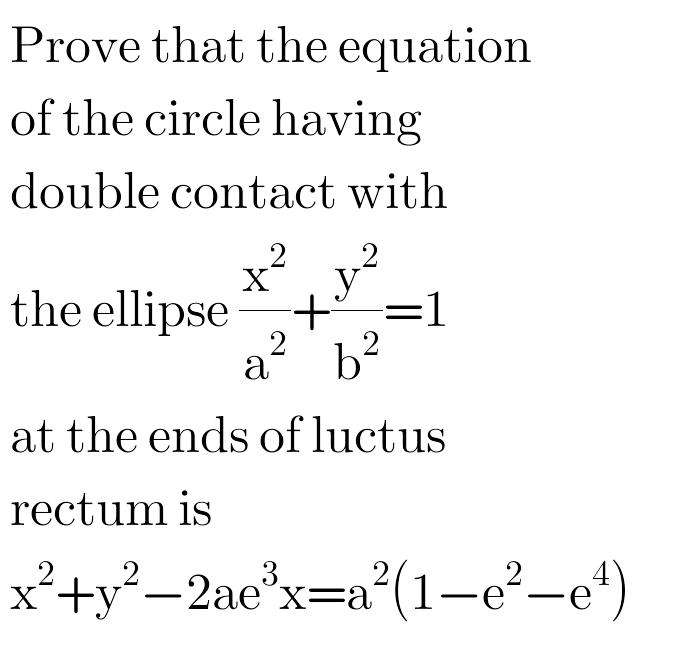
![(x^2 /a^2 )+(y^2 /b^2 )=1 ((2x)/a^2 )+((2y)/b^2 )y′=0⇒(x/a^2 )+(y/b^2 )y′=0 e^2 =((a^2 −b^2 )/a^2 ) eqn. of circle: (x−c)^2 +y^2 =r^2 (say) 2(x−c)+2yy′=0⇒(x−c)+yy′=0 contact points: (ea,k) and (ea,−k) ((e^2 a^2 )/a^2 )+(k^2 /b^2 )=1 e^2 +(k^2 /b^2 )=1 ⇒e^2 b^2 +k^2 =b^2 ...(i) (ea−c)^2 +k^2 =r^2 ...(ii) ((ea)/a^2 )+(k/b^2 )m=0 ((eb^2 )/a)+km=0 ...(iii) ea−c+km=0 ...(iv) (i)−(ii): e^2 b^2 −(ea−c)^2 =b^2 −r^2 ...(v) (iii)−(iv): ((eb^2 )/a)−ea+c=0 ⇒c=e(a−(b^2 /a))=((e(a^2 −b^2 ))/a)=e^3 a from (v): r^2 =b^2 (1−e^2 )+(ea−c)^2 =b^2 (1−e^2 )+((e^2 b^4 )/a^2 )=b^2 [1−((e^2 (a^2 −b^2 )/a^2 )]=b^2 (1−e^4 )=a^2 (1−e^2 )(1−e^4 )=a^2 (1−e^2 −e^4 +e^6 ) ⇒eqn. of circle: (x−e^3 a)^2 +y^2 =b^2 (1−e^4 ) y^2 +x^2 −2ae^3 x+a^2 e^6 =a^2 (1−e^2 −e^4 +e^6 ) ⇒y^2 +x^2 −2ae^3 x=a^2 (1−e^2 −e^4 )](Q46957.png)
$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\frac{\mathrm{2}{x}}{{a}^{\mathrm{2}} }+\frac{\mathrm{2}{y}}{{b}^{\mathrm{2}} }{y}'=\mathrm{0}\Rightarrow\frac{{x}}{{a}^{\mathrm{2}} }+\frac{{y}}{{b}^{\mathrm{2}} }{y}'=\mathrm{0} \\ $$$${e}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} } \\ $$$$ \\ $$$${eqn}.\:{of}\:{circle}: \\ $$$$\left({x}−{c}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} ={r}^{\mathrm{2}} \:\:\left({say}\right) \\ $$$$\mathrm{2}\left({x}−{c}\right)+\mathrm{2}{yy}'=\mathrm{0}\Rightarrow\left({x}−{c}\right)+{yy}'=\mathrm{0} \\ $$$$ \\ $$$${contact}\:{points}:\:\left({ea},{k}\right)\:{and}\:\left({ea},−{k}\right) \\ $$$$\frac{{e}^{\mathrm{2}} {a}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$${e}^{\mathrm{2}} +\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\Rightarrow{e}^{\mathrm{2}} {b}^{\mathrm{2}} +{k}^{\mathrm{2}} ={b}^{\mathrm{2}} \:\:\:...\left({i}\right) \\ $$$$\left({ea}−{c}\right)^{\mathrm{2}} +{k}^{\mathrm{2}} ={r}^{\mathrm{2}} \:\:\:...\left({ii}\right) \\ $$$$\frac{{ea}}{{a}^{\mathrm{2}} }+\frac{{k}}{{b}^{\mathrm{2}} }{m}=\mathrm{0} \\ $$$$\frac{{eb}^{\mathrm{2}} }{{a}}+{km}=\mathrm{0}\:\:\:...\left({iii}\right) \\ $$$${ea}−{c}+{km}=\mathrm{0}\:\:\:...\left({iv}\right) \\ $$$$ \\ $$$$\left({i}\right)−\left({ii}\right): \\ $$$${e}^{\mathrm{2}} {b}^{\mathrm{2}} −\left({ea}−{c}\right)^{\mathrm{2}} ={b}^{\mathrm{2}} −{r}^{\mathrm{2}} \:\:\:...\left({v}\right) \\ $$$$\left({iii}\right)−\left({iv}\right): \\ $$$$\frac{{eb}^{\mathrm{2}} }{{a}}−{ea}+{c}=\mathrm{0} \\ $$$$\Rightarrow{c}={e}\left({a}−\frac{{b}^{\mathrm{2}} }{{a}}\right)=\frac{{e}\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}{{a}}={e}^{\mathrm{3}} {a} \\ $$$${from}\:\left({v}\right): \\ $$$${r}^{\mathrm{2}} ={b}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} \right)+\left({ea}−{c}\right)^{\mathrm{2}} ={b}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} \right)+\frac{{e}^{\mathrm{2}} {b}^{\mathrm{4}} }{{a}^{\mathrm{2}} }={b}^{\mathrm{2}} \left[\mathrm{1}−\frac{{e}^{\mathrm{2}} \left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right.}{{a}^{\mathrm{2}} }\right]={b}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{4}} \right)={a}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} \right)\left(\mathrm{1}−{e}^{\mathrm{4}} \right)={a}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} −{e}^{\mathrm{4}} +{e}^{\mathrm{6}} \right) \\ $$$$\Rightarrow{eqn}.\:{of}\:{circle}: \\ $$$$\left({x}−{e}^{\mathrm{3}} {a}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} ={b}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{4}} \right) \\ $$$${y}^{\mathrm{2}} +{x}^{\mathrm{2}} −\mathrm{2}{ae}^{\mathrm{3}} {x}+{a}^{\mathrm{2}} {e}^{\mathrm{6}} ={a}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} −{e}^{\mathrm{4}} +{e}^{\mathrm{6}} \right) \\ $$$$\Rightarrow{y}^{\mathrm{2}} +{x}^{\mathrm{2}} −\mathrm{2}{ae}^{\mathrm{3}} {x}={a}^{\mathrm{2}} \left(\mathrm{1}−{e}^{\mathrm{2}} −{e}^{\mathrm{4}} \right) \\ $$
Commented by peter frank last updated on 04/Nov/18
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{mrW}_{\mathrm{3}} \\ $$
