
Question Number 44436 by ajfour last updated on 29/Sep/18
Commented by ajfour last updated on 29/Sep/18
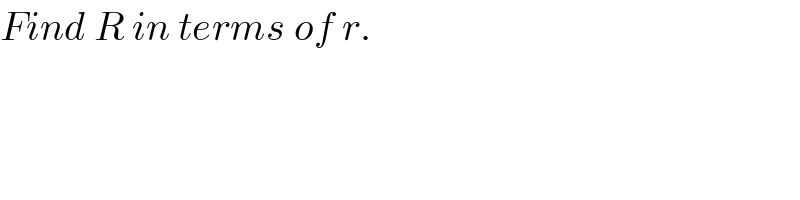
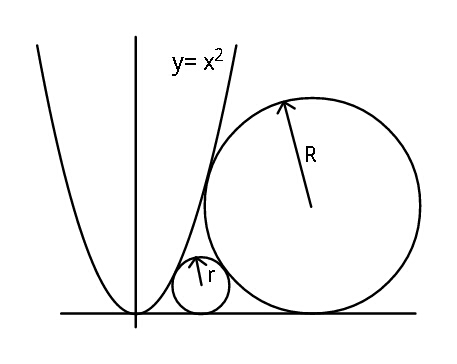
$${Find}\:{R}\:{in}\:{terms}\:{of}\:{r}. \\ $$
Answered by MrW3 last updated on 29/Sep/18
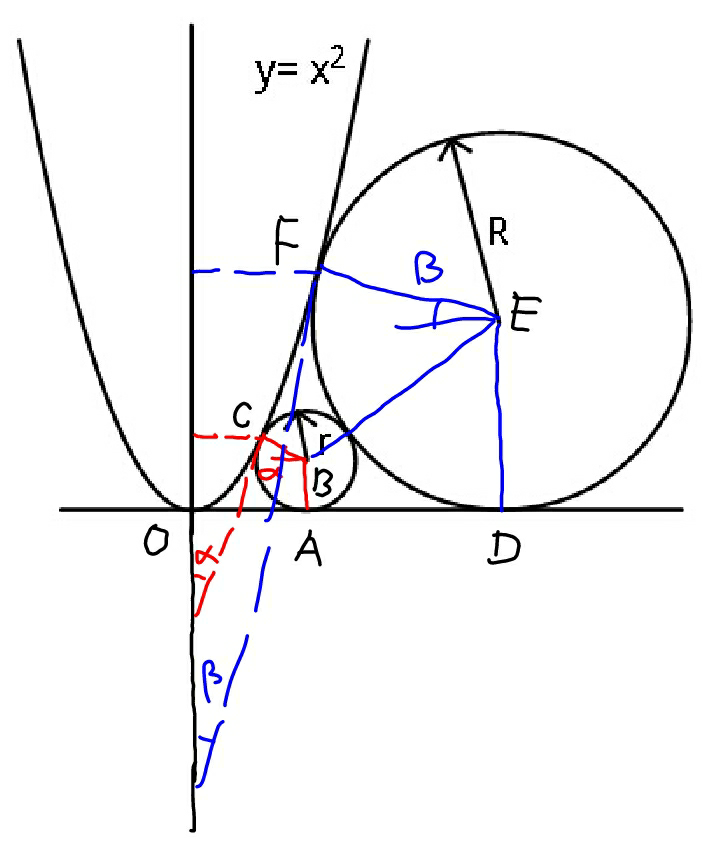
Commented by MrW3 last updated on 29/Sep/18

$${assume}\:{eqn}.\:{of}\:{parabola}\:{is}\:{y}=\frac{{x}^{\mathrm{2}} }{{a}} \\ $$$${y}_{{C}} ={x}_{{C}} ^{\mathrm{2}} /{a}={r}+{r}\:\mathrm{sin}\:\alpha={r}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right) \\ $$$$\mathrm{tan}\:\alpha=\frac{{x}_{{C}} }{\mathrm{2}{y}_{{C}} }=\frac{{ax}_{{C}} ^{\mathrm{2}} }{\mathrm{2}{ay}_{{C}} ×{x}_{{C}} }=\frac{{a}}{\mathrm{2}{x}_{{C}} }=\frac{\sqrt{{a}}}{\mathrm{2}\sqrt{{r}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)}} \\ $$$$\mathrm{4}\:\mathrm{tan}^{\mathrm{2}} \:\alpha=\frac{{a}}{{r}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)} \\ $$$$\mathrm{4}{r}\:\mathrm{sin}^{\mathrm{2}} \:\alpha\:\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)={a}\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\alpha\right) \\ $$$$\mathrm{4}\:\mathrm{sin}^{\mathrm{3}} \:\alpha\:+\left(\mathrm{4}+\frac{{a}}{{r}}\right)\mathrm{sin}^{\mathrm{2}} \:\alpha−\frac{{a}}{{r}}=\mathrm{0} \\ $$$${or}\:{with}\:\mu=\frac{{a}}{{r}} \\ $$$$\mathrm{4}\:\mathrm{sin}^{\mathrm{3}} \:\alpha\:+\left(\mathrm{4}+\mu\right)\mathrm{sin}^{\mathrm{2}} \:\alpha−\mu=\mathrm{0}\:\:\:\:...\left({i}\right) \\ $$$$ \\ $$$$\Rightarrow\alpha=...\:{in}\:{term}\:{of}\:{r} \\ $$$${x}_{{B}} ={x}_{{C}} +{r}\:\mathrm{cos}\:\alpha=\sqrt{{ar}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)}+{r}\:\mathrm{cos}\:\alpha \\ $$$$ \\ $$$${similarly}: \\ $$$${x}_{{E}} =\sqrt{{aR}\left(\mathrm{1}+\mathrm{sin}\:\beta\right)}+{R}\:\mathrm{cos}\:\beta \\ $$$$\mathrm{4}\:\mathrm{sin}^{\mathrm{3}} \:\beta\:+\left(\mathrm{4}+\frac{{a}}{{R}}\right)\mathrm{sin}^{\mathrm{2}} \:\beta−\frac{{a}}{{R}}=\mathrm{0} \\ $$$${or}\:{with}\:\lambda=\frac{{a}}{{R}} \\ $$$$\mathrm{4}\:\mathrm{sin}^{\mathrm{3}} \:\beta\:+\left(\mathrm{4}+\lambda\right)\mathrm{sin}^{\mathrm{2}} \:\beta−\lambda=\mathrm{0}\:\:\:...\left({ii}\right) \\ $$$$ \\ $$$$\left({R}−{r}\right)^{\mathrm{2}} +\left({x}_{{E}} −{x}_{{B}} \right)^{\mathrm{2}} =\left({R}+{r}\right)^{\mathrm{2}} \\ $$$${x}_{{E}} −{x}_{{B}} =\mathrm{2}\sqrt{{Rr}} \\ $$$$\sqrt{{aR}\left(\mathrm{1}+\mathrm{sin}\:\beta\right)}+{R}\:\mathrm{cos}\:\beta−\sqrt{{ar}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)}−{r}\:\mathrm{cos}\:\alpha=\mathrm{2}\sqrt{{Rr}} \\ $$$$\sqrt{{aR}\left(\mathrm{1}+\mathrm{sin}\:\beta\right)}+{R}\:\mathrm{cos}\:\beta−\mathrm{2}\sqrt{{Rr}}=\sqrt{{ar}\left(\mathrm{1}+\mathrm{sin}\:\alpha\right)}+{r}\:\mathrm{cos}\:\alpha \\ $$$$\sqrt{\frac{\mathrm{1}+\mathrm{sin}\:\beta}{\lambda}}+\frac{\mathrm{cos}\:\beta}{\lambda}−\frac{\mathrm{2}}{\sqrt{\mu\lambda}}=\sqrt{\frac{\mathrm{1}+\mathrm{sin}\:\alpha}{\mu}}+\frac{\mathrm{cos}\:\alpha}{\mu}\:\:\:...\left({iii}\right) \\ $$$$ \\ $$$${three}\:{eqn}.\:{with}\:{three}\:{unknowns}:\:\alpha,\beta,\lambda \\ $$$$..... \\ $$$${for}\:{r}=\mathrm{2},\:{I}\:{found}\:{R}\approx\mathrm{8}.\mathrm{842} \\ $$
Commented by ajfour last updated on 29/Sep/18
$${great}\:{way}\:{sir},\:{very}\:{nice},\:{thanks}. \\ $$
Commented by ajfour last updated on 29/Sep/18
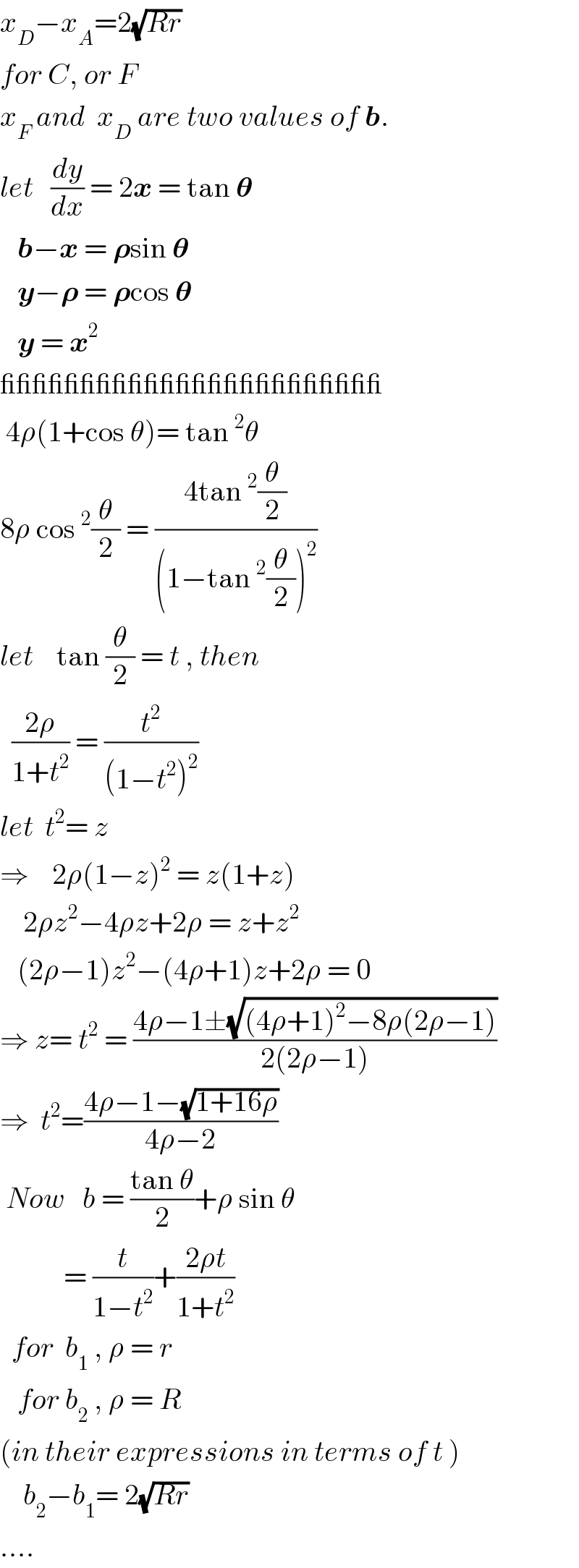
$${x}_{{D}} −{x}_{{A}} =\mathrm{2}\sqrt{{Rr}} \\ $$$${for}\:{C},\:{or}\:{F} \\ $$$${x}_{{F}} \:{and}\:\:{x}_{{D}} \:{are}\:{two}\:{values}\:{of}\:\boldsymbol{{b}}. \\ $$$${let}\:\:\:\frac{{dy}}{{dx}}\:=\:\mathrm{2}\boldsymbol{{x}}\:=\:\mathrm{tan}\:\boldsymbol{\theta} \\ $$$$\:\:\:\boldsymbol{{b}}−\boldsymbol{{x}}\:=\:\boldsymbol{\rho}\mathrm{sin}\:\boldsymbol{\theta} \\ $$$$\:\:\:\boldsymbol{{y}}−\boldsymbol{\rho}\:=\:\boldsymbol{\rho}\mathrm{cos}\:\boldsymbol{\theta} \\ $$$$\:\:\:\boldsymbol{{y}}\:=\:\boldsymbol{{x}}^{\mathrm{2}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\:\mathrm{4}\rho\left(\mathrm{1}+\mathrm{cos}\:\theta\right)=\:\mathrm{tan}\:^{\mathrm{2}} \theta \\ $$$$\mathrm{8}\rho\:\mathrm{cos}\:^{\mathrm{2}} \frac{\theta}{\mathrm{2}}\:=\:\frac{\mathrm{4tan}\:^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}{\left(\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \frac{\theta}{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$${let}\:\:\:\:\mathrm{tan}\:\frac{\theta}{\mathrm{2}}\:=\:{t}\:,\:{then} \\ $$$$\:\:\frac{\mathrm{2}\rho}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\:\frac{{t}^{\mathrm{2}} }{\left(\mathrm{1}−{t}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$${let}\:\:{t}^{\mathrm{2}} =\:{z} \\ $$$$\Rightarrow\:\:\:\:\mathrm{2}\rho\left(\mathrm{1}−{z}\right)^{\mathrm{2}} \:=\:{z}\left(\mathrm{1}+{z}\right) \\ $$$$\:\:\:\:\mathrm{2}\rho{z}^{\mathrm{2}} −\mathrm{4}\rho{z}+\mathrm{2}\rho\:=\:{z}+{z}^{\mathrm{2}} \\ $$$$\:\:\:\left(\mathrm{2}\rho−\mathrm{1}\right){z}^{\mathrm{2}} −\left(\mathrm{4}\rho+\mathrm{1}\right){z}+\mathrm{2}\rho\:=\:\mathrm{0} \\ $$$$\Rightarrow\:{z}=\:{t}^{\mathrm{2}} \:=\:\frac{\mathrm{4}\rho−\mathrm{1}\pm\sqrt{\left(\mathrm{4}\rho+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{8}\rho\left(\mathrm{2}\rho−\mathrm{1}\right)}}{\mathrm{2}\left(\mathrm{2}\rho−\mathrm{1}\right)} \\ $$$$\Rightarrow\:\:{t}^{\mathrm{2}} =\frac{\mathrm{4}\rho−\mathrm{1}−\sqrt{\mathrm{1}+\mathrm{16}\rho}}{\mathrm{4}\rho−\mathrm{2}} \\ $$$$\:{Now}\:\:\:{b}\:=\:\frac{\mathrm{tan}\:\theta}{\mathrm{2}}+\rho\:\mathrm{sin}\:\theta \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\:\frac{{t}}{\mathrm{1}−{t}^{\mathrm{2}} }+\frac{\mathrm{2}\rho{t}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$\:\:{for}\:\:{b}_{\mathrm{1}} \:,\:\rho\:=\:{r} \\ $$$$\:\:\:{for}\:{b}_{\mathrm{2}} \:,\:\rho\:=\:{R} \\ $$$$\left({in}\:{their}\:{expressions}\:{in}\:{terms}\:{of}\:{t}\:\right) \\ $$$$\:\:\:\:{b}_{\mathrm{2}} −{b}_{\mathrm{1}} =\:\mathrm{2}\sqrt{{Rr}} \\ $$$$.... \\ $$
Commented by MrW3 last updated on 29/Sep/18
$${Finding}\:{a}\:{direct}\:{expression}\:{for}\:\theta\:{in} \\ $$$${term}\:{of}\:{R}\:\left({and}\:{r}\right),\:{that}'{s}\:{great}\:{sir}! \\ $$
Commented by ajfour last updated on 29/Sep/18
$${i}\:{have}\:{changed}\:{it}\:{entirely}\:{sir}, \\ $$$${its}\:{all}\:{just}\:{the}\:{same}\:\left({parametric}\right). \\ $$$${probably},\:{implicit}\:{as}\:{well}. \\ $$
Commented by behi83417@gmail.com last updated on 29/Sep/18

$${nice}\:{work}\:{done}!{thanks}\:{master} \\ $$$${sir}\:{mrW}\mathrm{3}. \\ $$
Commented by behi83417@gmail.com last updated on 29/Sep/18
$${sir}\:{Ajfour}!\:{great}\:{question}\:\&\:{also} \\ $$$${nice}\:{prof}.{thanks}\:{for}\:{your}\:{nice} \\ $$$${viewpoint}. \\ $$
