
Question Number 42375 by Joel578 last updated on 24/Aug/18
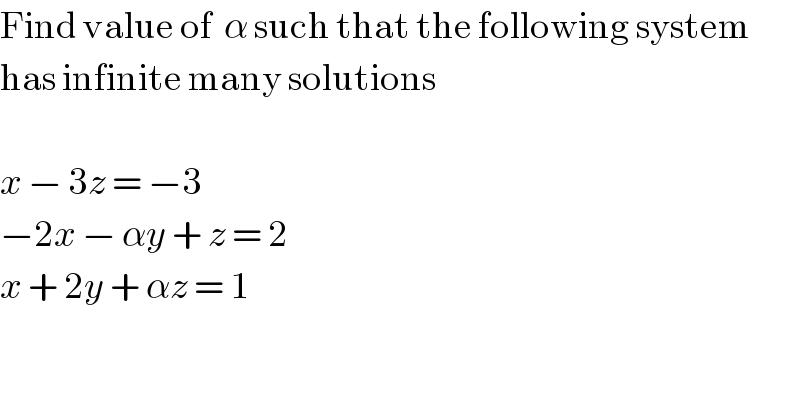
$$\mathrm{Find}\:\mathrm{value}\:\mathrm{of}\:\:\alpha\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{following}\:\mathrm{system} \\ $$$$\mathrm{has}\:\mathrm{infinite}\:\mathrm{many}\:\mathrm{solutions} \\ $$$$ \\ $$$${x}\:−\:\mathrm{3}{z}\:=\:−\mathrm{3} \\ $$$$−\mathrm{2}{x}\:−\:\alpha{y}\:+\:{z}\:=\:\mathrm{2} \\ $$$${x}\:+\:\mathrm{2}{y}\:+\:\alpha{z}\:=\:\mathrm{1} \\ $$
Commented by Joel578 last updated on 24/Aug/18
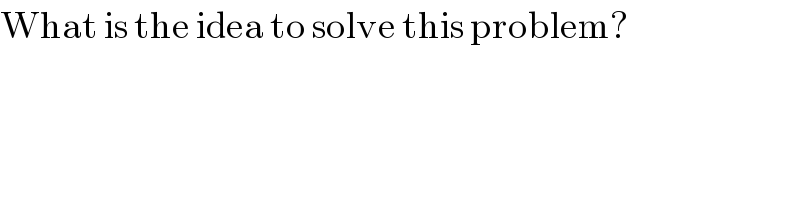
$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{idea}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{problem}? \\ $$
Commented by maxmathsup by imad last updated on 24/Aug/18

$${first}\:{we}\:{must}\:{have}\:{det}\:{A}\:=\mathrm{0}\:\:\:\:\:\left({if}\:{detA}\neq\mathrm{0}\:{weget}\:{a}\:{unique}\:{solution}\right) \\ $$$${with}\:{A}\:=\begin{pmatrix}{\mathrm{1}\:\:\:\:\:\:\mathrm{0}\:\:\:\:\:\:\:−\mathrm{3}}\\{−\mathrm{2}\:\:\:−\alpha\:\:\:\:\:\mathrm{1}}\end{pmatrix} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{1}\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\:\:\:\alpha\right) \\ $$$${det}\:{A}\:\:=−\alpha^{\mathrm{2}} −\mathrm{2}\:+\mathrm{2}\left(\mathrm{6}\right)\:−\mathrm{3}\alpha\:=−\alpha^{\mathrm{2}} \:−\mathrm{3}\alpha\:+\mathrm{10}\: \\ $$$${det}\:{A}\:=\mathrm{0}\:\Rightarrow\alpha^{\mathrm{2}} \:+\mathrm{3}\alpha\:−\mathrm{10}\:=\mathrm{0} \\ $$$$\Delta\:=\mathrm{9}\:+\mathrm{40}\:=\mathrm{49}\:\Rightarrow\alpha_{\mathrm{1}} =\:\:\frac{−\mathrm{3}\:+\mathrm{7}}{\mathrm{2}}\:=\mathrm{2}\:\:\:\:{and}\:\:\alpha_{\mathrm{2}} =\:\frac{−\mathrm{3}−\mathrm{7}}{\mathrm{2}}\:=−\mathrm{5} \\ $$$${for}\:\alpha\:=\mathrm{2}\:\:{we}\:{get}\:{the}\:{system}\:\:\:\begin{cases}{{x}−\mathrm{3}{z}\:=−\mathrm{3}}\\{−\mathrm{2}{x}−\mathrm{2}{y}+{z}\:\:=\mathrm{2}}\end{cases}\:\:\:\:\Rightarrow \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{{x}+\mathrm{2}{y}\:+\mathrm{2}{z}\:=\mathrm{1}\right. \\ $$$$\begin{cases}{{x}−\mathrm{3}{z}\:=−\mathrm{3}}\\{−{x}+\mathrm{3}{z}\:=\mathrm{3}\:\:\:\:\:\:\:\:{and}\:\:\:\:{x}+\mathrm{2}{y}\:+\mathrm{2}{z}\:=\mathrm{1}\:\Rightarrow\begin{cases}{{x}−\mathrm{3}{z}\:=−\mathrm{3}}\\{\mathrm{2}{y}\:=\mathrm{1}−{x}−\mathrm{2}{z}}\end{cases}}\end{cases} \\ $$$$\Rightarrow\:\begin{cases}{{x}\:=\mathrm{3}{z}−\mathrm{3}}\\{{y}=\frac{\mathrm{1}}{\mathrm{2}}\left\{\mathrm{1}−\mathrm{3}{z}\:+\mathrm{3}−\mathrm{2}{z}\right\}=\frac{\mathrm{1}}{\mathrm{2}}\left\{\mathrm{2}−\mathrm{5}{z}\right\}\:\:\:\Rightarrow}\end{cases} \\ $$$$\left({x},{y},{z}\right)\:=\left(\mathrm{3}{z}−\mathrm{3},\mathrm{1}−\frac{\mathrm{5}}{\mathrm{2}}{z}\:,{z}\right)\:\:\:\:{infinite}\:{solution} \\ $$$${for}\:\alpha\:=−\mathrm{5}\:\:\:{we}\:{follow}\:{the}\:{same}\:{method}\:.... \\ $$
Commented by Joel578 last updated on 25/Aug/18

$${thank}\:{you}\:{very}\:{much} \\ $$
Commented by math khazana by abdo last updated on 26/Aug/18
$${you}\:{are}\:{welcome}. \\ $$
Commented by Joel578 last updated on 27/Aug/18

$$\mathrm{Sir},\:\mathrm{if}\:\alpha\:=\:−\mathrm{5},\:\mathrm{the}\:\mathrm{system}\:\mathrm{will}\:\mathrm{have}\:\mathrm{no}\:\mathrm{solution} \\ $$
Answered by behi83417@gmail.com last updated on 24/Aug/18
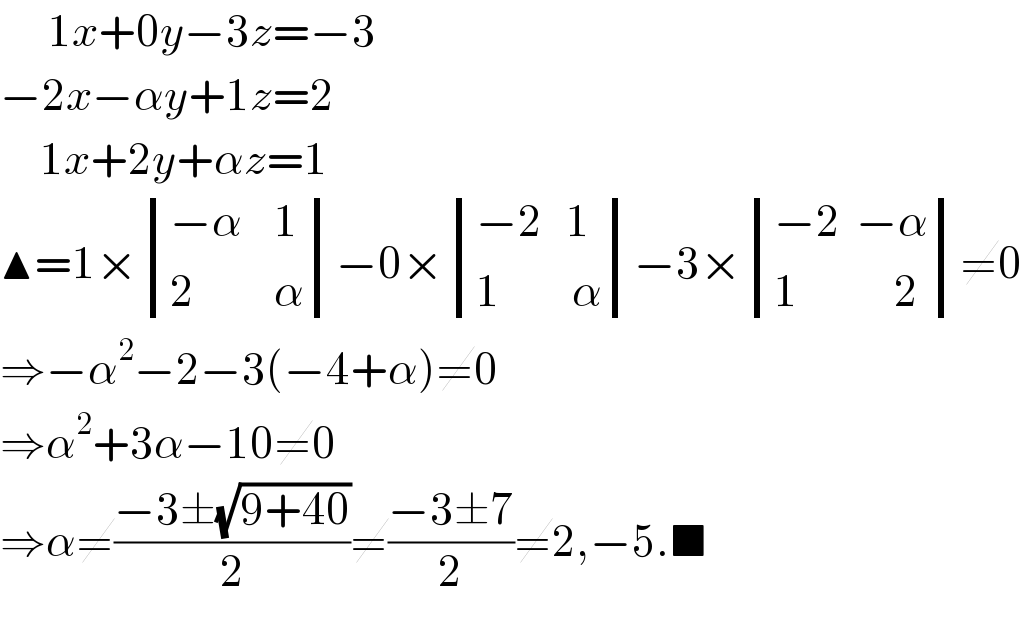
$$\:\:\:\:\:\:\mathrm{1}{x}+\mathrm{0}{y}−\mathrm{3}{z}=−\mathrm{3} \\ $$$$−\mathrm{2}{x}−\alpha{y}+\mathrm{1}{z}=\mathrm{2} \\ $$$$\:\:\:\:\:\mathrm{1}{x}+\mathrm{2}{y}+\alpha{z}=\mathrm{1} \\ $$$$\blacktriangle=\mathrm{1}×\begin{vmatrix}{−\alpha\:\:\:\:\mathrm{1}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:\alpha}\end{vmatrix}−\mathrm{0}×\begin{vmatrix}{−\mathrm{2}\:\:\:\mathrm{1}}\\{\mathrm{1}\:\:\:\:\:\:\:\:\:\alpha}\end{vmatrix}−\mathrm{3}×\begin{vmatrix}{−\mathrm{2}\:\:−\alpha}\\{\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}}\end{vmatrix}\neq\mathrm{0} \\ $$$$\Rightarrow−\alpha^{\mathrm{2}} −\mathrm{2}−\mathrm{3}\left(−\mathrm{4}+\alpha\right)\neq\mathrm{0} \\ $$$$\Rightarrow\alpha^{\mathrm{2}} +\mathrm{3}\alpha−\mathrm{10}\neq\mathrm{0} \\ $$$$\Rightarrow\alpha\neq\frac{−\mathrm{3}\pm\sqrt{\mathrm{9}+\mathrm{40}}}{\mathrm{2}}\neq\frac{−\mathrm{3}\pm\mathrm{7}}{\mathrm{2}}\neq\mathrm{2},−\mathrm{5}.\blacksquare \\ $$
Commented by maxmathsup by imad last updated on 24/Aug/18

$${sir}\:{behi}\:\:{if}\:{det}\:{M}\:\neq\:\mathrm{0}\:\:{the}\:{system}\:{have}\:{one}\:{solution}\:\:... \\ $$
Commented by behi83417@gmail.com last updated on 24/Aug/18
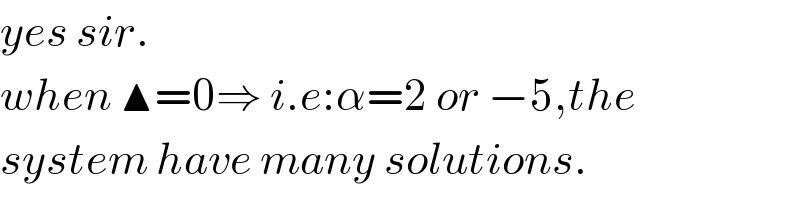
$${yes}\:{sir}. \\ $$$${when}\:\blacktriangle=\mathrm{0}\Rightarrow\:{i}.{e}:\alpha=\mathrm{2}\:{or}\:−\mathrm{5},{the} \\ $$$${system}\:{have}\:{many}\:{solutions}. \\ $$
Commented by Joel578 last updated on 25/Aug/18
$${thank}\:{you}\:{very}\:{much} \\ $$
Commented by Joel578 last updated on 27/Aug/18
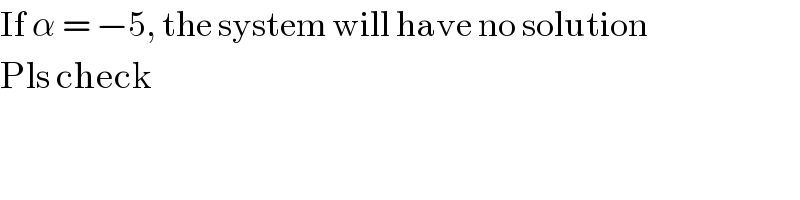
$$\mathrm{If}\:\alpha\:=\:−\mathrm{5},\:\mathrm{the}\:\mathrm{system}\:\mathrm{will}\:\mathrm{have}\:\mathrm{no}\:\mathrm{solution} \\ $$$$\mathrm{Pls}\:\mathrm{check} \\ $$
Commented by Joel578 last updated on 27/Aug/18
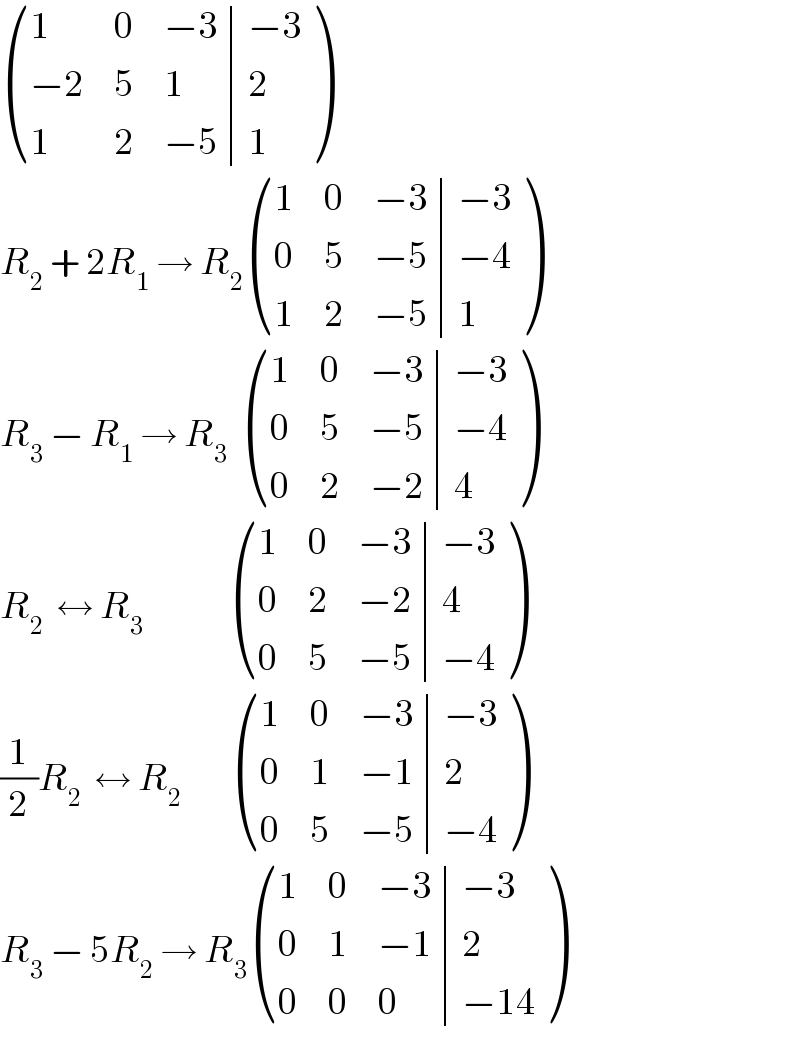
$$\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{−\mathrm{2}}&{\mathrm{5}}&{\mathrm{1}}&{\mathrm{2}}\\{\mathrm{1}}&{\mathrm{2}}&{−\mathrm{5}}&{\mathrm{1}}\end{pmatrix} \\ $$$${R}_{\mathrm{2}} \:+\:\mathrm{2}{R}_{\mathrm{1}} \:\rightarrow\:{R}_{\mathrm{2}} \begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{\mathrm{0}}&{\mathrm{5}}&{−\mathrm{5}}&{−\mathrm{4}}\\{\mathrm{1}}&{\mathrm{2}}&{−\mathrm{5}}&{\mathrm{1}}\end{pmatrix} \\ $$$${R}_{\mathrm{3}} \:−\:{R}_{\mathrm{1}} \:\rightarrow\:{R}_{\mathrm{3}} \:\:\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{\mathrm{0}}&{\mathrm{5}}&{−\mathrm{5}}&{−\mathrm{4}}\\{\mathrm{0}}&{\mathrm{2}}&{−\mathrm{2}}&{\mathrm{4}}\end{pmatrix} \\ $$$${R}_{\mathrm{2}} \:\:\leftrightarrow\:{R}_{\mathrm{3}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{\mathrm{0}}&{\mathrm{2}}&{−\mathrm{2}}&{\mathrm{4}}\\{\mathrm{0}}&{\mathrm{5}}&{−\mathrm{5}}&{−\mathrm{4}}\end{pmatrix} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}{R}_{\mathrm{2}} \:\:\leftrightarrow\:{R}_{\mathrm{2}} \:\:\:\:\:\:\:\:\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{\mathrm{0}}&{\mathrm{1}}&{−\mathrm{1}}&{\mathrm{2}}\\{\mathrm{0}}&{\mathrm{5}}&{−\mathrm{5}}&{−\mathrm{4}}\end{pmatrix} \\ $$$${R}_{\mathrm{3}} \:−\:\mathrm{5}{R}_{\mathrm{2}} \:\rightarrow\:{R}_{\mathrm{3}} \begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{−\mathrm{3}}&{−\mathrm{3}}\\{\mathrm{0}}&{\mathrm{1}}&{−\mathrm{1}}&{\mathrm{2}}\\{\mathrm{0}}&{\mathrm{0}}&{\mathrm{0}}&{−\mathrm{14}}\end{pmatrix} \\ $$
