
Previous in Relation and Functions Next in Relation and Functions
Question Number 40882 by prof Abdo imad last updated on 28/Jul/18
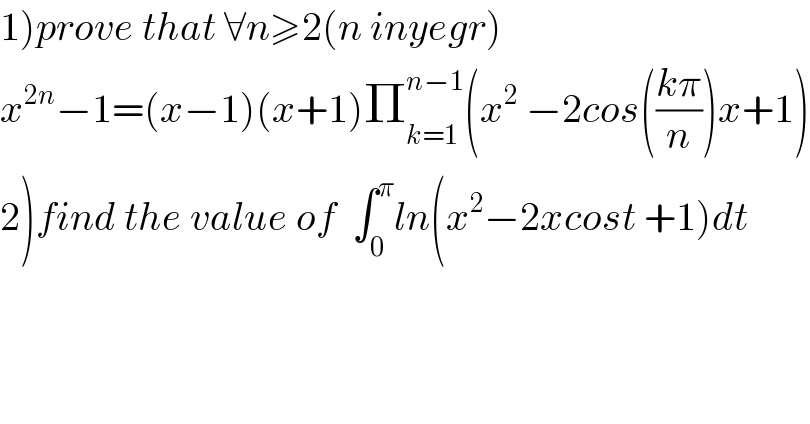
$$\left.\mathrm{1}\right){prove}\:{that}\:\forall{n}\geqslant\mathrm{2}\left({n}\:{inyegr}\right) \\ $$$${x}^{\mathrm{2}{n}} −\mathrm{1}=\left({x}−\mathrm{1}\right)\left({x}+\mathrm{1}\right)\prod_{{k}=\mathrm{1}} ^{{n}−\mathrm{1}} \left({x}^{\mathrm{2}} \:−\mathrm{2}{cos}\left(\frac{{k}\pi}{{n}}\right){x}+\mathrm{1}\right) \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\pi} {ln}\left({x}^{\mathrm{2}} −\mathrm{2}{xcost}\:+\mathrm{1}\right){dt} \\ $$
