
Question Number 40422 by rahul 19 last updated on 21/Jul/18
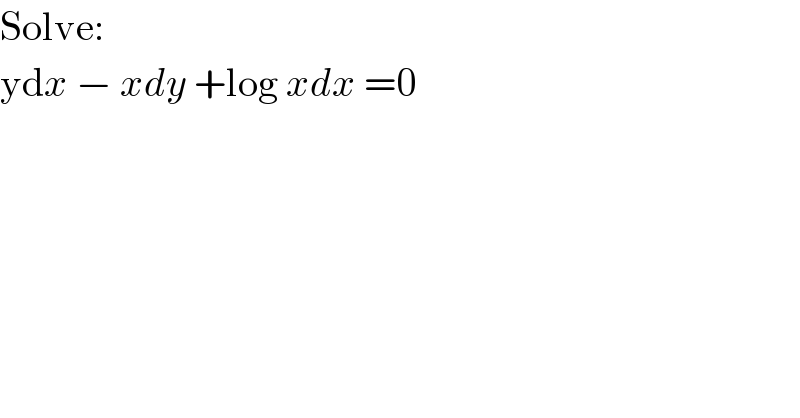
$$\mathrm{Solve}: \\ $$$$\mathrm{yd}{x}\:−\:{xdy}\:+\mathrm{log}\:{xdx}\:=\mathrm{0} \\ $$
Commented by prof Abdo imad last updated on 22/Jul/18
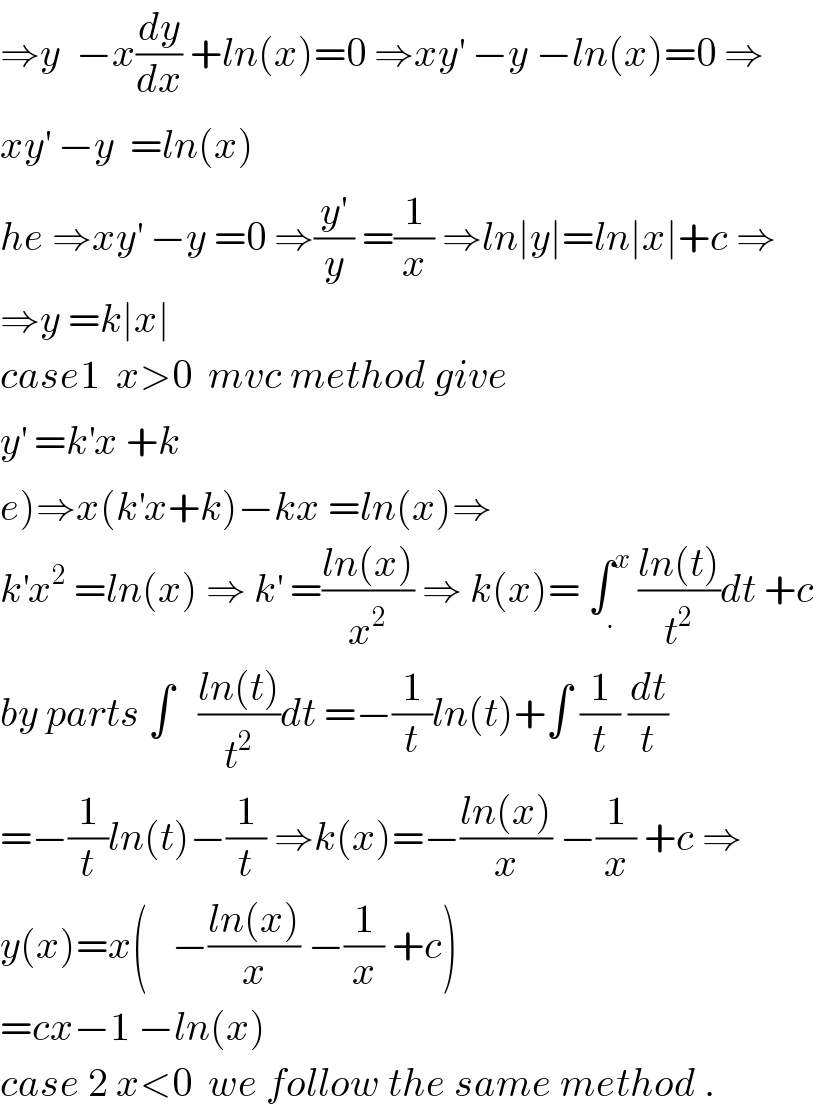
$$\Rightarrow{y}\:\:−{x}\frac{{dy}}{{dx}}\:+{ln}\left({x}\right)=\mathrm{0}\:\Rightarrow{xy}^{'} \:−{y}\:−{ln}\left({x}\right)=\mathrm{0}\:\Rightarrow \\ $$$${xy}^{'} \:−{y}\:\:={ln}\left({x}\right)\:\: \\ $$$${he}\:\Rightarrow{xy}^{'} \:−{y}\:=\mathrm{0}\:\Rightarrow\frac{{y}^{'} }{{y}}\:=\frac{\mathrm{1}}{{x}}\:\Rightarrow{ln}\mid{y}\mid={ln}\mid{x}\mid+{c}\:\Rightarrow \\ $$$$\Rightarrow{y}\:={k}\mid{x}\mid\: \\ $$$${case}\mathrm{1}\:\:{x}>\mathrm{0}\:\:{mvc}\:{method}\:{give}\: \\ $$$${y}^{'} \:={k}^{'} {x}\:+{k} \\ $$$$\left.{e}\right)\Rightarrow{x}\left({k}^{'} {x}+{k}\right)−{kx}\:={ln}\left({x}\right)\Rightarrow \\ $$$${k}^{'} {x}^{\mathrm{2}} \:={ln}\left({x}\right)\:\Rightarrow\:{k}^{'} \:=\frac{{ln}\left({x}\right)}{{x}^{\mathrm{2}} }\:\Rightarrow\:{k}\left({x}\right)=\:\int_{.} ^{{x}} \:\frac{{ln}\left({t}\right)}{{t}^{\mathrm{2}} }{dt}\:+{c} \\ $$$${by}\:{parts}\:\int\:\:\:\frac{{ln}\left({t}\right)}{{t}^{\mathrm{2}} }{dt}\:=−\frac{\mathrm{1}}{{t}}{ln}\left({t}\right)+\int\:\frac{\mathrm{1}}{{t}}\:\frac{{dt}}{{t}} \\ $$$$=−\frac{\mathrm{1}}{{t}}{ln}\left({t}\right)−\frac{\mathrm{1}}{{t}}\:\Rightarrow{k}\left({x}\right)=−\frac{{ln}\left({x}\right)}{{x}}\:−\frac{\mathrm{1}}{{x}}\:+{c}\:\Rightarrow \\ $$$${y}\left({x}\right)={x}\left(\:\:\:−\frac{{ln}\left({x}\right)}{{x}}\:−\frac{\mathrm{1}}{{x}}\:+{c}\right) \\ $$$$={cx}−\mathrm{1}\:−{ln}\left({x}\right) \\ $$$${case}\:\mathrm{2}\:{x}<\mathrm{0}\:\:{we}\:{follow}\:{the}\:{same}\:{method}\:. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 21/Jul/18
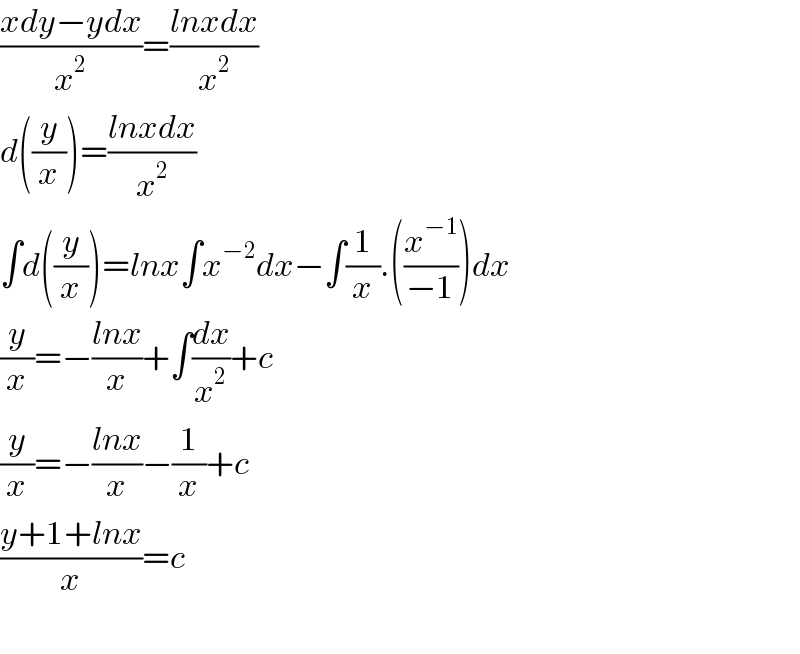
$$\frac{{xdy}−{ydx}}{{x}^{\mathrm{2}} }=\frac{{lnxdx}}{{x}^{\mathrm{2}} } \\ $$$${d}\left(\frac{{y}}{{x}}\right)=\frac{{lnxdx}}{{x}^{\mathrm{2}} } \\ $$$$\int{d}\left(\frac{{y}}{{x}}\right)={lnx}\int{x}^{−\mathrm{2}} {dx}−\int\frac{\mathrm{1}}{{x}}.\left(\frac{{x}^{−\mathrm{1}} }{−\mathrm{1}}\right){dx} \\ $$$$\frac{{y}}{{x}}=−\frac{{lnx}}{{x}}+\int\frac{{dx}}{{x}^{\mathrm{2}} }+{c} \\ $$$$\frac{{y}}{{x}}=−\frac{{lnx}}{{x}}−\frac{\mathrm{1}}{{x}}+{c} \\ $$$$\frac{{y}+\mathrm{1}+{lnx}}{{x}}={c} \\ $$$$ \\ $$
Commented by rahul 19 last updated on 21/Jul/18
thank you sir ��
