
Question Number 101985 by I want to learn more last updated on 06/Jul/20
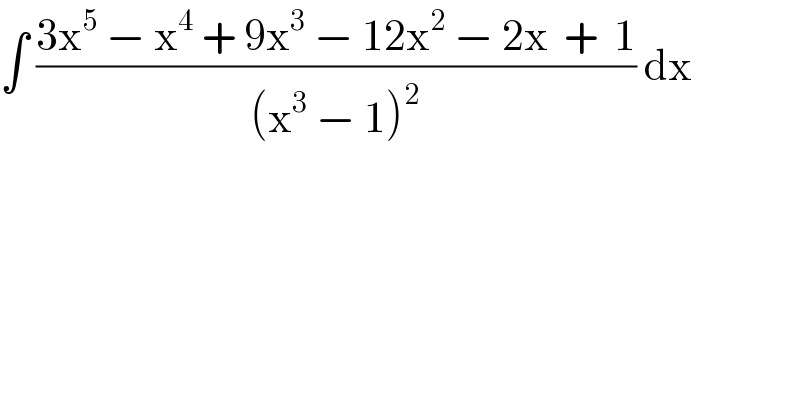
$$\int\:\frac{\mathrm{3x}^{\mathrm{5}} \:−\:\mathrm{x}^{\mathrm{4}} \:+\:\mathrm{9x}^{\mathrm{3}} \:−\:\mathrm{12x}^{\mathrm{2}} \:−\:\mathrm{2x}\:\:+\:\:\mathrm{1}}{\left(\mathrm{x}^{\mathrm{3}} \:−\:\mathrm{1}\right)^{\mathrm{2}} }\:\mathrm{dx} \\ $$
Answered by 1549442205 last updated on 06/Jul/20
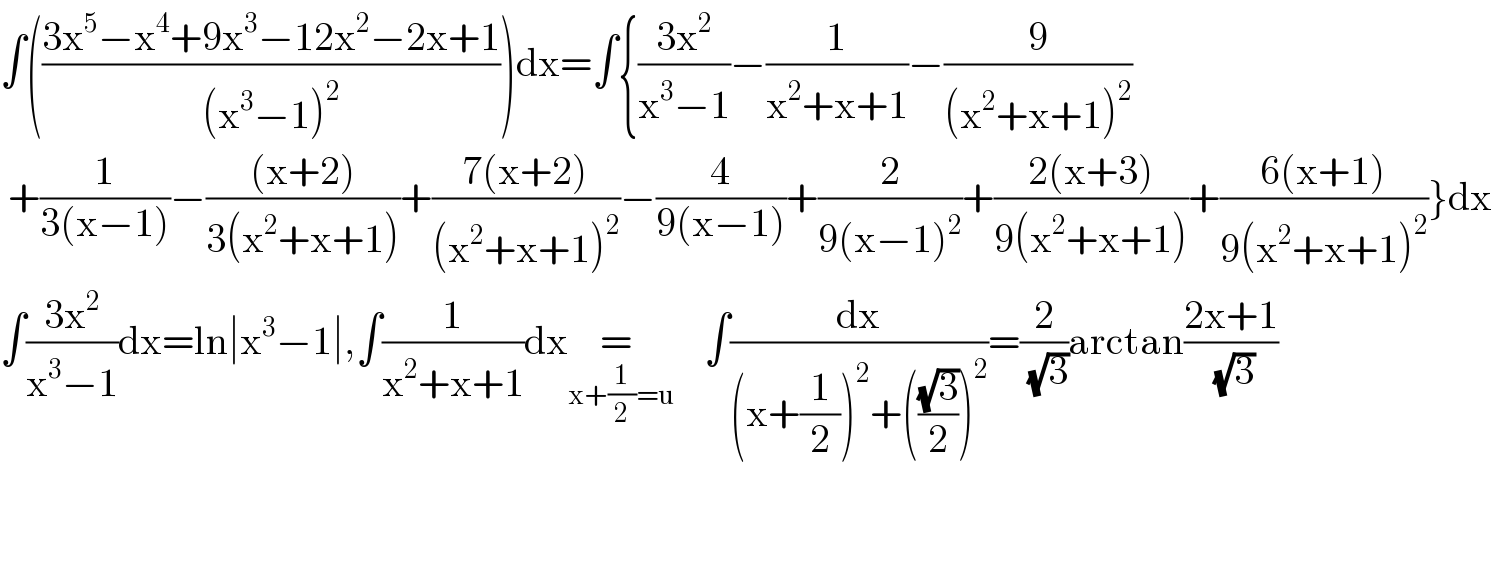
$$\int\left(\frac{\mathrm{3x}^{\mathrm{5}} −\mathrm{x}^{\mathrm{4}} +\mathrm{9x}^{\mathrm{3}} −\mathrm{12x}^{\mathrm{2}} −\mathrm{2x}+\mathrm{1}}{\left(\mathrm{x}^{\mathrm{3}} −\mathrm{1}\right)^{\mathrm{2}} }\right)\mathrm{dx}=\int\left\{\frac{\mathrm{3x}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{3}} −\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}−\frac{\mathrm{9}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} }\right. \\ $$$$\left.\:+\frac{\mathrm{1}}{\mathrm{3}\left(\mathrm{x}−\mathrm{1}\right)}−\frac{\left(\mathrm{x}+\mathrm{2}\right)}{\mathrm{3}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)}+\frac{\mathrm{7}\left(\mathrm{x}+\mathrm{2}\right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{4}}{\mathrm{9}\left(\mathrm{x}−\mathrm{1}\right)}+\frac{\mathrm{2}}{\mathrm{9}\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} }+\frac{\mathrm{2}\left(\mathrm{x}+\mathrm{3}\right)}{\mathrm{9}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)}+\frac{\mathrm{6}\left(\mathrm{x}+\mathrm{1}\right)}{\mathrm{9}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} }\right\}\mathrm{dx} \\ $$$$\int\frac{\mathrm{3x}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{3}} −\mathrm{1}}\mathrm{dx}=\mathrm{ln}\mid\mathrm{x}^{\mathrm{3}} −\mathrm{1}\mid,\int\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\mathrm{dx}\underset{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{u}} {=\:}\:\:\:\:\int\frac{\mathrm{dx}}{\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{2}} }=\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\mathrm{arctan}\frac{\mathrm{2x}+\mathrm{1}}{\sqrt{\mathrm{3}}} \\ $$$$ \\ $$$$ \\ $$
Commented by I want to learn more last updated on 07/Jul/20

$$\mathrm{Thanks}\:\mathrm{sir}. \\ $$
