
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 35347 by chakraborty ankit last updated on 18/May/18

Answered by Rasheed.Sindhi last updated on 18/May/18

$$\mathrm{Q}#\mathrm{1}\left(\mathrm{i}\right) \\ $$$$\mathrm{Let}\:\mathrm{P}_{\mathrm{1}} ,\mathrm{P}_{\mathrm{2}} ,\mathrm{P}_{\mathrm{3}} ,\mathrm{P}_{\mathrm{4}} ,\mathrm{P}_{\mathrm{5}} \:\&\:\mathrm{P}_{\mathrm{6}} \:\mathrm{are}\:\mathrm{6}\:\mathrm{vertices}. \\ $$$$\:^{\bullet} \mathrm{P}_{\mathrm{1}} \:\mathrm{can}\:\mathrm{be}\:\mathrm{joined}\:\mathrm{to}\:\mathrm{5}\:\mathrm{other}\:\mathrm{points}.\mathrm{Hence} \\ $$$$\:\:\:\mathrm{producing}\:\mathrm{5}\:\mathrm{straight}\:\mathrm{lines}. \\ $$$$\:^{\bullet} \mathrm{P}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{already}\:\mathrm{connected}\:\mathrm{to}\:\mathrm{P}_{\mathrm{1}} .\mathrm{It}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{joined}\:\mathrm{4}\:\mathrm{other}\:\mathrm{points}\:\mathrm{to}\:\mathrm{produce}\:\mathrm{4}\:\mathrm{straight} \\ $$$$\mathrm{lines}. \\ $$$$\:^{\bullet} \mathrm{P}_{\mathrm{3}} \:\mathrm{is}\:\mathrm{to}\:\mathrm{join}\:\mathrm{3}\:\mathrm{new}\:\mathrm{points}\:\mathrm{producing} \\ $$$$\mathrm{3}\:\mathrm{straight}\:\mathrm{lines}. \\ $$$$\:^{\bullet} \mathrm{P}_{\mathrm{4}\:} \mathrm{produces}\:\mathrm{2}\:\mathrm{new}\:\mathrm{straight}\:\mathrm{lines} \\ $$$$\:^{\bullet\:} \mathrm{P}_{\mathrm{5}} :\:\mathrm{1}\:\mathrm{straight}\:\mathrm{new}\:\mathrm{line}. \\ $$$$\:^{\bullet} \mathrm{P}_{\mathrm{6}} :\:\mathrm{0}\:\mathrm{new}\:\mathrm{straight}\:\mathrm{lines}. \\ $$$$\mathcal{N}\mathrm{ote}:\:{The}\:{center}\:{cannot}\:{produce}\:{extra} \\ $$$${line}\:{as}\:{it}'{s}\:{part}\:{of}\:{some}\:{existing}\:{lines} \\ $$$$\mathrm{Total}\:\mathrm{straight}\:\mathrm{lines}:\mathrm{5}+\mathrm{4}+\mathrm{3}+\mathrm{2}+\mathrm{1}+\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{15}\: \\ $$$$\mathrm{Q}#\mathrm{1}\left(\mathrm{ii}\right) \\ $$$$\mathrm{There}\:\mathrm{are}\:\mathrm{total}\:\mathrm{7}\:\mathrm{points}. \\ $$$$\mathrm{Number}\:\mathrm{of}\:\mathrm{triplets}=\begin{pmatrix}{\mathrm{7}}\\{\mathrm{3}}\end{pmatrix}=\frac{\mathrm{7}.\mathrm{6}.\mathrm{5}}{\mathrm{1}.\mathrm{2}.\mathrm{3}}=\mathrm{35} \\ $$$$\mathrm{3}\:\mathrm{triplets}\:\mathrm{are}\:\mathrm{not}\:\mathrm{traingles}.\mathrm{They}\:\mathrm{are} \\ $$$$\mathrm{straight}\:\mathrm{lines}. \\ $$$$\mathrm{Hence}\:\mathrm{number}\:\mathrm{of}\:\mathrm{triangles}=\mathrm{35}−\mathrm{3}=\mathrm{32} \\ $$
Commented by Rasheed.Sindhi last updated on 18/May/18
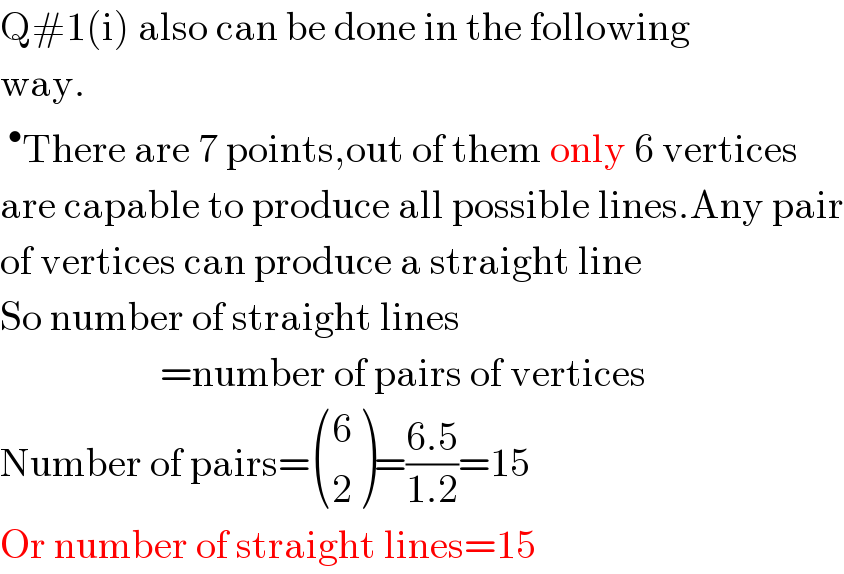
$$\mathrm{Q}#\mathrm{1}\left(\mathrm{i}\right)\:\mathrm{also}\:\mathrm{can}\:\mathrm{be}\:\mathrm{done}\:\mathrm{in}\:\mathrm{the}\:\mathrm{following} \\ $$$$\mathrm{way}. \\ $$$$\:^{\bullet} \mathrm{There}\:\mathrm{are}\:\mathrm{7}\:\mathrm{points},\mathrm{out}\:\mathrm{of}\:\mathrm{them}\:\mathrm{only}\:\mathrm{6}\:\mathrm{vertices} \\ $$$$\mathrm{are}\:\mathrm{capable}\:\mathrm{to}\:\mathrm{produce}\:\mathrm{all}\:\mathrm{possible}\:\mathrm{lines}.\mathrm{Any}\:\mathrm{pair} \\ $$$$\mathrm{of}\:\mathrm{vertices}\:\mathrm{can}\:\mathrm{produce}\:\mathrm{a}\:\mathrm{straight}\:\mathrm{line} \\ $$$$\mathrm{So}\:\mathrm{number}\:\mathrm{of}\:\mathrm{straight}\:\mathrm{lines} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{number}\:\mathrm{of}\:\mathrm{pairs}\:\mathrm{of}\:\mathrm{vertices} \\ $$$$\mathrm{Number}\:\mathrm{of}\:\mathrm{pairs}=\begin{pmatrix}{\mathrm{6}}\\{\mathrm{2}}\end{pmatrix}=\frac{\mathrm{6}.\mathrm{5}}{\mathrm{1}.\mathrm{2}}=\mathrm{15} \\ $$$$\mathrm{Or}\:\mathrm{number}\:\mathrm{of}\:\mathrm{straight}\:\mathrm{lines}=\mathrm{15} \\ $$
Answered by Rasheed.Sindhi last updated on 18/May/18

$$\mathrm{Q}#\mathrm{2}\: \\ $$$$\bigstar\mathrm{Join}\:\mathrm{of}\:\mathrm{two}\:\mathrm{non}-\mathrm{consecutive}\:\mathrm{vertices} \\ $$$$\mathrm{is}\:\mathrm{defined}\:\mathrm{as}\:\boldsymbol{\mathrm{diagonal}}\:\mathrm{of}\:\mathrm{polygon}. \\ $$$$\bigstar\mathrm{Number}\:\mathrm{of}\:\mathrm{non}-\mathrm{consecutive}\:\mathrm{vertices} \\ $$$$\mathrm{to}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{vertex}\:\mathrm{in}\:\mathrm{an}\:\mathrm{n}-\mathrm{gon}\:\mathrm{is}\: \\ $$$$\mathrm{n}−\mathrm{3}\:\left(\mathrm{3}\:\mathrm{points}\:\mathrm{are}\:\mathrm{consecutive}\right) \\ $$$$\:\:\:\mathrm{So}\:\mathrm{in}\:\mathrm{octagon}\:\mathrm{to}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{vertex} \\ $$$$\:\:\:\:\:\mathrm{8}−\mathrm{2}=\mathrm{6}\:\mathrm{non}-\mathrm{consecutive}\:\mathrm{vertices}. \\ $$$$\mathrm{Let}\:\mathrm{the}\:\mathrm{vertices}\:\mathrm{are}\:\mathrm{P}_{\mathrm{1}} ,\mathrm{P}_{\mathrm{2}} ,...,\mathrm{P}_{\mathrm{8}} \\ $$$$\divideontimes\mathrm{Begin}\:\mathrm{with}\:\mathrm{P}_{\mathrm{1}} :\mathrm{P}_{\mathrm{1}} \mathrm{can}\:\mathrm{be}\:\mathrm{joined}\:\mathrm{P}_{\mathrm{3}} ,...,\mathrm{P}_{\mathrm{7}} \\ $$$$\mathrm{in}\:\mathrm{order}\:\mathrm{to}\:\mathrm{produce}\:\mathrm{5}\:\mathrm{diagonals}. \\ $$$$\left(\mathrm{Of}\:\mathrm{course}\:\mathrm{P}_{\mathrm{1}} \mathrm{P}_{\mathrm{2}} \:\&\:\mathrm{P}_{\mathrm{1}} \mathrm{P}_{\mathrm{8}} \:\mathrm{are}\:\mathrm{not}\:\mathrm{diagonals}\right. \\ $$$$\left.\mathrm{because}\:\mathrm{P}_{\mathrm{2}} \:\&\:\mathrm{P}_{\mathrm{8}} \:\mathrm{are}\:\mathrm{consecutive}\:\mathrm{to}\:\mathrm{P}_{\mathrm{1}} \right) \\ $$$$\divideontimes\mathrm{Similarly}\:\mathrm{P}_{\mathrm{2}} \:\mathrm{can}\:\mathrm{be}\:\mathrm{joined}\:\mathrm{all}\:\mathrm{other} \\ $$$$\mathrm{points}\:\mathrm{except}\:\mathrm{P}_{\mathrm{1}\:} \&\:\mathrm{P}_{\mathrm{3}} \:\mathrm{to}\:\mathrm{produce}\:\mathrm{5}\:\mathrm{new} \\ $$$$\mathrm{diagonals}. \\ $$$$\divideontimes\mathrm{P}_{\mathrm{3}} ,\mathrm{however}\:\mathrm{already}\:\mathrm{conected}\:\mathrm{to}\:\mathrm{P}_{\mathrm{1}} \\ $$$$\mathrm{so}\:\mathrm{the}\:\mathrm{diagonal}\:\:\mathrm{P}_{\mathrm{3}} \mathrm{P}_{\mathrm{1}} \:\mathrm{is}\:\mathrm{already}\:\mathrm{counted}. \\ $$$$\mathrm{Hence}\:\mathrm{it}\:\mathrm{produces}\:\mathrm{4}\:\mathrm{new}\:\mathrm{diagonals}. \\ $$$$\divideontimes\mathrm{In}\:\mathrm{this}\:\mathrm{way} \\ $$$$\:\:\:\mathrm{P}_{\mathrm{4}} \:\mathrm{produces}\:\mathrm{3}\:\mathrm{new}\:\mathrm{diagonals}. \\ $$$$\:\:\:\mathrm{P}_{\mathrm{5}} \:\mathrm{produces}\:\mathrm{2}\:\mathrm{new}\:\mathrm{diagonals}. \\ $$$$\:\:\:\mathrm{P}_{\mathrm{6}} \:\mathrm{produces}\:\mathrm{1}\:\mathrm{new}\:\mathrm{diagonals}. \\ $$$$\:\:\:\:\mathrm{P}_{\mathrm{7}} \:\&\:\mathrm{P}_{\mathrm{8}} \:\mathrm{produce}\:\mathrm{no}\:\mathrm{new}\:\mathrm{diagonal}. \\ $$$$\:\mathrm{Total}\:\mathrm{of}\:\mathrm{diagonals}=\mathrm{5}+\mathrm{5}+\mathrm{4}+\mathrm{3}+\mathrm{2}+\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{20} \\ $$
Answered by Rasheed.Sindhi last updated on 19/May/18

$$\mathrm{Q}#\mathrm{3}\: \\ $$$$\:^{\bullet} \:\mathrm{3}\:\mathrm{collinear}\:\mathrm{points}\:\mathrm{produce}\:\mathrm{1}\:\mathrm{line}. \\ $$$$\:\:\mathrm{Each}\:\mathrm{of}\:\mathrm{three}\:\mathrm{points}\:\mathrm{can}\:\mathrm{be}\:\mathrm{joined}\:\mathrm{to} \\ $$$$\:\:\:\mathrm{4}+\mathrm{5}=\mathrm{9}\:\mathrm{points} \\ $$$$\:\:\:\mathrm{Each}\:\mathrm{point}\:\mathrm{produce}\:\mathrm{9}\:\mathrm{lines} \\ $$$$\:\:\:\:\mathrm{All}\:\mathrm{3}\:\mathrm{points}\:\mathrm{produce}\:\mathrm{9}×\mathrm{3}=\mathrm{27}\:\mathrm{lines}. \\ $$$$\:^{\bullet} \:\mathrm{4}\:\mathrm{collinear}\:\mathrm{points}\:\mathrm{produce}\:\mathrm{1}\:\mathrm{line}. \\ $$$$\:\:\:\:\mathrm{Each}\:\mathrm{of}\:\mathrm{4}\:\mathrm{points}\:\mathrm{can}\:\mathrm{be}\:\mathrm{joined}\:\mathrm{to} \\ $$$$\:\:\:\:\mathrm{5}\:\mathrm{points}\:\mathrm{to}\:\mathrm{produce}\:\boldsymbol{\mathrm{new}}\:\mathrm{lines} \\ $$$$\:\:\:\mathrm{Each}\:\mathrm{point}\:\mathrm{produce}\:\mathrm{5}\:\mathrm{lines}. \\ $$$$\:\:\:\:\mathrm{All}\:\mathrm{4}\:\mathrm{points}\:\mathrm{produce}\:\mathrm{5}×\mathrm{4}=\mathrm{20}\:\boldsymbol{\mathrm{new}}\:\mathrm{lines}. \\ $$$$\:^{\bullet} \:\mathrm{5}\:\mathrm{collinear}\:\mathrm{points}\:\mathrm{produce}\:\mathrm{1}\:\mathrm{line}. \\ $$$$\:\:\:\:\mathrm{All}\:\mathrm{the}\:\mathrm{lines}\:\mathrm{produced}\:\mathrm{by}\:\mathrm{these} \\ $$$$\:\:\:\:\:\mathrm{points}\:\mathrm{have}\:\mathrm{already}\:\mathrm{been}\:\mathrm{counted}. \\ $$$$\:\:\:\:\:\mathrm{So}\:\mathrm{produce}\:\mathrm{0}\:\boldsymbol{\mathrm{new}}\:\mathrm{line}. \\ $$$$\:\:\mathrm{Number}\:\mathrm{of}\:\mathrm{all}\:\mathrm{possible}\:\mathrm{lines}=\: \\ $$$$\:\:\:\:\mathrm{1}+\mathrm{27}+\mathrm{1}+\mathrm{20}+\mathrm{1}=\mathrm{50} \\ $$$$\:\:\:\: \\ $$
Commented by chakraborty ankit last updated on 21/May/18

$${thank}\:{a}\:{lot}\:{sir} \\ $$
