
Question Number 3465 by Rasheed Soomro last updated on 13/Dec/15

$${Find}\:{the}\:{value}\:{of}\:{n}\:{so}\:{that} \\ $$$$\:\:\:\:\:\:\:\:\:\:\frac{{a}^{{n}+\mathrm{1}} +{b}^{{n}+\mathrm{1}} }{{a}^{{n}} +{b}^{{n}} } \\ $$$${may}\:{become}\:{the}\:{G}.{M}.\:{between}\: \\ $$$${a}\:\:{and}\:\:\:{b}. \\ $$
Answered by prakash jain last updated on 13/Dec/15

$$\frac{{a}^{{n}+\mathrm{1}} +{b}^{{n}+\mathrm{1}} }{{a}^{{n}} +{b}^{{n}} }=\sqrt{{ab}} \\ $$$${a}/{b}={k}\Rightarrow{a}={kb} \\ $$$$\frac{{k}^{{n}+\mathrm{1}} +\mathrm{1}}{{k}^{{n}} +\mathrm{1}}{b}={bk}^{\mathrm{1}/\mathrm{2}} \\ $$$$\frac{{k}^{{n}+\mathrm{1}} +\mathrm{1}}{{k}^{{n}} +\mathrm{1}}={k}^{\mathrm{1}/\mathrm{2}} \\ $$$$\frac{{k}^{\mathrm{2}{n}+\mathrm{2}} +\mathrm{1}+\mathrm{2}{k}^{{n}+\mathrm{1}} }{{k}^{\mathrm{2}{n}} +\mathrm{1}+\mathrm{2}{k}^{{n}} }={k} \\ $$$${k}^{\mathrm{2}{n}+\mathrm{2}} +\mathrm{1}+\mathrm{2}{k}^{{n}+\mathrm{1}} ={k}^{\mathrm{2}{n}+\mathrm{1}} +{k}+\mathrm{2}{k}^{{n}+\mathrm{1}} \\ $$$${k}^{\mathrm{2}{n}+\mathrm{2}} +\mathrm{1}={k}^{\mathrm{2}{n}+\mathrm{1}} +{k} \\ $$$$\Rightarrow{n}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$
Commented by prakash jain last updated on 13/Dec/15
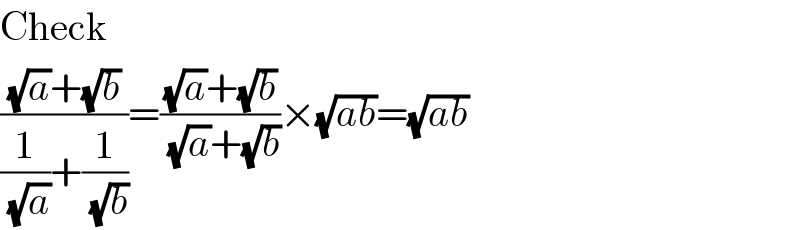
$$\mathrm{Check} \\ $$$$\frac{\sqrt{{a}}+\sqrt{{b}}}{\frac{\mathrm{1}}{\sqrt{{a}}}+\frac{\mathrm{1}}{\sqrt{{b}}}}=\frac{\sqrt{{a}}+\sqrt{{b}}}{\sqrt{{a}}+\sqrt{{b}}}×\sqrt{{ab}}=\sqrt{{ab}} \\ $$
Answered by RasheedSindhi last updated on 14/Dec/15
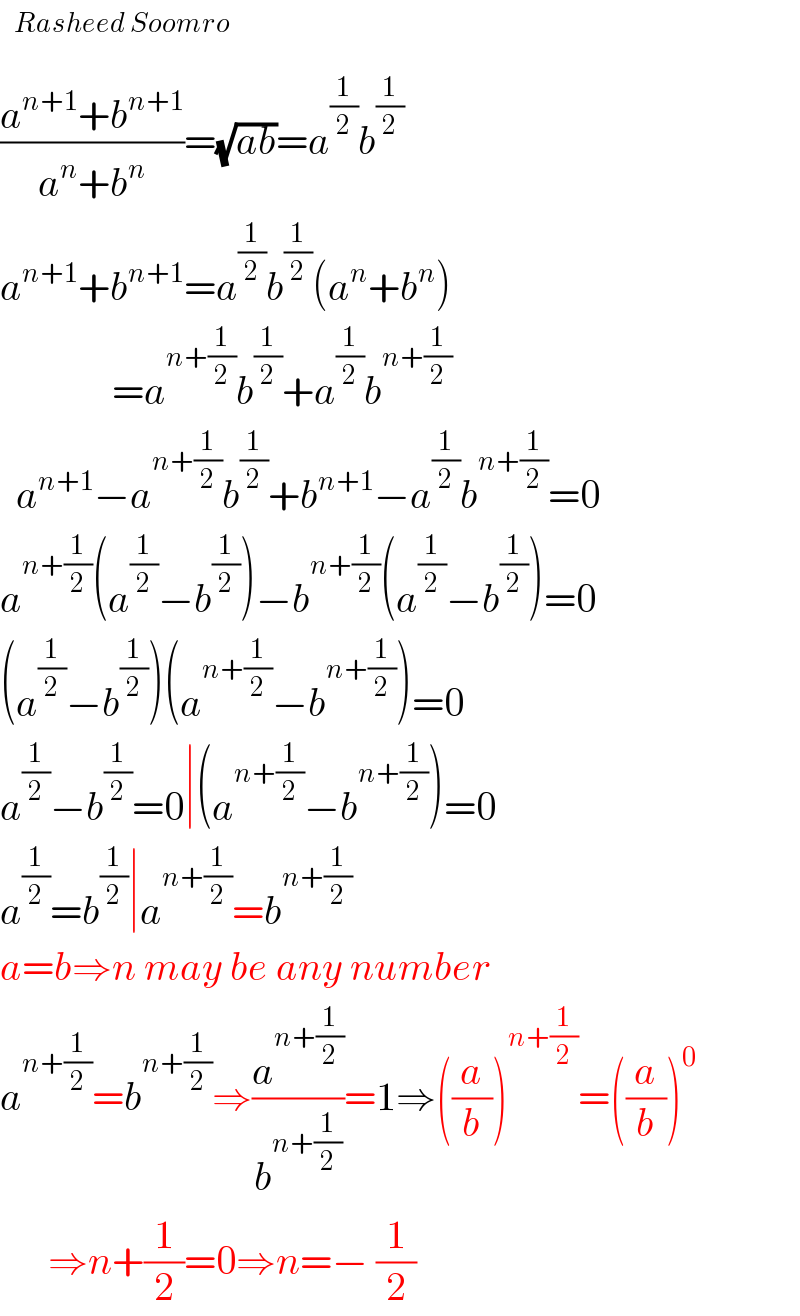
$$ \\ $$$$\frac{{a}^{{n}+\mathrm{1}} +{b}^{{n}+\mathrm{1}} }{{a}^{{n}} +{b}^{{n}} }=\sqrt{{ab}}={a}^{\frac{\mathrm{1}}{\mathrm{2}}} {b}^{\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${a}^{{n}+\mathrm{1}} +{b}^{{n}+\mathrm{1}} ={a}^{\frac{\mathrm{1}}{\mathrm{2}}} {b}^{\frac{\mathrm{1}}{\mathrm{2}}} \left({a}^{{n}} +{b}^{{n}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:={a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} {b}^{\frac{\mathrm{1}}{\mathrm{2}}} +{a}^{\frac{\mathrm{1}}{\mathrm{2}}} {b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\:\:{a}^{{n}+\mathrm{1}} −{a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} {b}^{\frac{\mathrm{1}}{\mathrm{2}}} +{b}^{{n}+\mathrm{1}} −{a}^{\frac{\mathrm{1}}{\mathrm{2}}} {b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} =\mathrm{0} \\ $$$${a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \left({a}^{\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{\frac{\mathrm{1}}{\mathrm{2}}} \right)−{b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \left({a}^{\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{\frac{\mathrm{1}}{\mathrm{2}}} \right)=\mathrm{0} \\ $$$$\left({a}^{\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{\frac{\mathrm{1}}{\mathrm{2}}} \right)\left({a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \right)=\mathrm{0} \\ $$$${a}^{\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{\frac{\mathrm{1}}{\mathrm{2}}} =\mathrm{0}\mid\left({a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} −{b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \right)=\mathrm{0} \\ $$$${a}^{\frac{\mathrm{1}}{\mathrm{2}}} ={b}^{\frac{\mathrm{1}}{\mathrm{2}}} \mid{a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} ={b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${a}={b}\Rightarrow{n}\:{may}\:{be}\:{any}\:{number} \\ $$$${a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} ={b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} \Rightarrow\frac{{a}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} }{{b}^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} }=\mathrm{1}\Rightarrow\left(\frac{{a}}{{b}}\right)^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} =\left(\frac{{a}}{{b}}\right)^{\mathrm{0}} \\ $$$$\:\:\:\:\:\:\Rightarrow{n}+\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0}\Rightarrow{n}=−\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
