Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 200718 by hardmath last updated on 22/Nov/23

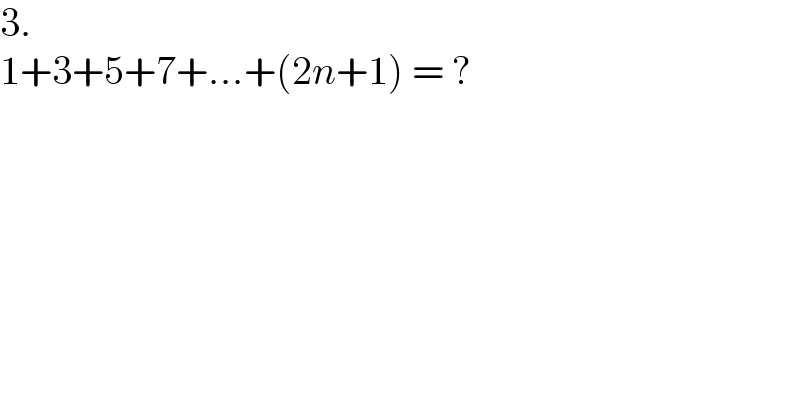
3. 1+3+5+7+...+(2n+1) = ?

$$\mathrm{3}. \\ $$$$\mathrm{1}+\mathrm{3}+\mathrm{5}+\mathrm{7}+...+\left(\mathrm{2}{n}+\mathrm{1}\right)\:=\:? \\ $$

Answered by deleteduser1 last updated on 22/Nov/23

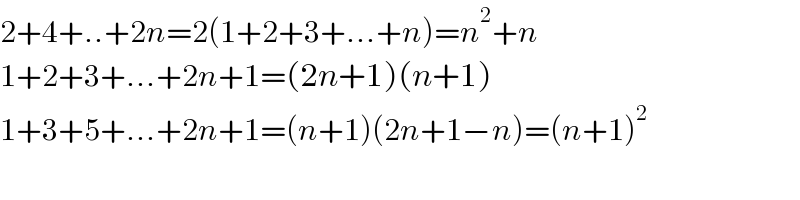
2+4+..+2n=2(1+2+3+...+n)=n^2 +n 1+2+3+...+2n+1=(2n+1)(n+1) 1+3+5+...+2n+1=(n+1)(2n+1−n)=(n+1)^2

$$\mathrm{2}+\mathrm{4}+..+\mathrm{2}{n}=\mathrm{2}\left(\mathrm{1}+\mathrm{2}+\mathrm{3}+...+{n}\right)={n}^{\mathrm{2}} +{n} \\ $$$$\mathrm{1}+\mathrm{2}+\mathrm{3}+...+\mathrm{2}{n}+\mathrm{1}=\left(\mathrm{2}{n}+\mathrm{1}\right)\left({n}+\mathrm{1}\right) \\ $$$$\mathrm{1}+\mathrm{3}+\mathrm{5}+...+\mathrm{2}{n}+\mathrm{1}=\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}−{n}\right)=\left({n}+\mathrm{1}\right)^{\mathrm{2}} \\ $$

Answered by mr W last updated on 22/Nov/23

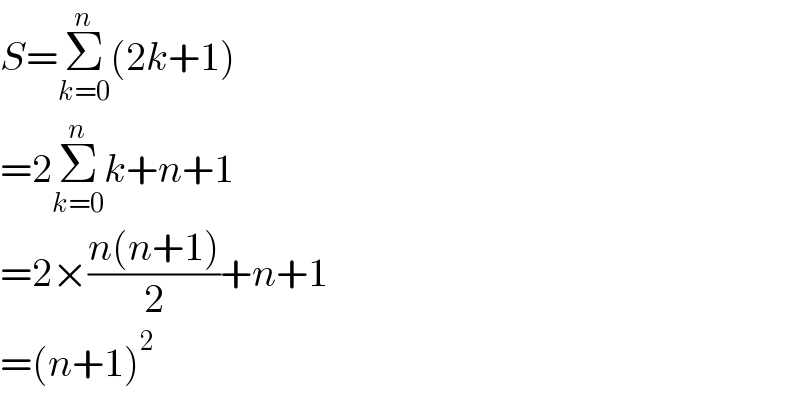
S=Σ_(k=0) ^n (2k+1) =2Σ_(k=0) ^n k+n+1 =2×((n(n+1))/2)+n+1 =(n+1)^2

$${S}=\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}\left(\mathrm{2}{k}+\mathrm{1}\right) \\ $$$$=\mathrm{2}\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{k}+{n}+\mathrm{1} \\ $$$$=\mathrm{2}×\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}+{n}+\mathrm{1} \\ $$$$=\left({n}+\mathrm{1}\right)^{\mathrm{2}} \\ $$

Answered by Mathspace last updated on 23/Nov/23

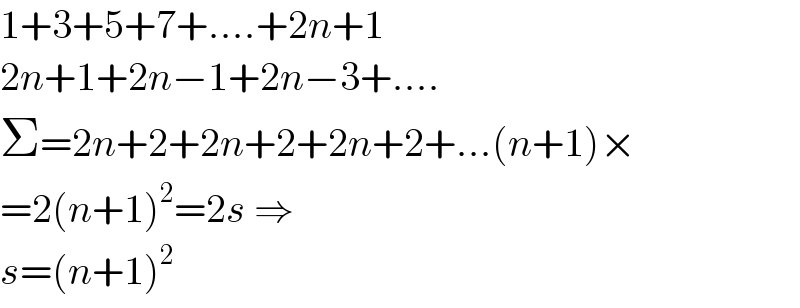
1+3+5+7+....+2n+1 2n+1+2n−1+2n−3+.... Σ=2n+2+2n+2+2n+2+...(n+1)× =2(n+1)^2 =2s ⇒ s=(n+1)^2

$$\mathrm{1}+\mathrm{3}+\mathrm{5}+\mathrm{7}+....+\mathrm{2}{n}+\mathrm{1} \\ $$$$\mathrm{2}{n}+\mathrm{1}+\mathrm{2}{n}−\mathrm{1}+\mathrm{2}{n}−\mathrm{3}+.... \\ $$$$\Sigma=\mathrm{2}{n}+\mathrm{2}+\mathrm{2}{n}+\mathrm{2}+\mathrm{2}{n}+\mathrm{2}+...\left({n}+\mathrm{1}\right)× \\ $$$$=\mathrm{2}\left({n}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{2}{s}\:\Rightarrow \\ $$$${s}=\left({n}+\mathrm{1}\right)^{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com