Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 161675 by cortano last updated on 21/Dec/21

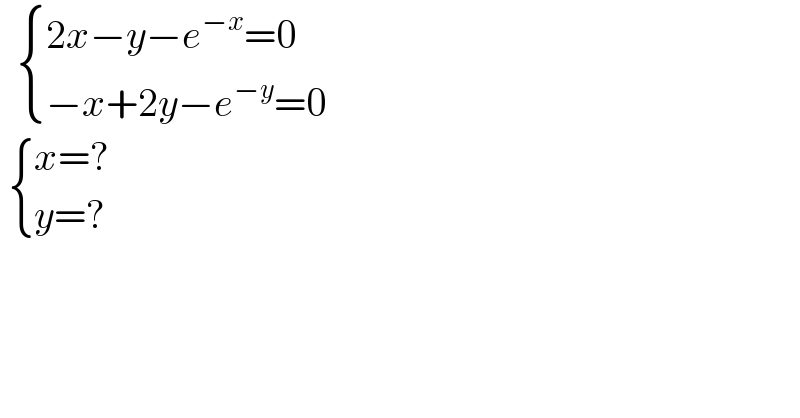
{ ((2x−y−e^(−x) =0)),((−x+2y−e^(−y) =0)) :} { ((x=?)),((y=?)) :}

$$\:\:\begin{cases}{\mathrm{2}{x}−{y}−{e}^{−{x}} =\mathrm{0}}\\{−{x}+\mathrm{2}{y}−{e}^{−{y}} =\mathrm{0}}\end{cases} \\ $$$$\:\begin{cases}{{x}=?}\\{{y}=?}\end{cases} \\ $$

Answered by mr W last updated on 21/Dec/21

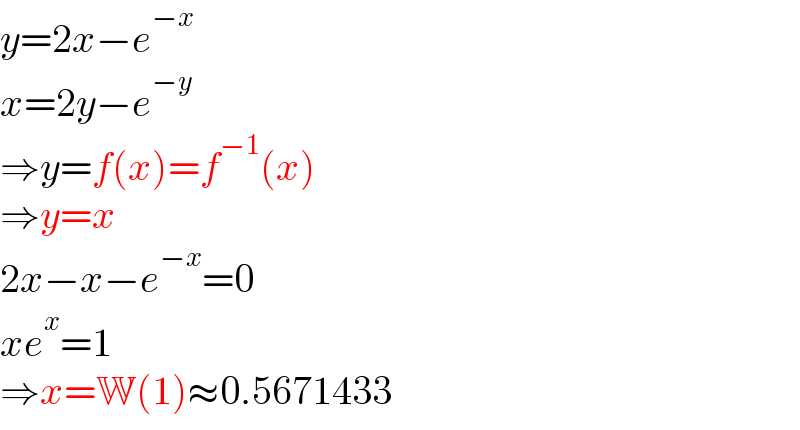
y=2x−e^(−x) x=2y−e^(−y) ⇒y=f(x)=f^(−1) (x) ⇒y=x 2x−x−e^(−x) =0 xe^x =1 ⇒x=W(1)≈0.5671433

$${y}=\mathrm{2}{x}−{e}^{−{x}} \\ $$$${x}=\mathrm{2}{y}−{e}^{−{y}} \\ $$$$\Rightarrow{y}={f}\left({x}\right)={f}^{−\mathrm{1}} \left({x}\right) \\ $$$$\Rightarrow{y}={x} \\ $$$$\mathrm{2}{x}−{x}−{e}^{−{x}} =\mathrm{0} \\ $$$${xe}^{{x}} =\mathrm{1} \\ $$$$\Rightarrow{x}=\mathbb{W}\left(\mathrm{1}\right)\approx\mathrm{0}.\mathrm{5671433} \\ $$

Commented by cortano last updated on 21/Dec/21

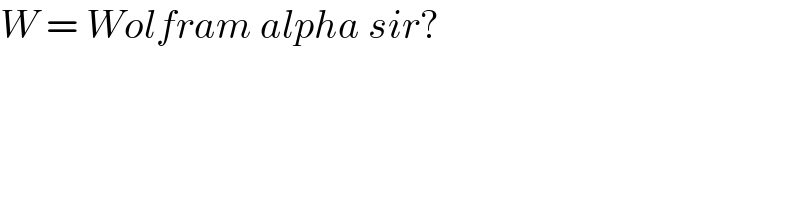
W = Wolfram alpha sir?

$${W}\:=\:{Wolfram}\:{alpha}\:{sir}? \\ $$

Commented by cortano last updated on 21/Dec/21

hahaha...i′m sorry

$${hahaha}...{i}'{m}\:{sorry} \\ $$

Commented by Ar Brandon last updated on 21/Dec/21

������

Commented by Ar Brandon last updated on 21/Dec/21

Lambert′s function

$$\mathrm{Lambert}'\mathrm{s}\:\mathrm{function} \\ $$

Commented by mr W last updated on 21/Dec/21

mr W has nothing to do with Wolfram alpha/beta/gamma /delta/omicron etc. :)

$${mr}\:\boldsymbol{\mathcal{W}}\:{has}\:{nothing}\:{to}\:{do}\:{with} \\ $$$${Wolfram}\:{alpha}/{beta}/{gamma} \\ $$$$\left./{delta}/{omicron}\:{etc}.\:\::\right) \\ $$

Commented by Ar Brandon last updated on 21/Dec/21

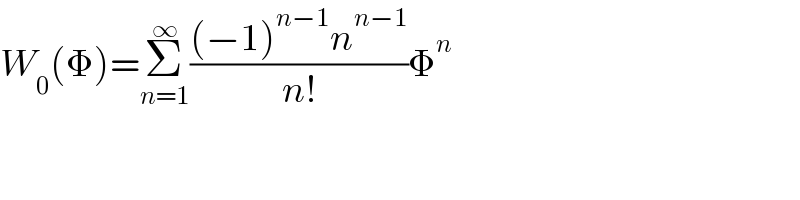
W_0 (Φ)=Σ_(n=1) ^∞ (((−1)^(n−1) n^(n−1) )/(n!))Φ^n

$${W}_{\mathrm{0}} \left(\Phi\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} {n}^{{n}−\mathrm{1}} }{{n}!}\Phi^{{n}} \: \\ $$

Commented by Ar Brandon last updated on 21/Dec/21

��

Commented by cortano last updated on 21/Dec/21

haha covid 19

$${haha}\:{covid}\:\mathrm{19} \\ $$

Commented by aleks041103 last updated on 24/Dec/21

Isn′t it f(x)=f^(−1) (x)⇒f(f(x))=x and not y=x ⇒x=2f(x)−e^(−f(x)) = =2(2x−e^(−x) )−e^(−(2x−e^(−x) )) = =4x−2e^(−x) −e^(−2x) e^e^(−x) =x e^e^(−x) e^(−2x) +2e^(−x) =3x if x=W(1) then xe^x =1⇒x=e^(−x) ⇒e^x x^2 +2x=3x e^x x=1 ⇒ no error since really x=W(1)

$${Isn}'{t}\:{it} \\ $$$${f}\left({x}\right)={f}^{−\mathrm{1}} \left({x}\right)\Rightarrow{f}\left({f}\left({x}\right)\right)={x}\:{and}\:{not}\:{y}={x} \\ $$$$\Rightarrow{x}=\mathrm{2}{f}\left({x}\right)−{e}^{−{f}\left({x}\right)} = \\ $$$$=\mathrm{2}\left(\mathrm{2}{x}−{e}^{−{x}} \right)−{e}^{−\left(\mathrm{2}{x}−{e}^{−{x}} \right)} = \\ $$$$=\mathrm{4}{x}−\mathrm{2}{e}^{−{x}} −{e}^{−\mathrm{2}{x}} {e}^{{e}^{−{x}} } ={x} \\ $$$${e}^{{e}^{−{x}} } {e}^{−\mathrm{2}{x}} +\mathrm{2}{e}^{−{x}} =\mathrm{3}{x} \\ $$$${if}\:{x}={W}\left(\mathrm{1}\right)\:{then}\:{xe}^{{x}} =\mathrm{1}\Rightarrow{x}={e}^{−{x}} \\ $$$$\Rightarrow{e}^{{x}} {x}^{\mathrm{2}} +\mathrm{2}{x}=\mathrm{3}{x} \\ $$$${e}^{{x}} {x}=\mathrm{1}\:\Rightarrow\:{no}\:{error}\:{since}\:{really}\:{x}={W}\left(\mathrm{1}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com