
Question Number 97476 by M±th+et+s last updated on 08/Jun/20
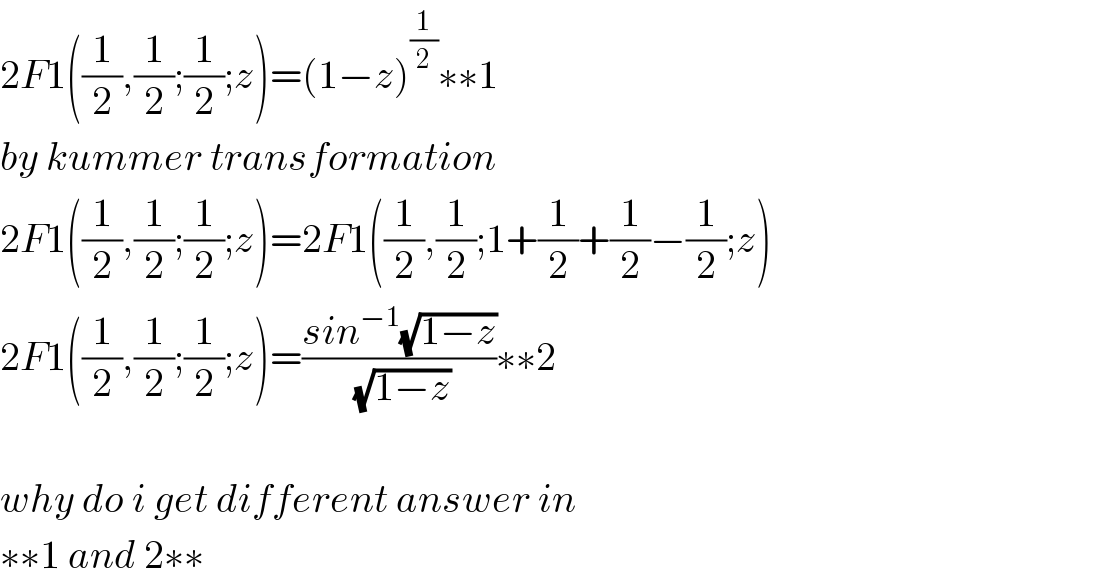
$$\mathrm{2}{F}\mathrm{1}\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}};\frac{\mathrm{1}}{\mathrm{2}};{z}\right)=\left(\mathrm{1}−{z}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \ast\ast\mathrm{1} \\ $$$${by}\:{kummer}\:{transformation} \\ $$$$\mathrm{2}{F}\mathrm{1}\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}};\frac{\mathrm{1}}{\mathrm{2}};{z}\right)=\mathrm{2}{F}\mathrm{1}\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}};\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}};{z}\right) \\ $$$$\mathrm{2}{F}\mathrm{1}\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}};\frac{\mathrm{1}}{\mathrm{2}};{z}\right)=\frac{{sin}^{−\mathrm{1}} \sqrt{\mathrm{1}−{z}}}{\sqrt{\mathrm{1}−{z}}}\ast\ast\mathrm{2} \\ $$$$ \\ $$$${why}\:{do}\:{i}\:{get}\:{different}\:{answer}\:{in} \\ $$$$\ast\ast\mathrm{1}\:{and}\:\mathrm{2}\ast\ast \\ $$
