Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 60577 by naka3546 last updated on 22/May/19

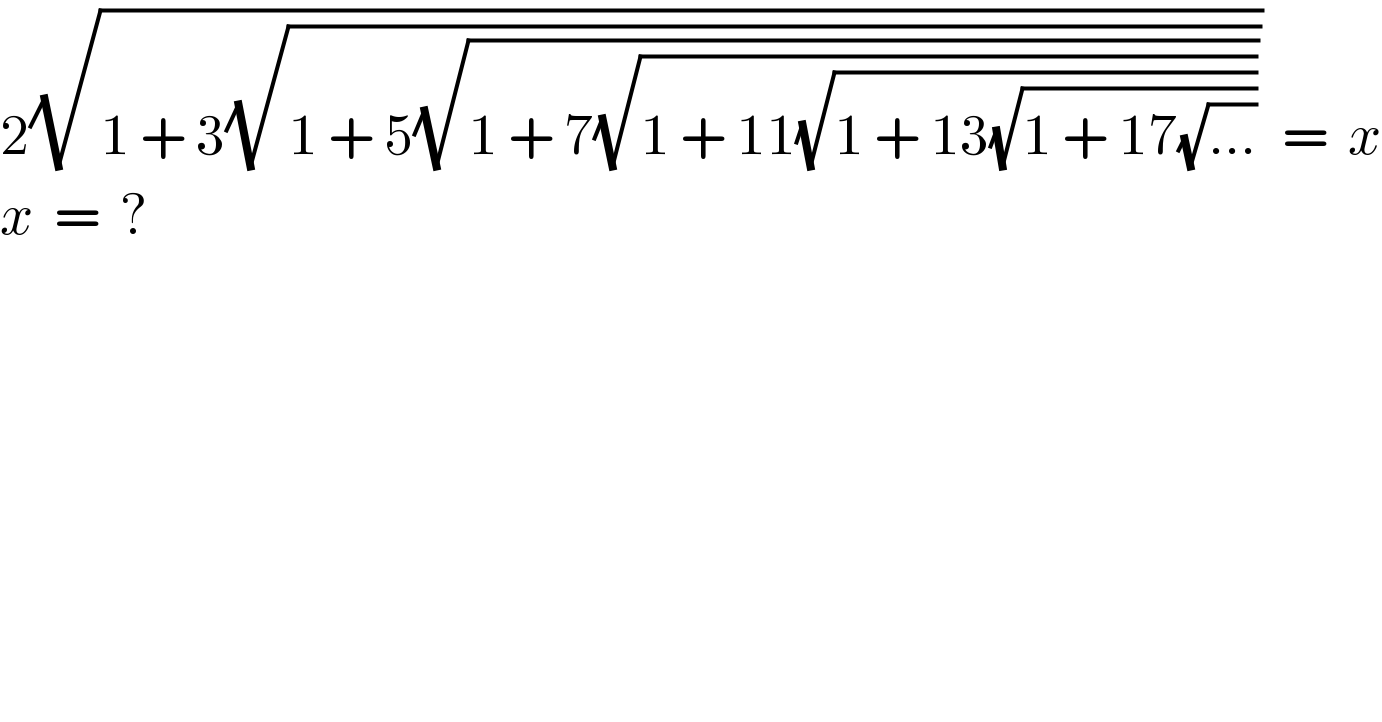
2(√(1 + 3(√(1 + 5(√(1 + 7(√(1 + 11(√(1 + 13(√(1 + 17(√(...)))))))))))))) = x x = ?

$$\mathrm{2}\sqrt{\mathrm{1}\:+\:\mathrm{3}\sqrt{\mathrm{1}\:+\:\mathrm{5}\sqrt{\mathrm{1}\:+\:\mathrm{7}\sqrt{\mathrm{1}\:+\:\mathrm{11}\sqrt{\mathrm{1}\:+\:\mathrm{13}\sqrt{\mathrm{1}\:+\:\mathrm{17}\sqrt{...}}}}}}}\:\:=\:\:{x} \\ $$$${x}\:\:=\:\:? \\ $$

Commented by ajfour last updated on 22/May/19

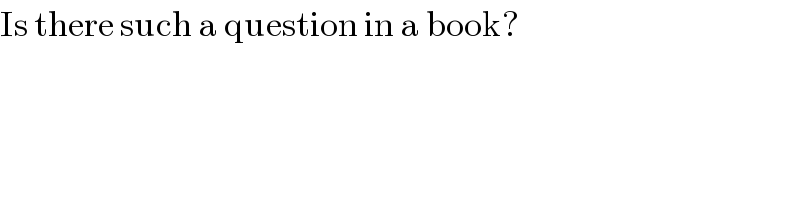
Is there such a question in a book?

$$\mathrm{Is}\:\mathrm{there}\:\mathrm{such}\:\mathrm{a}\:\mathrm{question}\:\mathrm{in}\:\mathrm{a}\:\mathrm{book}? \\ $$

Answered by Prithwish sen last updated on 22/May/19

(n+1)(n+3)=(n+1)(√((n+3)^2 )) =(n+1)(√(1+(n+2)(n+4))) =(n+1)(√(1+(n+2)(√((n+4)^2 )))) =(n+1)(√(1+(n+2)(√(1+(n+3)(n+5))))) =(n+1)(√(1+(n+2)(√(1+(n+3)(√((n+5)^2 )))))) and so on putting n=l 8=2(√(1+3(√(1+4(√(1+5(√(1+.......)))))))) is the qiestion ok?

$$\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{n}+\mathrm{3}\right)=\left(\mathrm{n}+\mathrm{1}\right)\sqrt{\left(\mathrm{n}+\mathrm{3}\right)^{\mathrm{2}} } \\ $$$$=\left(\mathrm{n}+\mathrm{1}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{2}\right)\left(\mathrm{n}+\mathrm{4}\right)} \\ $$$$=\left(\mathrm{n}+\mathrm{1}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{2}\right)\sqrt{\left(\mathrm{n}+\mathrm{4}\right)^{\mathrm{2}} }} \\ $$$$=\left(\mathrm{n}+\mathrm{1}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{2}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{3}\right)\left(\mathrm{n}+\mathrm{5}\right)}} \\ $$$$=\left(\mathrm{n}+\mathrm{1}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{2}\right)\sqrt{\mathrm{1}+\left(\mathrm{n}+\mathrm{3}\right)\sqrt{\left(\mathrm{n}+\mathrm{5}\right)^{\mathrm{2}} }}} \\ $$$$\mathrm{and}\:\mathrm{so}\:\mathrm{on} \\ $$$$\mathrm{putting}\:\mathrm{n}=\mathrm{l} \\ $$$$\mathrm{8}=\mathrm{2}\sqrt{\mathrm{1}+\mathrm{3}\sqrt{\mathrm{1}+\mathrm{4}\sqrt{\mathrm{1}+\mathrm{5}\sqrt{\mathrm{1}+.......}}}} \\ $$$$\mathrm{is}\:\mathrm{the}\:\mathrm{qiestion}\:\mathrm{ok}? \\ $$

Commented by ajfour last updated on 22/May/19

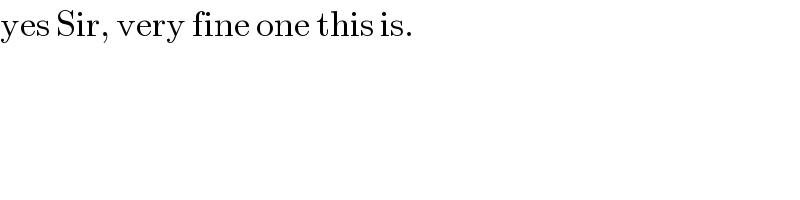
yes Sir, very fine one this is.

$$\mathrm{yes}\:\mathrm{Sir},\:\mathrm{very}\:\mathrm{fine}\:\mathrm{one}\:\mathrm{this}\:\mathrm{is}. \\ $$

Commented by Prithwish sen last updated on 22/May/19

thank you.

$$\mathrm{thank}\:\mathrm{you}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com