
Question Number 199170 by tri26112004 last updated on 28/Oct/23
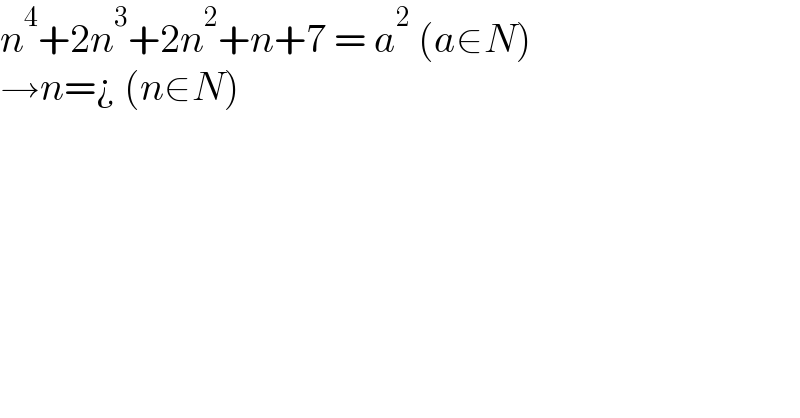
$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}\:=\:{a}^{\mathrm{2}} \:\left({a}\in{N}\right) \\ $$$$\rightarrow{n}=¿\:\left({n}\in{N}\right) \\ $$
Commented by Rasheed.Sindhi last updated on 31/Oct/23

$$\mathbb{G}\boldsymbol{\mathrm{reat}}! \\ $$$$\:\mathcal{T}{han}\mathcal{X}\:\mathcal{S}{ir}\: \\ $$
Commented by Rasheed.Sindhi last updated on 30/Oct/23
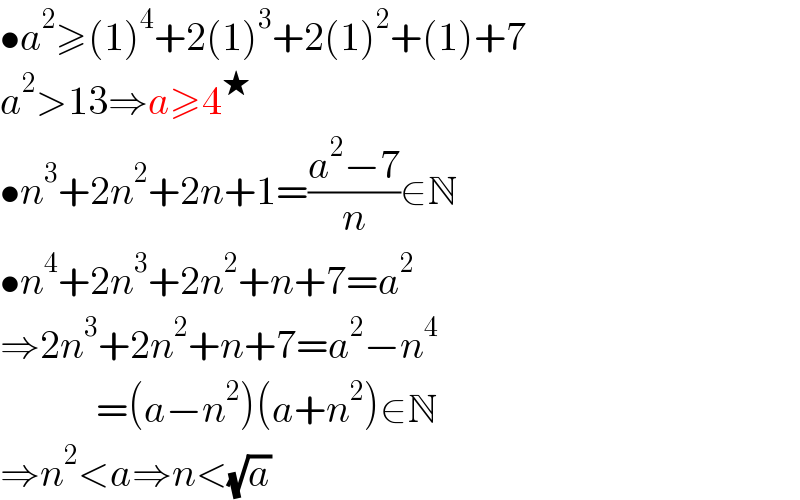
$$\bullet{a}^{\mathrm{2}} \geqslant\left(\mathrm{1}\right)^{\mathrm{4}} +\mathrm{2}\left(\mathrm{1}\right)^{\mathrm{3}} +\mathrm{2}\left(\mathrm{1}\right)^{\mathrm{2}} +\left(\mathrm{1}\right)+\mathrm{7} \\ $$$${a}^{\mathrm{2}} >\mathrm{13}\Rightarrow{a}\geqslant\mathrm{4}^{\bigstar} \\ $$$$\bullet{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +\mathrm{2}{n}+\mathrm{1}=\frac{{a}^{\mathrm{2}} −\mathrm{7}}{{n}}\in\mathbb{N} \\ $$$$\bullet{n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}={a}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}={a}^{\mathrm{2}} −{n}^{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\left({a}−{n}^{\mathrm{2}} \right)\left({a}+{n}^{\mathrm{2}} \right)\in\mathbb{N} \\ $$$$\Rightarrow{n}^{\mathrm{2}} <{a}\Rightarrow{n}<\sqrt{{a}}\: \\ $$
Commented by Rasheed.Sindhi last updated on 30/Oct/23
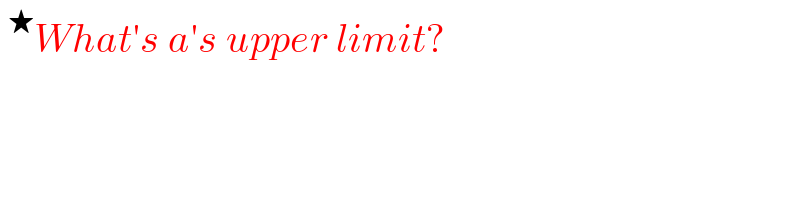
$$\:^{\bigstar} {What}'{s}\:{a}'{s}\:{upper}\:{limit}? \\ $$
Commented by Frix last updated on 30/Oct/23
![SOLUTION Part 1 We can factorize it. n^4 +2n^3 +2n^3 +n−a^2 +7=0 n=t−(1/2) t^4 +(t^2 /2)−a^2 +((109)/(16))=0 It′s easy to get n=−(1/2)±((√(−1±2(√(4a^2 −27))))/2) [_(of the ± signs) ^(all 4 combinations) ] n∈R^+ ⇒ n=−(1/2)+((√(−1+2(√(4a^2 −27))))/2) ⇒ for a∈R 4a^2 −27≥0∧−1+2(√(4a^2 −27))≥0 ⇒ a≤−((√(109))/4)∨a≥((√(109))/4) a∈N ⇒ a≥3 No upper limit.](Q199248.png)
$$\mathbb{SOLUTION}\:\mathrm{Part}\:\mathrm{1} \\ $$$$\mathrm{We}\:\mathrm{can}\:\mathrm{factorize}\:\mathrm{it}. \\ $$$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{3}} +{n}−{a}^{\mathrm{2}} +\mathrm{7}=\mathrm{0} \\ $$$${n}={t}−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${t}^{\mathrm{4}} +\frac{{t}^{\mathrm{2}} }{\mathrm{2}}−{a}^{\mathrm{2}} +\frac{\mathrm{109}}{\mathrm{16}}=\mathrm{0} \\ $$$$\mathrm{It}'\mathrm{s}\:\mathrm{easy}\:\mathrm{to}\:\mathrm{get} \\ $$$${n}=−\frac{\mathrm{1}}{\mathrm{2}}\pm\frac{\sqrt{−\mathrm{1}\pm\mathrm{2}\sqrt{\mathrm{4}{a}^{\mathrm{2}} −\mathrm{27}}}}{\mathrm{2}}\:\left[_{\mathrm{of}\:\mathrm{the}\:\pm\:\mathrm{signs}} ^{\mathrm{all}\:\mathrm{4}\:\mathrm{combinations}} \right] \\ $$$${n}\in\mathbb{R}^{+} \:\Rightarrow\:{n}=−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\sqrt{−\mathrm{1}+\mathrm{2}\sqrt{\mathrm{4}{a}^{\mathrm{2}} −\mathrm{27}}}}{\mathrm{2}} \\ $$$$\Rightarrow\:\mathrm{for}\:{a}\in\mathbb{R} \\ $$$$\mathrm{4}{a}^{\mathrm{2}} −\mathrm{27}\geqslant\mathrm{0}\wedge−\mathrm{1}+\mathrm{2}\sqrt{\mathrm{4}{a}^{\mathrm{2}} −\mathrm{27}}\geqslant\mathrm{0} \\ $$$$\Rightarrow\:{a}\leqslant−\frac{\sqrt{\mathrm{109}}}{\mathrm{4}}\vee{a}\geqslant\frac{\sqrt{\mathrm{109}}}{\mathrm{4}} \\ $$$${a}\in\mathbb{N}\:\Rightarrow\:{a}\geqslant\mathrm{3} \\ $$$$\mathrm{No}\:\mathrm{upper}\:\mathrm{limit}. \\ $$
Commented by Rasheed.Sindhi last updated on 30/Oct/23
$$\boldsymbol{\mathcal{T}{hanks}}\:\boldsymbol{{Frix}}\:\boldsymbol{{sir}}! \\ $$
Commented by Frix last updated on 30/Oct/23

$$\mathbb{SOLUTION}\:\mathrm{Part}\:\mathrm{2} \\ $$$$\mathrm{4}{a}^{\mathrm{2}} −\mathrm{27}={b}^{\mathrm{2}} \\ $$$$\mathrm{4}{a}^{\mathrm{2}} −{b}^{\mathrm{2}} =\mathrm{27} \\ $$$$\left(\mathrm{2}{a}−{b}\right)\left(\mathrm{2}{a}+{b}\right)=\mathrm{27} \\ $$$$\mathrm{2}{a}−{b}=\mathrm{1}\wedge\mathrm{2}{a}+{b}=\mathrm{27}\:\Rightarrow\:{a}=\mathrm{7}\wedge{b}=\mathrm{13}\:\Rightarrow\:{n}=\mathrm{2}\:\bigstar \\ $$$$\mathrm{2}{a}−{b}=\mathrm{3}\wedge\mathrm{2}{a}+{b}=\mathrm{9}\:\Rightarrow\:{a}=\mathrm{3}\wedge{b}=\mathrm{3}\:\Rightarrow\:{n}\notin\mathbb{N} \\ $$$$\mathrm{No}\:\mathrm{other}\:\mathrm{possibilities}. \\ $$
Commented by Frix last updated on 30/Oct/23
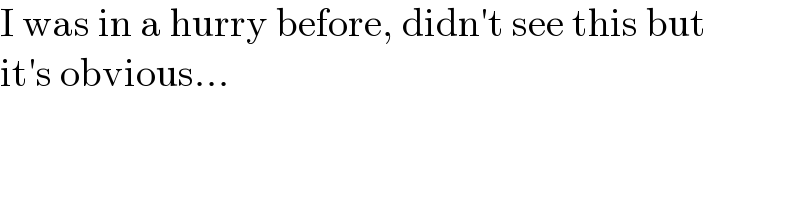
$$\mathrm{I}\:\mathrm{was}\:\mathrm{in}\:\mathrm{a}\:\mathrm{hurry}\:\mathrm{before},\:\mathrm{didn}'\mathrm{t}\:\mathrm{see}\:\mathrm{this}\:\mathrm{but} \\ $$$$\mathrm{it}'\mathrm{s}\:\mathrm{obvious}... \\ $$
Commented by Frix last updated on 31/Oct/23
��
Commented by tri26112004 last updated on 01/Nov/23

$${a}\geqslant\mathrm{4}\:{and}\:{n}<\sqrt{{a}} \\ $$$${It}'{s}\:{not}\:{OK} \\ $$
Answered by Frix last updated on 28/Oct/23
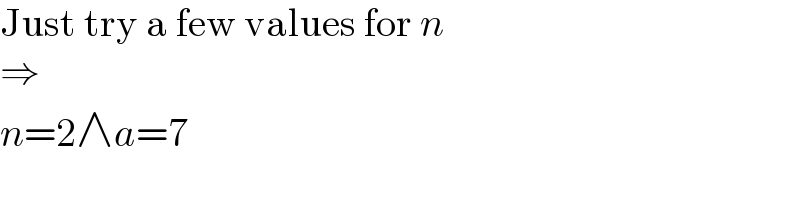
$$\mathrm{Just}\:\mathrm{try}\:\mathrm{a}\:\mathrm{few}\:\mathrm{values}\:\mathrm{for}\:{n} \\ $$$$\Rightarrow \\ $$$${n}=\mathrm{2}\wedge{a}=\mathrm{7} \\ $$
Commented by tri26112004 last updated on 29/Oct/23
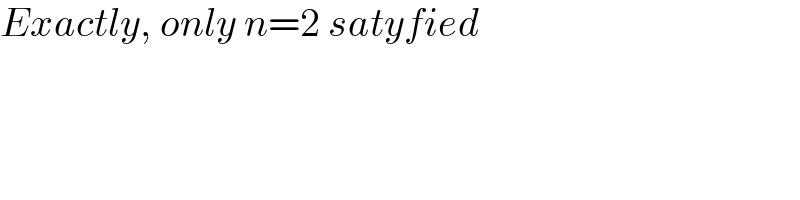
$${Exactly},\:{only}\:{n}=\mathrm{2}\:{satyfied} \\ $$
Answered by Rasheed.Sindhi last updated on 29/Oct/23
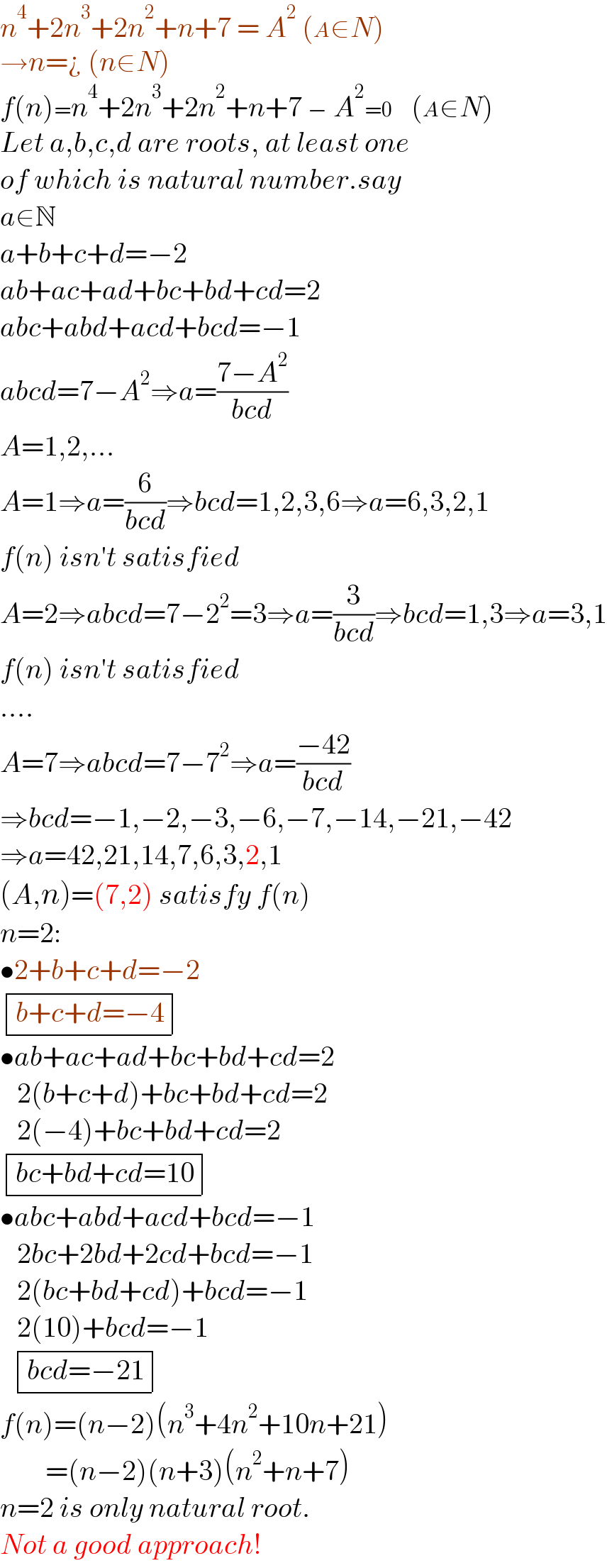
$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}\:=\:{A}^{\mathrm{2}} \:\left({A}\in{N}\right) \\ $$$$\rightarrow{n}=¿\:\left({n}\in{N}\right) \\ $$$${f}\left({n}\right)={n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}\:−\:{A}^{\mathrm{2}} =\mathrm{0}\:\:\:\:\left({A}\in{N}\right) \\ $$$${Let}\:{a},{b},{c},{d}\:{are}\:{roots},\:{at}\:{least}\:{one} \\ $$$${of}\:{which}\:{is}\:{natural}\:{number}.{say} \\ $$$${a}\in\mathbb{N} \\ $$$${a}+{b}+{c}+{d}=−\mathrm{2}\: \\ $$$${ab}+{ac}+{ad}+{bc}+{bd}+{cd}=\mathrm{2} \\ $$$${abc}+{abd}+{acd}+{bcd}=−\mathrm{1} \\ $$$${abcd}=\mathrm{7}−{A}^{\mathrm{2}} \Rightarrow{a}=\frac{\mathrm{7}−{A}^{\mathrm{2}} }{{bcd}} \\ $$$${A}=\mathrm{1},\mathrm{2},... \\ $$$${A}=\mathrm{1}\Rightarrow{a}=\frac{\mathrm{6}}{{bcd}}\Rightarrow{bcd}=\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{6}\Rightarrow{a}=\mathrm{6},\mathrm{3},\mathrm{2},\mathrm{1}\: \\ $$$${f}\left({n}\right)\:{isn}'{t}\:{satisfied} \\ $$$${A}=\mathrm{2}\Rightarrow{abcd}=\mathrm{7}−\mathrm{2}^{\mathrm{2}} =\mathrm{3}\Rightarrow{a}=\frac{\mathrm{3}}{{bcd}}\Rightarrow{bcd}=\mathrm{1},\mathrm{3}\Rightarrow{a}=\mathrm{3},\mathrm{1} \\ $$$${f}\left({n}\right)\:{isn}'{t}\:{satisfied} \\ $$$$.... \\ $$$${A}=\mathrm{7}\Rightarrow{abcd}=\mathrm{7}−\mathrm{7}^{\mathrm{2}} \Rightarrow{a}=\frac{−\mathrm{42}}{{bcd}}\: \\ $$$$\Rightarrow{bcd}=−\mathrm{1},−\mathrm{2},−\mathrm{3},−\mathrm{6},−\mathrm{7},−\mathrm{14},−\mathrm{21},−\mathrm{42} \\ $$$$\Rightarrow{a}=\mathrm{42},\mathrm{21},\mathrm{14},\mathrm{7},\mathrm{6},\mathrm{3},\mathrm{2},\mathrm{1} \\ $$$$\left({A},{n}\right)=\left(\mathrm{7},\mathrm{2}\right)\:{satisfy}\:{f}\left({n}\right) \\ $$$${n}=\mathrm{2}: \\ $$$$\bullet\mathrm{2}+{b}+{c}+{d}=−\mathrm{2} \\ $$$$\begin{array}{|c|}{{b}+{c}+{d}=−\mathrm{4}}\\\hline\end{array} \\ $$$$\bullet{ab}+{ac}+{ad}+{bc}+{bd}+{cd}=\mathrm{2} \\ $$$$\:\:\:\mathrm{2}\left({b}+{c}+{d}\right)+{bc}+{bd}+{cd}=\mathrm{2} \\ $$$$\:\:\:\mathrm{2}\left(−\mathrm{4}\right)+{bc}+{bd}+{cd}=\mathrm{2} \\ $$$$\begin{array}{|c|}{{bc}+{bd}+{cd}=\mathrm{10}}\\\hline\end{array} \\ $$$$\bullet{abc}+{abd}+{acd}+{bcd}=−\mathrm{1} \\ $$$$\:\:\:\mathrm{2}{bc}+\mathrm{2}{bd}+\mathrm{2}{cd}+{bcd}=−\mathrm{1} \\ $$$$\:\:\:\mathrm{2}\left({bc}+{bd}+{cd}\right)+{bcd}=−\mathrm{1} \\ $$$$\:\:\:\mathrm{2}\left(\mathrm{10}\right)+{bcd}=−\mathrm{1} \\ $$$$\:\:\begin{array}{|c|}{{bcd}=−\mathrm{21}}\\\hline\end{array} \\ $$$${f}\left({n}\right)=\left({n}−\mathrm{2}\right)\left({n}^{\mathrm{3}} +\mathrm{4}{n}^{\mathrm{2}} +\mathrm{10}{n}+\mathrm{21}\right) \\ $$$$\:\:\:\:\:\:\:\:=\left({n}−\mathrm{2}\right)\left({n}+\mathrm{3}\right)\left({n}^{\mathrm{2}} +{n}+\mathrm{7}\right) \\ $$$${n}=\mathrm{2}\:{is}\:{only}\:{natural}\:{root}. \\ $$$${Not}\:{a}\:{good}\:{approach}! \\ $$
Commented by Rasheed.Sindhi last updated on 29/Oct/23

$${Once}\:{we}\:{found}\:{n}=\mathrm{2}\:\wedge\:{A}=\mathrm{7} \\ $$$${We}'{ve}\:{to}\:{prove}\:{that}\:{there}'{s}\:{no} \\ $$$${other}\:{natural}\:{root}\:{of}\:{f}\left({n}\right). \\ $$$${For}\:{this}\:{put}\:{A}=\mathrm{7}\:{in}\:{f}\left({n}\right)\:{and} \\ $$$${then}\:{trying}\:{to}\:{solve}\:{f}\left({n}\right)\:{whose} \\ $$$${one}\:{factor}\:{is}\:{already}\:{known}:\:{n}−\mathrm{2} \\ $$$$ \\ $$$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}\:−\:{A}^{\mathrm{2}} =\mathrm{0}\:\:\:\:\left({A}\in{N}\right) \\ $$$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}+\mathrm{7}\:−\:\mathrm{7}^{\mathrm{2}} =\mathrm{0}\:\:\:\: \\ $$$${n}^{\mathrm{4}} +\mathrm{2}{n}^{\mathrm{3}} +\mathrm{2}{n}^{\mathrm{2}} +{n}−\mathrm{42}=\mathrm{0}\:\:\:\: \\ $$$$\left({n}−\mathrm{2}\right)\left({n}+\mathrm{3}\right)\left({n}^{\mathrm{2}} +{n}+\mathrm{7}\right)=\mathrm{0} \\ $$$$\therefore\:{n}=\mathrm{2}\:{is}\:{only}\:{natural}\:{root}. \\ $$$$ \\ $$
