
Question Number 197585 by Erico last updated on 23/Sep/23
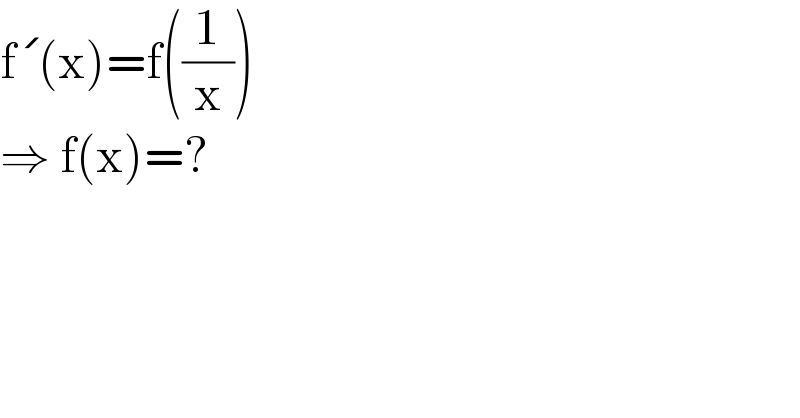
$$\mathrm{f}\: \left(\mathrm{x}\right)=\mathrm{f}\left(\frac{\mathrm{1}}{\mathrm{x}}\right) \\ $$$$\Rightarrow\:\mathrm{f}\left(\mathrm{x}\right)=? \\ $$
Answered by EdwarT last updated on 24/Sep/23

$$−\frac{\mathrm{1}}{{x}^{\mathrm{2}} } \\ $$
Answered by Erico last updated on 24/Sep/23
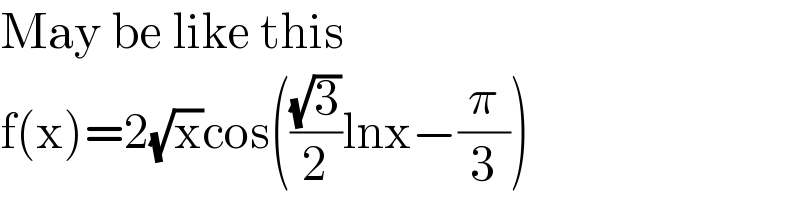
$$\mathrm{May}\:\mathrm{be}\:\mathrm{like}\:\mathrm{this} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{2}\sqrt{\mathrm{x}}\mathrm{cos}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mathrm{lnx}−\frac{\pi}{\mathrm{3}}\right) \\ $$
Answered by Frix last updated on 24/Sep/23
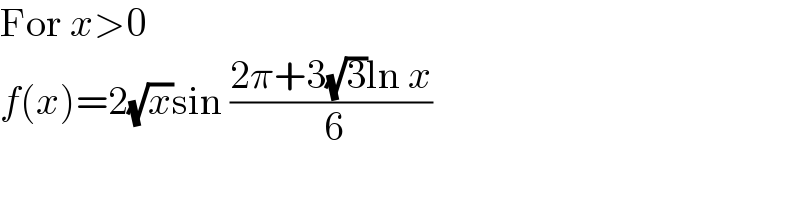
$$\mathrm{For}\:{x}>\mathrm{0} \\ $$$${f}\left({x}\right)=\mathrm{2}\sqrt{{x}}\mathrm{sin}\:\frac{\mathrm{2}\pi+\mathrm{3}\sqrt{\mathrm{3}}\mathrm{ln}\:{x}}{\:\mathrm{6}} \\ $$
Answered by witcher3 last updated on 24/Sep/23
)+((b/2)+(a/( 2))(√3))cos(((ln(x))/(2 ))(√3))) =(1/( (√x)))(asin(((ln((1/x)))/( 2))(√3))+bcos(((ln((1/x)))/2)(√3))),∀x>0 ⇒(a/2)−(b/( 2))(√3)=−a (b/2)+(a/( 2))(√3)=b b=a(√3) f(x)=a(√x)(sin(((ln(x)(√3))/2))+(√3)cos(((ln(x)(√3))/2))) =2a(√x)(sin(((ln(x)(√3))/2)+(π/3))),a∈R](Q197626.png)
$$\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{f}\left(\frac{\mathrm{1}}{\mathrm{x}}\right) \\ $$$$\mathrm{f}''\left(\mathrm{x}\right)=−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\mathrm{f}'\left(\frac{\mathrm{1}}{\mathrm{x}}\right)=−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\mathrm{f}\left(\mathrm{x}\right) \\ $$$$\Leftrightarrow\mathrm{x}^{\mathrm{2}} \mathrm{f}''\left(\mathrm{x}\right)+\mathrm{f}\left(\mathrm{x}\right)=\mathrm{0} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{r}} \\ $$$$\Rightarrow\left(\mathrm{r}\left(\mathrm{r}−\mathrm{1}\right)+\mathrm{1}\right)\mathrm{x}^{\mathrm{r}} =\mathrm{0} \\ $$$$\Rightarrow\mathrm{r}^{\mathrm{2}} −\mathrm{r}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{r}=\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}},\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\frac{\mathrm{1}}{\mathrm{2}}} \left(\mathrm{ax}^{\frac{\mathrm{i}}{\:\mathrm{2}\sqrt{\mathrm{3}}}} +\mathrm{bx}^{−\frac{\mathrm{i}}{\mathrm{2}\:\sqrt{\mathrm{3}}}} \right) \\ $$$$=\sqrt{\mathrm{x}}\left(\mathrm{asin}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{2}\:}\sqrt{\mathrm{3}}\right)+\mathrm{bcos}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{2}}\sqrt{\mathrm{3}}\right)\right. \\ $$$$ \\ $$$$\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{f}\left(\frac{\mathrm{1}}{\mathrm{x}}\right) \\ $$$$\Rightarrow \\ $$$$\left.\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}}}\left(\left(\frac{\mathrm{a}}{\mathrm{2}}−\frac{\mathrm{b}}{\:\mathrm{2}}\sqrt{\mathrm{3}}\right)\mathrm{sin}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{2}\:}\right]\sqrt{\mathrm{3}}\right)+\left(\frac{\mathrm{b}}{\mathrm{2}}+\frac{\mathrm{a}}{\:\mathrm{2}}\sqrt{\mathrm{3}}\right)\mathrm{cos}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{2}\:}\sqrt{\mathrm{3}}\right)\right) \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{x}}}\left(\mathrm{asin}\left(\frac{\mathrm{ln}\left(\frac{\mathrm{1}}{\mathrm{x}}\right)}{\:\mathrm{2}}\sqrt{\mathrm{3}}\right)+\mathrm{bcos}\left(\frac{\mathrm{ln}\left(\frac{\mathrm{1}}{\mathrm{x}}\right)}{\mathrm{2}}\sqrt{\mathrm{3}}\right)\right),\forall\mathrm{x}>\mathrm{0} \\ $$$$\Rightarrow\frac{\mathrm{a}}{\mathrm{2}}−\frac{\mathrm{b}}{\:\mathrm{2}}\sqrt{\mathrm{3}}=−\mathrm{a} \\ $$$$\frac{\mathrm{b}}{\mathrm{2}}+\frac{\mathrm{a}}{\:\mathrm{2}}\sqrt{\mathrm{3}}=\mathrm{b} \\ $$$$\mathrm{b}=\mathrm{a}\sqrt{\mathrm{3}} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{a}\sqrt{\mathrm{x}}\left(\mathrm{sin}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)\sqrt{\mathrm{3}}}{\mathrm{2}}\right)+\sqrt{\mathrm{3}}\mathrm{cos}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\right) \\ $$$$=\mathrm{2a}\sqrt{\mathrm{x}}\left(\mathrm{sin}\left(\frac{\mathrm{ln}\left(\mathrm{x}\right)\sqrt{\mathrm{3}}}{\mathrm{2}}+\frac{\pi}{\mathrm{3}}\right)\right),\mathrm{a}\in\mathbb{R} \\ $$$$ \\ $$$$ \\ $$
