
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 195538 by SLVR last updated on 04/Aug/23

$${Number}\:{of}\:{distributions}\:{of} \\ $$$${n}\:{different}\:{articles}\:{to}\:{r}\:{different}\:\:{boxes} \\ $$$$\left.{so}\:{as}\:\mathrm{1}\right){empty}\:{box}\:{allowed} \\ $$$$\left.\mathrm{2}\right){empty}\:{box}\:{not}\:{allowed} \\ $$$${with}\:{proof}...{kindly}\:{help}\:{me} \\ $$
Commented by SLVR last updated on 04/Aug/23
$${sir}...{kindly}\:{give}\:{proof} \\ $$
Commented by SLVR last updated on 05/Aug/23
$${sir}\:{Mr}.{W}..{or}...{any}\:{one}\:{can} \\ $$$${help}\:{me}...{i}\:{need}\:{bit}\:{urgently} \\ $$
Commented by mr W last updated on 05/Aug/23
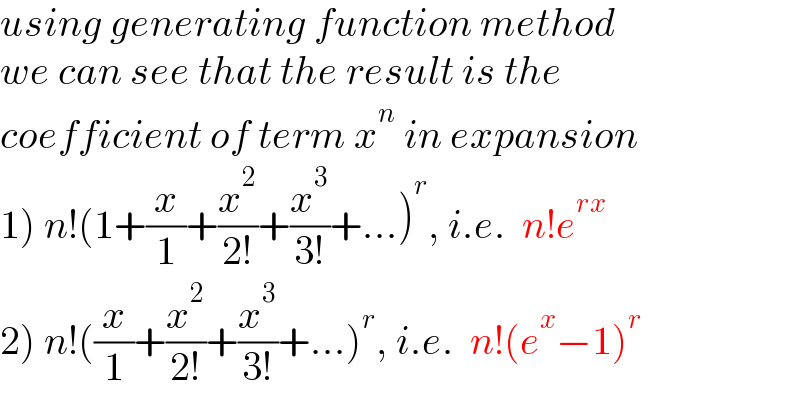
$${using}\:{generating}\:{function}\:{method} \\ $$$${we}\:{can}\:{see}\:{that}\:{the}\:{result}\:{is}\:{the} \\ $$$${coefficient}\:{of}\:{term}\:{x}^{{n}} \:{in}\:{expansion} \\ $$$$\left.\mathrm{1}\right)\:{n}!\left(\mathrm{1}+\frac{{x}}{\mathrm{1}}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+...\right)^{{r}} ,\:{i}.{e}.\:\:{n}!{e}^{{rx}} \\ $$$$\left.\mathrm{2}\right)\:{n}!\left(\frac{{x}}{\mathrm{1}}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+...\right)^{{r}} ,\:{i}.{e}.\:\:{n}!\left({e}^{{x}} −\mathrm{1}\right)^{{r}} \\ $$
Commented by SLVR last updated on 05/Aug/23
$${Thanks}\:{Prof}.{W}...{so}\:{kind}\:{of}\:{you} \\ $$
Commented by mr W last updated on 05/Aug/23

$${a}\:{little}\:{explanation}: \\ $$$${say}\:{box}\:\mathrm{1}\:{has}\:{k}_{\mathrm{1}} \:{objects},\:{box}\:\mathrm{2}\:{has}\:{k}_{\mathrm{2}} \\ $$$${objects},\:{etc}.\:{with}\:{k}_{\mathrm{1}} +{k}_{\mathrm{2}} +...{k}_{{r}} ={n}\:{and} \\ $$$${k}_{\mathrm{1}} ,{k}_{\mathrm{2}} ,...,{k}_{{r}} \:{are}\:{fixed}\:{values}. \\ $$$${to}\:{distribute}\:{n}\:{objects}\:{in}\:{r}\:{boxes}\:{in} \\ $$$${this}\:{way}\:{there}\:{are}\:\frac{{n}!}{\left({k}_{\mathrm{1}} \right)!×\left({k}_{\mathrm{2}} \right)!×...\left({k}_{{r}} \right)!} \\ $$$${possibilities}. \\ $$$${since}\:{k}_{\mathrm{1}} ,{k}_{\mathrm{2}} ,...,{k}_{{r}} \:{can}\:{be}\:{variable}\:{values}, \\ $$$${we}\:{can}\:{construct}\:{a}\:{generating} \\ $$$${function}\:{like} \\ $$$${n}!\left(\frac{{x}}{\mathrm{1}!}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+...+...\right)^{{r}} \:{if}\:{each}\:{box}\:{must} \\ $$$${get}\:{at}\:{least}\:{an}\:{object},\:{or} \\ $$$${n}!\left(\mathrm{1}+\frac{{x}}{\mathrm{1}!}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+...+...\right)^{{r}} \:{if}\:{each}\:{box}\:{may} \\ $$$${also}\:{be}\:{empty}. \\ $$$$ \\ $$$$\left.{for}\:\mathrm{1}\right)\:{the}\:{formula}\:{is}\:{r}^{{n}} . \\ $$$$\left.{for}\:\mathrm{2}\right)\:{the}\:{formula}\:{is}\: \\ $$$$\:\:\:{r}^{{n}} −{C}_{\mathrm{1}} ^{{r}} \left({r}−\mathrm{1}\right)^{{n}} +{C}_{\mathrm{2}} ^{{r}} \left({r}−\mathrm{2}\right)^{{n}} −...+\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} {C}_{{r}−\mathrm{1}} ^{{r}} \\ $$
Commented by mr W last updated on 05/Aug/23

$${example}: \\ $$$${we}\:{have}\:\mathrm{10}\:{foreign}\:{students}\:{who} \\ $$$${should}\:{be}\:{distributed}\:{to}\:\mathrm{3}\:{schools}. \\ $$$${in}\:{how}\:{many}\:{ways}\:{can}\:{this}\:{be}\:{done}? \\ $$$$\left.\mathrm{1}\right)\:\mathrm{3}^{\mathrm{10}} =\mathrm{59049}\:{ways} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{3}^{\mathrm{10}} −\mathrm{3}×\mathrm{2}^{\mathrm{10}} +\mathrm{3}×\mathrm{1}^{\mathrm{10}} =\mathrm{55980}\:{ways} \\ $$
Commented by mr W last updated on 05/Aug/23

Answered by MM42 last updated on 05/Aug/23
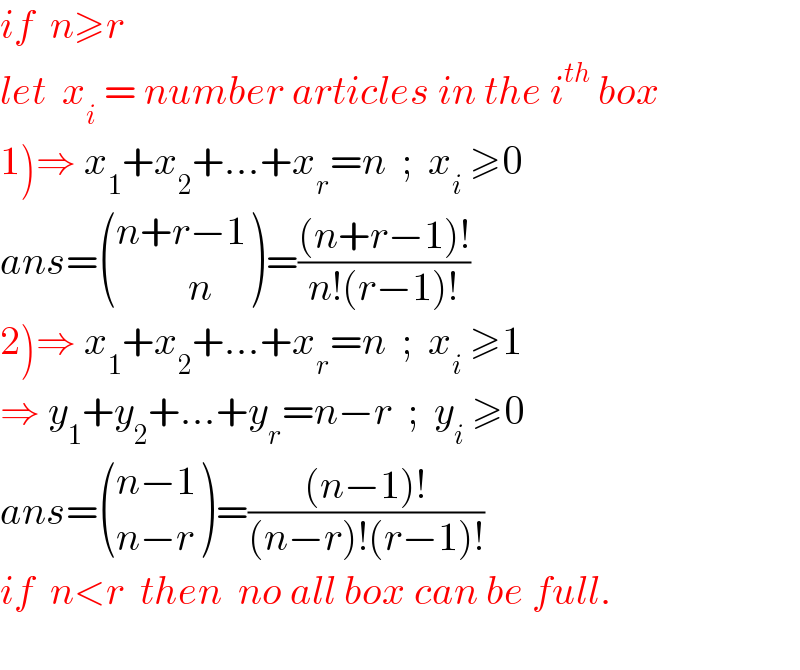
$${if}\:\:{n}\geqslant{r} \\ $$$${let}\:\:{x}_{{i}} \:=\:{number}\:{articles}\:{in}\:{the}\:{i}^{{th}} \:{box} \\ $$$$\left.\mathrm{1}\right)\Rightarrow\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +...+{x}_{{r}} ={n}\:\:;\:\:{x}_{{i}} \:\geqslant\mathrm{0} \\ $$$${ans}=\begin{pmatrix}{{n}+{r}−\mathrm{1}}\\{\:\:\:\:\:\:\:\:\:{n}}\end{pmatrix}=\frac{\left({n}+{r}−\mathrm{1}\right)!}{{n}!\left({r}−\mathrm{1}\right)!} \\ $$$$\left.\mathrm{2}\right)\Rightarrow\:{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +...+{x}_{{r}} ={n}\:\:;\:\:{x}_{{i}} \:\geqslant\mathrm{1} \\ $$$$\Rightarrow\:{y}_{\mathrm{1}} +{y}_{\mathrm{2}} +...+{y}_{{r}} ={n}−{r}\:\:;\:\:{y}_{{i}} \:\geqslant\mathrm{0} \\ $$$${ans}=\begin{pmatrix}{{n}−\mathrm{1}}\\{{n}−{r}}\end{pmatrix}=\frac{\left({n}−\mathrm{1}\right)!}{\left({n}−{r}\right)!\left({r}−\mathrm{1}\right)!} \\ $$$${if}\:\:{n}<{r}\:\:{then}\:\:{no}\:{all}\:{box}\:{can}\:{be}\:{full}. \\ $$$$ \\ $$
Commented by mr W last updated on 05/Aug/23

$${what}\:{you}\:{solved}\:{is}\:{for}\:{the}\:{case}\:{that} \\ $$$${the}\:{objects}\:{are}\:{identical}.\:{but}\:{the} \\ $$$${question}\:{said}\:{that}\:{both}\:{the}\:{boxes} \\ $$$${and}\:{the}\:{objects}\:{are}\:{different}. \\ $$
Commented by MM42 last updated on 08/Aug/23
$${yes}.{you}\:{are}\:{right}.{i}\:{did}\:{not}\:{notice} \\ $$$${the}\:{diffrence}\:{between}\:{the}\:{subjects}. \\ $$$${thanks}\:{for}\:{you}\: \\ $$$$ \\ $$
