
Question Number 195443 by MM42 last updated on 02/Aug/23
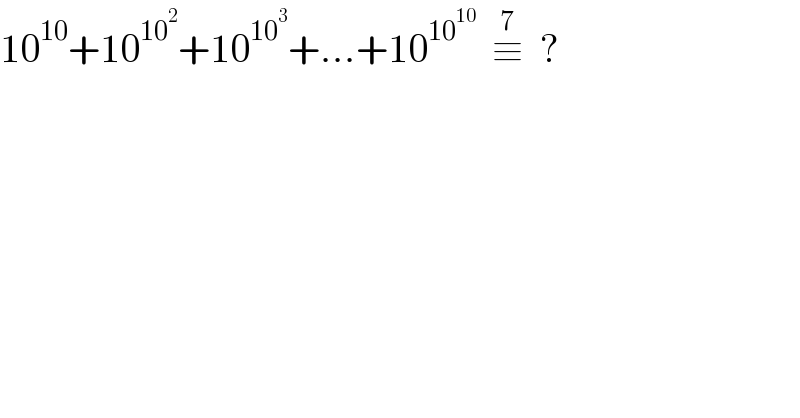
$$\mathrm{10}^{\mathrm{10}} +\mathrm{10}^{\mathrm{10}^{\mathrm{2}} } +\mathrm{10}^{\mathrm{10}^{\mathrm{3}} } +...+\mathrm{10}^{\mathrm{10}^{\mathrm{10}} } \:\:\overset{\mathrm{7}} {\equiv}\:\:? \\ $$
Answered by BaliramKumar last updated on 02/Aug/23

$$\mathrm{5} \\ $$
Commented by MM42 last updated on 02/Aug/23

$${ok} \\ $$
Answered by BaliramKumar last updated on 02/Aug/23
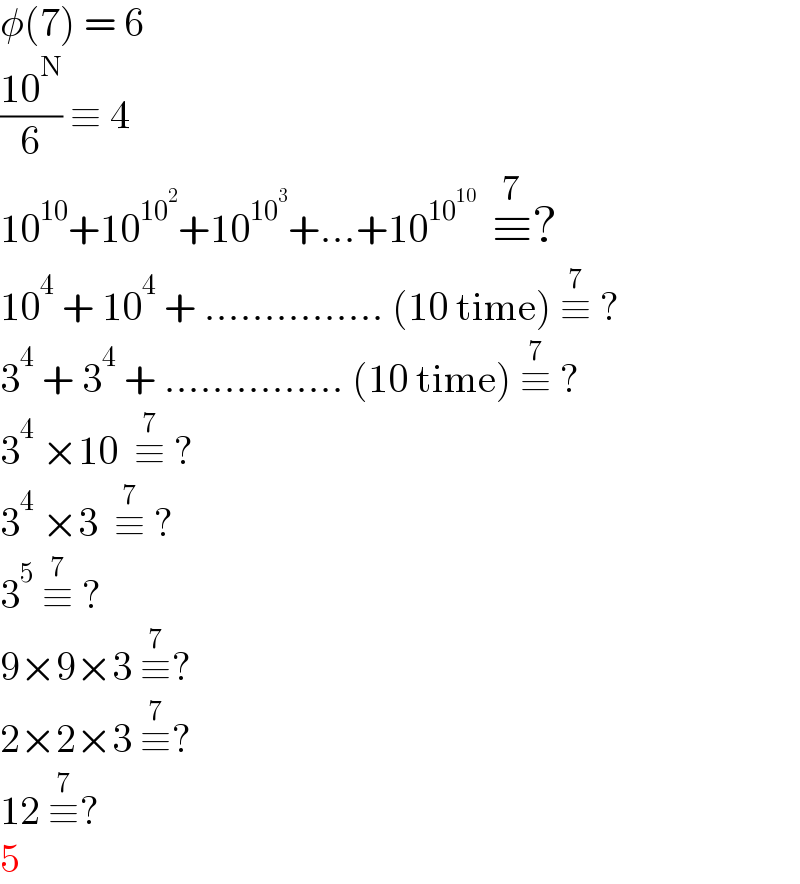
$$\phi\left(\mathrm{7}\right)\:=\:\mathrm{6} \\ $$$$\frac{\mathrm{10}^{\mathrm{N}} }{\mathrm{6}}\:\equiv\:\mathrm{4} \\ $$$$\mathrm{10}^{\mathrm{10}} +\mathrm{10}^{\mathrm{10}^{\mathrm{2}} } +\mathrm{10}^{\mathrm{10}^{\mathrm{3}} } +...+\mathrm{10}^{\mathrm{10}^{\mathrm{10}} } \:\:\overset{\mathrm{7}} {\equiv}? \\ $$$$\mathrm{10}^{\mathrm{4}} \:+\:\mathrm{10}^{\mathrm{4}} \:+\:...............\:\left(\mathrm{10}\:\mathrm{time}\right)\:\overset{\mathrm{7}} {\equiv}\:? \\ $$$$\mathrm{3}^{\mathrm{4}} \:+\:\mathrm{3}^{\mathrm{4}} \:+\:...............\:\left(\mathrm{10}\:\mathrm{time}\right)\:\overset{\mathrm{7}} {\equiv}\:? \\ $$$$\mathrm{3}^{\mathrm{4}} \:×\mathrm{10}\:\:\overset{\mathrm{7}} {\equiv}\:? \\ $$$$\mathrm{3}^{\mathrm{4}} \:×\mathrm{3}\:\:\overset{\mathrm{7}} {\equiv}\:? \\ $$$$\mathrm{3}^{\mathrm{5}} \:\overset{\mathrm{7}} {\equiv}\:? \\ $$$$\mathrm{9}×\mathrm{9}×\mathrm{3}\:\overset{\mathrm{7}} {\equiv}? \\ $$$$\mathrm{2}×\mathrm{2}×\mathrm{3}\:\overset{\mathrm{7}} {\equiv}? \\ $$$$\mathrm{12}\:\overset{\mathrm{7}} {\equiv}? \\ $$$$\mathrm{5} \\ $$
Commented by MM42 last updated on 03/Aug/23

$${your}\:{solution}\:{was}\:{beautiful} \\ $$$${another}\:{solution} \\ $$$$\mathrm{10}^{{n}} =\mathrm{3}{k}+\mathrm{1}\:\:;\:``{k}''\:{is}\:{odd}. \\ $$$$\mathrm{10}\underset{\mathrm{7}} {\equiv}\mathrm{3} \\ $$$$\mathrm{10}^{\mathrm{10}} +\mathrm{10}^{\mathrm{10}^{\mathrm{2}} } +\mathrm{10}^{\mathrm{10}^{\mathrm{3}} } +...+\mathrm{10}^{\mathrm{10}^{\mathrm{10}} } \\ $$$$\underset{\mathrm{7}} {\equiv}\mathrm{3}^{\mathrm{10}} +\mathrm{3}^{\mathrm{10}^{\mathrm{2}} } +\mathrm{3}^{\mathrm{10}^{\mathrm{3}} } +...+\mathrm{3}^{\mathrm{10}^{\mathrm{10}} } \\ $$$$=\mathrm{3}^{\mathrm{3}{k}_{\mathrm{1}} +\mathrm{1}} +\mathrm{3}^{\mathrm{3}{k}_{\mathrm{2}} +\mathrm{1}} +\mathrm{3}^{\mathrm{3}{k}_{\mathrm{3}} +\mathrm{1}} +...+\mathrm{3}^{\mathrm{3}{k}_{\mathrm{10}} +\mathrm{1}} \:\:\:\:;\:``{k}_{{i}} ''\:\:{is}\:\:{odd} \\ $$$$=\mathrm{3}×\left(\mathrm{27}\right)^{{k}_{\mathrm{1}} } +\mathrm{3}×\left(\mathrm{27}\right)^{{k}_{\mathrm{2}} } +\mathrm{3}×\left(\mathrm{27}\right)^{{k}_{\mathrm{3}} } +...+\mathrm{3}×\left(\mathrm{27}\right)^{{k}_{\mathrm{10}} } \\ $$$$\underset{\mathrm{7}} {\equiv}−\mathrm{3}−\mathrm{3}−\mathrm{3}−...−\mathrm{3}=−\mathrm{30}\underset{\mathrm{7}} {\equiv}\:\mathrm{5} \\ $$$$\: \\ $$
