
Question Number 195342 by Matica last updated on 31/Jul/23

$$\:\:\mathrm{1}.\:\mathrm{Prove}\:\mathrm{that}\:\:\forall{n}\:\in\:\mathbb{N}^{\ast} \:,\:\mathrm{4}^{{n}} \left({n}!\right)^{\mathrm{3}} \:<\:\left({n}+\mathrm{1}\right)^{\mathrm{3}{n}} \:. \\ $$$$\mathrm{2}.\:\mathrm{Solve}\:\mathrm{the}\:\mathrm{equations}\:\mathrm{in}\:\mathbb{Z}^{\mathrm{2}} \:: \\ $$$$\:\:\:\:\:{a}./\:\:\mathrm{2}{x}^{\mathrm{3}} +{xy}−\mathrm{7}=\mathrm{0}\:, \\ $$$$\:\:\:\:\:{b}./\:\:{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)={y}^{\mathrm{2}} . \\ $$
Answered by Rasheed.Sindhi last updated on 31/Jul/23

$$\:\:\:\:\:{a}./\:\:\mathrm{2}{x}^{\mathrm{3}} +{xy}−\mathrm{7}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:{x}\left(\mathrm{2}{x}^{\mathrm{2}} +{y}\right)=\mathrm{7} \\ $$$$\begin{cases}{{x}=\mathrm{1}\:\wedge\:\mathrm{2}{x}^{\mathrm{2}} +{y}=\mathrm{7}\Rightarrow{y}=\mathrm{5}\:}\\{{x}=−\mathrm{1}\:\wedge\:\mathrm{2}{x}^{\mathrm{2}} +{y}=−\mathrm{7}\Rightarrow{y}=−\mathrm{9}\:\:}\\{{x}=\mathrm{7}\:\wedge\:\mathrm{2}{x}^{\mathrm{2}} +{y}=\mathrm{1}\Rightarrow{y}=−\mathrm{97}\:}\\{{x}=−\mathrm{7}\:\wedge\:\mathrm{2}{x}^{\mathrm{2}} +{y}=−\mathrm{1}\Rightarrow{y}=−\mathrm{99}\:}\end{cases} \\ $$
Commented by Matica last updated on 31/Jul/23

$${Thank}\:{you}.\:{So}\:{the}\:{trick}\:{is}\:``\:{don}'{t}\:{forget}\: \\ $$$${x},\:{y}\:\in\:\mathbb{Z}. \\ $$
Answered by Rasheed.Sindhi last updated on 31/Jul/23

$$\:\:\:\:\:{b}./\:\:{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)={y}^{\mathrm{2}} \\ $$$${SOME}\:{cases}: \\ $$$$\bullet{x}\left({x}+\mathrm{1}\right)=\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right) \\ $$$$\:\:\:{x}^{\mathrm{2}} +{x}={x}^{\mathrm{2}} +\mathrm{15}{x}+\mathrm{56} \\ $$$$\:\:\:\:\mathrm{14}{x}+\mathrm{56}=\mathrm{0}\Rightarrow{x}=−\mathrm{4}\checkmark \\ $$$$\bullet{x}\left({x}+\mathrm{7}\right)=\left({x}+\mathrm{1}\right)\left({x}+\mathrm{8}\right) \\ $$$$\:\:\:{x}^{\mathrm{2}} +\mathrm{7}{x}={x}^{\mathrm{2}} +\mathrm{9}{x}+\mathrm{8} \\ $$$$\:\:\:\:\mathrm{2}{x}+\mathrm{8}=\mathrm{0}\Rightarrow{x}=−\mathrm{4}\checkmark \\ $$$$\bullet{x}\left({x}+\mathrm{8}\right)=\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right) \\ $$$$\:\:\:{x}^{\mathrm{2}} +\mathrm{8}{x}={x}^{\mathrm{2}} +\mathrm{8}{x}+\mathrm{7}\:\left({No}\:{result}\right) \\ $$$$\bullet{x}={x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)\:\left({x}\notin\mathbb{Z}\right) \\ $$$$\bullet{x}+\mathrm{1}={x}\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)\:\:\:\:\left({x}\notin\mathbb{Z}\right) \\ $$$$\bullet{x}+\mathrm{7}={x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{8}\right)\:\:\:\left({x}\notin\mathbb{Z}\right) \\ $$$$\bullet{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)=\mathrm{0} \\ $$$$\:\:{x}=\mathrm{0},−\mathrm{1},−\mathrm{7},−\mathrm{8}\:\checkmark \\ $$$$\bullet{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)=\mathrm{144} \\ $$$$\:\:\:{x}=\mathrm{1},−\mathrm{4},−\mathrm{9}\:\checkmark \\ $$
Commented by Matica last updated on 01/Aug/23

$${Thank}\:{you}. \\ $$
Answered by witcher3 last updated on 31/Jul/23
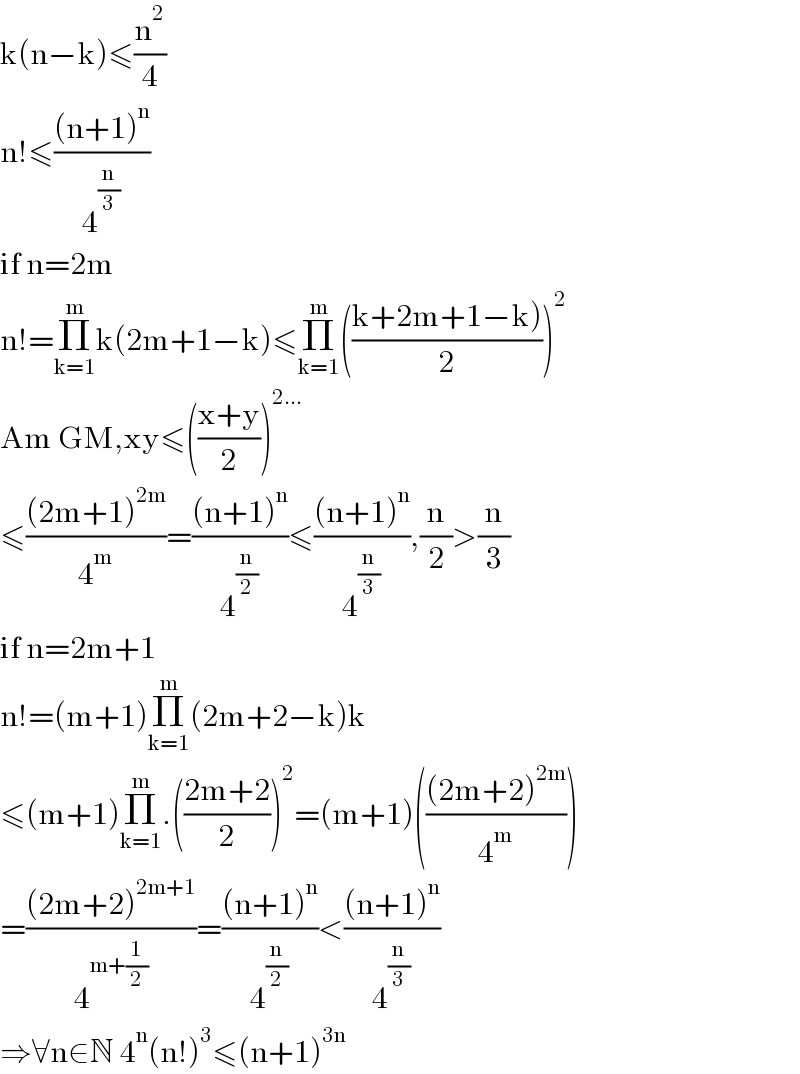
$$\mathrm{k}\left(\mathrm{n}−\mathrm{k}\right)\leqslant\frac{\mathrm{n}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\mathrm{n}!\leqslant\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\frac{\mathrm{n}}{\mathrm{3}}} } \\ $$$$\mathrm{if}\:\mathrm{n}=\mathrm{2m} \\ $$$$\mathrm{n}!=\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{m}} {\prod}}\mathrm{k}\left(\mathrm{2m}+\mathrm{1}−\mathrm{k}\right)\leqslant\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{m}} {\prod}}\left(\frac{\left.\mathrm{k}+\mathrm{2m}+\mathrm{1}−\mathrm{k}\right)}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$$$\mathrm{Am}\:\mathrm{GM},\mathrm{xy}\leqslant\left(\frac{\mathrm{x}+\mathrm{y}}{\mathrm{2}}\right)^{\mathrm{2}...} \\ $$$$\leqslant\frac{\left(\mathrm{2m}+\mathrm{1}\right)^{\mathrm{2m}} }{\mathrm{4}^{\mathrm{m}} }=\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\frac{\mathrm{n}}{\mathrm{2}}} }\leqslant\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\frac{\mathrm{n}}{\mathrm{3}}} },\frac{\mathrm{n}}{\mathrm{2}}>\frac{\mathrm{n}}{\mathrm{3}} \\ $$$$\mathrm{if}\:\mathrm{n}=\mathrm{2m}+\mathrm{1} \\ $$$$\mathrm{n}!=\left(\mathrm{m}+\mathrm{1}\right)\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{m}} {\prod}}\left(\mathrm{2m}+\mathrm{2}−\mathrm{k}\right)\mathrm{k} \\ $$$$\leqslant\left(\mathrm{m}+\mathrm{1}\right)\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{m}} {\prod}}.\left(\frac{\mathrm{2m}+\mathrm{2}}{\mathrm{2}}\right)^{\mathrm{2}} =\left(\mathrm{m}+\mathrm{1}\right)\left(\frac{\left(\mathrm{2m}+\mathrm{2}\right)^{\mathrm{2m}} }{\mathrm{4}^{\mathrm{m}} }\right) \\ $$$$=\frac{\left(\mathrm{2m}+\mathrm{2}\right)^{\mathrm{2m}+\mathrm{1}} }{\mathrm{4}^{\mathrm{m}+\frac{\mathrm{1}}{\mathrm{2}}} }=\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\frac{\mathrm{n}}{\mathrm{2}}} }<\frac{\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{4}^{\frac{\mathrm{n}}{\mathrm{3}}} } \\ $$$$\Rightarrow\forall\mathrm{n}\in\mathbb{N}\:\mathrm{4}^{\mathrm{n}} \left(\mathrm{n}!\right)^{\mathrm{3}} \leqslant\left(\mathrm{n}+\mathrm{1}\right)^{\mathrm{3n}} \: \\ $$
Commented by Matica last updated on 01/Aug/23
$${Thank}\:{you}\:{brother}. \\ $$
Commented by witcher3 last updated on 01/Aug/23

$$\mathrm{withe}\:\mathrm{Pleasur}\:\mathrm{god}\:\mathrm{bless}\:\mathrm{You} \\ $$
Answered by Rasheed.Sindhi last updated on 31/Jul/23

$$\:\:\:\:\:{b}./\:\:{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{7}\right)\left({x}+\mathrm{8}\right)={y}^{\mathrm{2}} \\ $$$${Let}\:{x}+\mathrm{4}={a} \\ $$$$\Rightarrow\left({a}−\mathrm{4}\right)\left({a}−\mathrm{3}\right)\left({a}+\mathrm{3}\right)\left({a}+\mathrm{4}\right)={y}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\left({a}^{\mathrm{2}} −\mathrm{16}\right)\left({a}^{\mathrm{2}} −\mathrm{9}\right)={y}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:{a}^{\mathrm{4}} −\mathrm{25}{a}^{\mathrm{2}} +\mathrm{144}={y}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:{a}^{\mathrm{4}} −\mathrm{25}{a}^{\mathrm{2}} +\mathrm{144}−{y}^{\mathrm{2}} =\mathrm{0} \\ $$$${a}^{\mathrm{2}} =\frac{\mathrm{25}\pm\sqrt{\mathrm{625}−\mathrm{4}\left(\mathrm{144}−{y}^{\mathrm{2}} \right)}}{\mathrm{2}} \\ $$$$\:\:\:\:=\frac{\mathrm{25}\pm\sqrt{\mathrm{49}+\mathrm{4}{y}^{\mathrm{2}} }}{\mathrm{2}}\in\mathbb{Z}^{+} \\ $$$$\:\:\:\:\:\:\sqrt{\mathrm{49}+\mathrm{4}{y}^{\mathrm{2}} }\:\leqslant\mathrm{25}\:\wedge\:\mathrm{4}{y}^{\mathrm{2}} +\mathrm{49}\:{is}\:{perfect}\:{square}. \\ $$$$\:\:\:\:\mathrm{4}{y}^{\mathrm{2}} +\mathrm{49}\leqslant\mathrm{625} \\ $$$$\:{y}^{\mathrm{2}} \leqslant\mathrm{144}\Rightarrow−\mathrm{12}\leqslant{y}\leqslant\mathrm{12} \\ $$$${The}\:{value}\:{of}\:{y}\:{for}\:{which}\:\mathrm{4}{y}^{\mathrm{2}} +\mathrm{49} \\ $$$${is}\:{perfect}\:{square}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{y}=\mathrm{0},\pm\mathrm{12} \\ $$$${y}=\mathrm{0}: \\ $$$${a}^{\mathrm{2}} =\frac{\mathrm{25}\pm\sqrt{\mathrm{49}+\mathrm{4}\left(\mathrm{0}\right)^{\mathrm{2}} }}{\mathrm{2}}=\mathrm{16},\mathrm{9} \\ $$$${a}=\pm\mathrm{4},\pm\mathrm{3} \\ $$$${x}=\pm\mathrm{4}−\mathrm{4},\pm\mathrm{3}−\mathrm{4} \\ $$$$\:\:=\mathrm{0},−\mathrm{8},−\mathrm{1},−\mathrm{7}\:\checkmark \\ $$$$\: \\ $$$${y}=\pm\mathrm{12}: \\ $$$${a}^{\mathrm{2}} =\frac{\mathrm{25}\pm\sqrt{\mathrm{49}+\mathrm{4}\left(\pm\mathrm{12}\right)^{\mathrm{2}} }}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:=\frac{\mathrm{25}\pm\mathrm{25}}{\mathrm{2}}=\mathrm{25},\mathrm{0} \\ $$$${a}=\pm\mathrm{5},\mathrm{0} \\ $$$${x}=\pm\mathrm{5}−\mathrm{4},\mathrm{0}−\mathrm{4} \\ $$$${x}=\mathrm{1},−\mathrm{9},−\mathrm{4}\:\checkmark \\ $$$$\: \\ $$$$\left({x},{y}\right)=\left(\mathrm{0},\mathrm{0}\right),\left(−\mathrm{1},\mathrm{0}\right),\left(−\mathrm{7},\mathrm{0}\right),\left(−\mathrm{8},\mathrm{0}\right), \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{1},\pm\mathrm{12}\right),\left(−\mathrm{4},\pm\mathrm{12}\right),\left(−\mathrm{9},\pm\mathrm{12}\right) \\ $$$$\:\left({Total}\:\mathrm{10}\:{solutions}\right) \\ $$
Commented by Matica last updated on 01/Aug/23

$${Tahnk}\:{you}\:{a}\:{lot}. \\ $$
