
Question Number 194767 by horsebrand11 last updated on 15/Jul/23
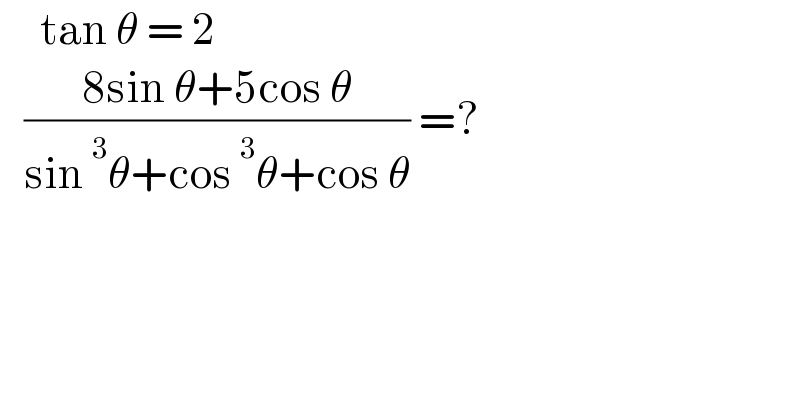
$$\:\:\:\:\:\mathrm{tan}\:\theta\:=\:\mathrm{2}\: \\ $$$$\:\:\:\frac{\mathrm{8sin}\:\theta+\mathrm{5cos}\:\theta}{\mathrm{sin}\:^{\mathrm{3}} \theta+\mathrm{cos}\:^{\mathrm{3}} \theta+\mathrm{cos}\:\theta}\:=? \\ $$
Answered by dimentri last updated on 15/Jul/23

$$\:\:\:\mathrm{sec}\:^{\mathrm{2}} {x}=\mathrm{5}\:,\:\mathrm{tan}\:^{\mathrm{2}} {x}=\mathrm{4} \\ $$$$\:\:\equiv\:\frac{\mathrm{8tan}\:{x}\:\mathrm{sec}\:^{\mathrm{2}} {x}+\mathrm{5sec}\:^{\mathrm{2}} {x}}{\mathrm{tan}\:^{\mathrm{3}} {x}+\mathrm{1}+\mathrm{sec}^{\mathrm{2}} \:{x}} \\ $$$$\:\:=\:\frac{\mathrm{105}}{\mathrm{14}}=\frac{\mathrm{15}}{\mathrm{2}} \\ $$
Answered by Frix last updated on 15/Jul/23
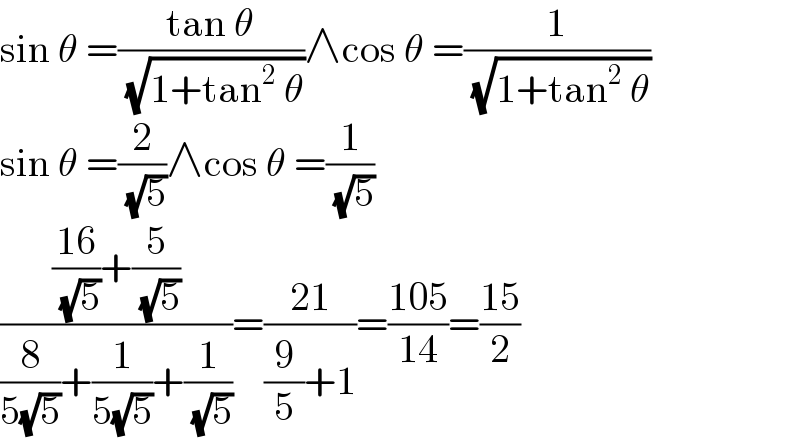
$$\mathrm{sin}\:\theta\:=\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta}}\wedge\mathrm{cos}\:\theta\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta}} \\ $$$$\mathrm{sin}\:\theta\:=\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}\wedge\mathrm{cos}\:\theta\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}} \\ $$$$\frac{\frac{\mathrm{16}}{\:\sqrt{\mathrm{5}}}+\frac{\mathrm{5}}{\:\sqrt{\mathrm{5}}}}{\frac{\mathrm{8}}{\mathrm{5}\sqrt{\mathrm{5}}}+\frac{\mathrm{1}}{\mathrm{5}\sqrt{\mathrm{5}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}}=\frac{\mathrm{21}}{\frac{\mathrm{9}}{\mathrm{5}}+\mathrm{1}}=\frac{\mathrm{105}}{\mathrm{14}}=\frac{\mathrm{15}}{\mathrm{2}} \\ $$
