
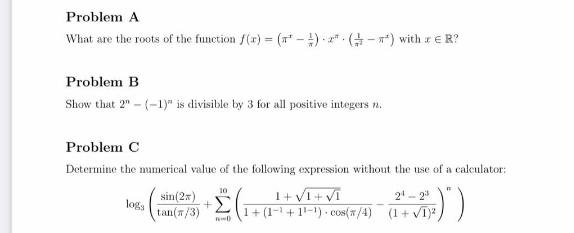
Question Number 194732 by MathsFan last updated on 14/Jul/23
Commented by York12 last updated on 15/Jul/23
$${do}\:{not}\:{participate}\:{IYMC} \\ $$$${it}\:{is}\:{fake} \\ $$
Answered by mahdipoor last updated on 14/Jul/23
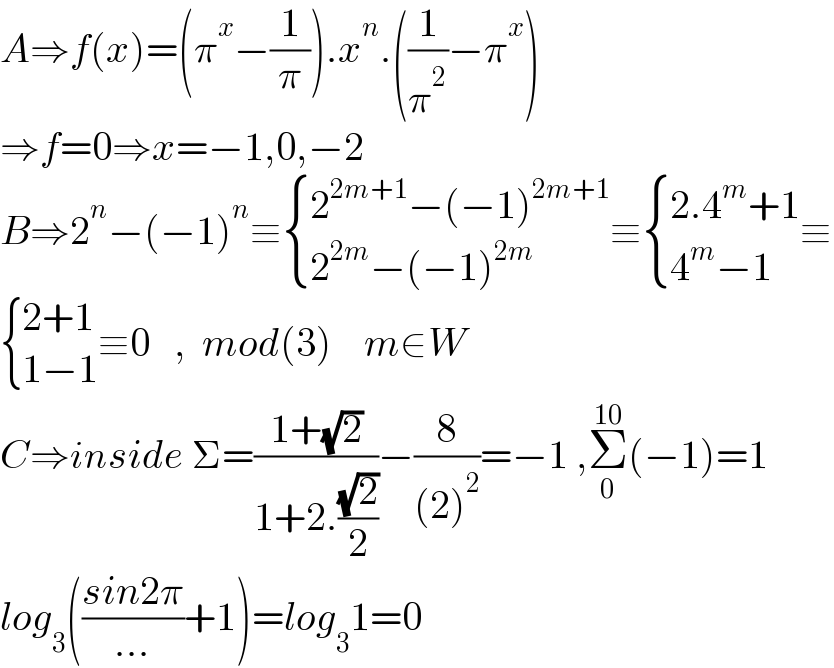
$${A}\Rightarrow{f}\left({x}\right)=\left(\pi^{{x}} −\frac{\mathrm{1}}{\pi}\right).{x}^{{n}} .\left(\frac{\mathrm{1}}{\pi^{\mathrm{2}} }−\pi^{{x}} \right) \\ $$$$\Rightarrow{f}=\mathrm{0}\Rightarrow{x}=−\mathrm{1},\mathrm{0},−\mathrm{2} \\ $$$${B}\Rightarrow\mathrm{2}^{{n}} −\left(−\mathrm{1}\right)^{{n}} \equiv\begin{cases}{\mathrm{2}^{\mathrm{2}{m}+\mathrm{1}} −\left(−\mathrm{1}\right)^{\mathrm{2}{m}+\mathrm{1}} }\\{\mathrm{2}^{\mathrm{2}{m}} −\left(−\mathrm{1}\right)^{\mathrm{2}{m}} }\end{cases}\equiv\begin{cases}{\mathrm{2}.\mathrm{4}^{{m}} +\mathrm{1}}\\{\mathrm{4}^{{m}} −\mathrm{1}}\end{cases}\equiv \\ $$$$\begin{cases}{\mathrm{2}+\mathrm{1}}\\{\mathrm{1}−\mathrm{1}}\end{cases}\equiv\mathrm{0}\:\:\:,\:\:{mod}\left(\mathrm{3}\right)\:\:\:\:{m}\in{W}\:\:\:\: \\ $$$${C}\Rightarrow{inside}\:\Sigma=\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{1}+\mathrm{2}.\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}−\frac{\mathrm{8}}{\left(\mathrm{2}\right)^{\mathrm{2}} }=−\mathrm{1}\:,\underset{\mathrm{0}} {\overset{\mathrm{10}} {\sum}}\left(−\mathrm{1}\right)=\mathrm{1} \\ $$$${log}_{\mathrm{3}} \left(\frac{{sin}\mathrm{2}\pi}{...}+\mathrm{1}\right)={log}_{\mathrm{3}} \mathrm{1}=\mathrm{0} \\ $$
Commented by York12 last updated on 15/Jul/23

$${bro}\:{it}\:{is}\:{an}\:{onlie}\:{exam}\: \\ $$$${called}\:{IYMC}\:\&\:{he}\:{is}\:{cheating} \\ $$
Answered by qaz last updated on 15/Jul/23
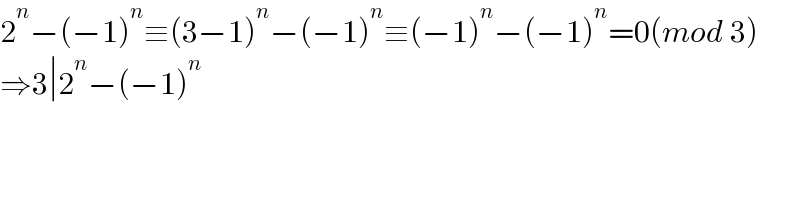
$$\mathrm{2}^{{n}} −\left(−\mathrm{1}\right)^{{n}} \equiv\left(\mathrm{3}−\mathrm{1}\right)^{{n}} −\left(−\mathrm{1}\right)^{{n}} \equiv\left(−\mathrm{1}\right)^{{n}} −\left(−\mathrm{1}\right)^{{n}} =\mathrm{0}\left({mod}\:\mathrm{3}\right) \\ $$$$\Rightarrow\mathrm{3}\mid\mathrm{2}^{{n}} −\left(−\mathrm{1}\right)^{{n}} \\ $$
