Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 121522 by benjo_mathlover last updated on 09/Nov/20

19 sin 2x =37 cos 2x +38 sin^2 x find the value of tan x .

$$\mathrm{19}\:\mathrm{sin}\:\mathrm{2x}\:=\mathrm{37}\:\mathrm{cos}\:\mathrm{2x}\:+\mathrm{38}\:\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}\: \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{tan}\:\mathrm{x}\:. \\ $$

Commented by liberty last updated on 09/Nov/20

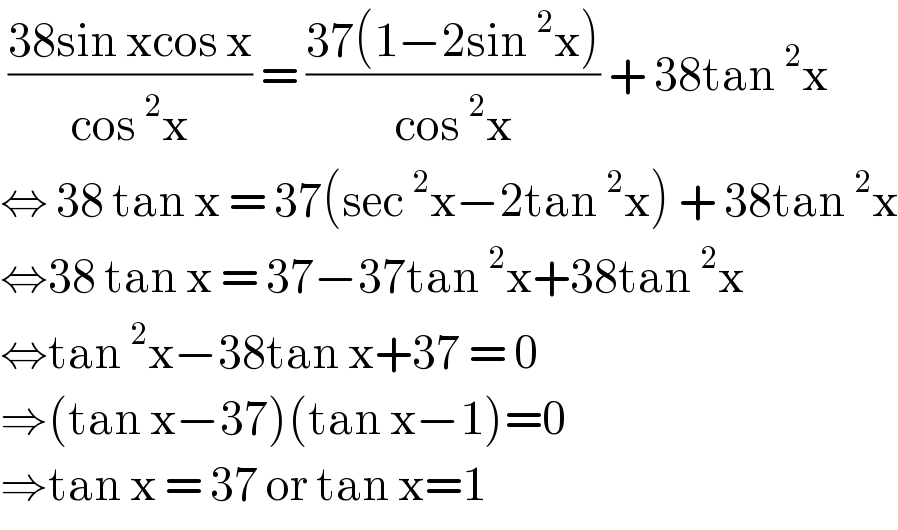
((38sin xcos x)/(cos^2 x)) = ((37(1−2sin^2 x))/(cos^2 x)) + 38tan^2 x ⇔ 38 tan x = 37(sec^2 x−2tan^2 x) + 38tan^2 x ⇔38 tan x = 37−37tan^2 x+38tan^2 x ⇔tan^2 x−38tan x+37 = 0 ⇒(tan x−37)(tan x−1)=0 ⇒tan x = 37 or tan x=1

$$\:\frac{\mathrm{38sin}\:\mathrm{xcos}\:\mathrm{x}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}\:=\:\frac{\mathrm{37}\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{x}\right)}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}\:+\:\mathrm{38tan}\:^{\mathrm{2}} \mathrm{x} \\ $$$$\Leftrightarrow\:\mathrm{38}\:\mathrm{tan}\:\mathrm{x}\:=\:\mathrm{37}\left(\mathrm{sec}\:^{\mathrm{2}} \mathrm{x}−\mathrm{2tan}\:^{\mathrm{2}} \mathrm{x}\right)\:+\:\mathrm{38tan}\:^{\mathrm{2}} \mathrm{x} \\ $$$$\Leftrightarrow\mathrm{38}\:\mathrm{tan}\:\mathrm{x}\:=\:\mathrm{37}−\mathrm{37tan}\:^{\mathrm{2}} \mathrm{x}+\mathrm{38tan}\:^{\mathrm{2}} \mathrm{x}\: \\ $$$$\Leftrightarrow\mathrm{tan}\:^{\mathrm{2}} \mathrm{x}−\mathrm{38tan}\:\mathrm{x}+\mathrm{37}\:=\:\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{tan}\:\mathrm{x}−\mathrm{37}\right)\left(\mathrm{tan}\:\mathrm{x}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{tan}\:\mathrm{x}\:=\:\mathrm{37}\:\mathrm{or}\:\mathrm{tan}\:\mathrm{x}=\mathrm{1} \\ $$

Commented by MJS_new last updated on 09/Nov/20

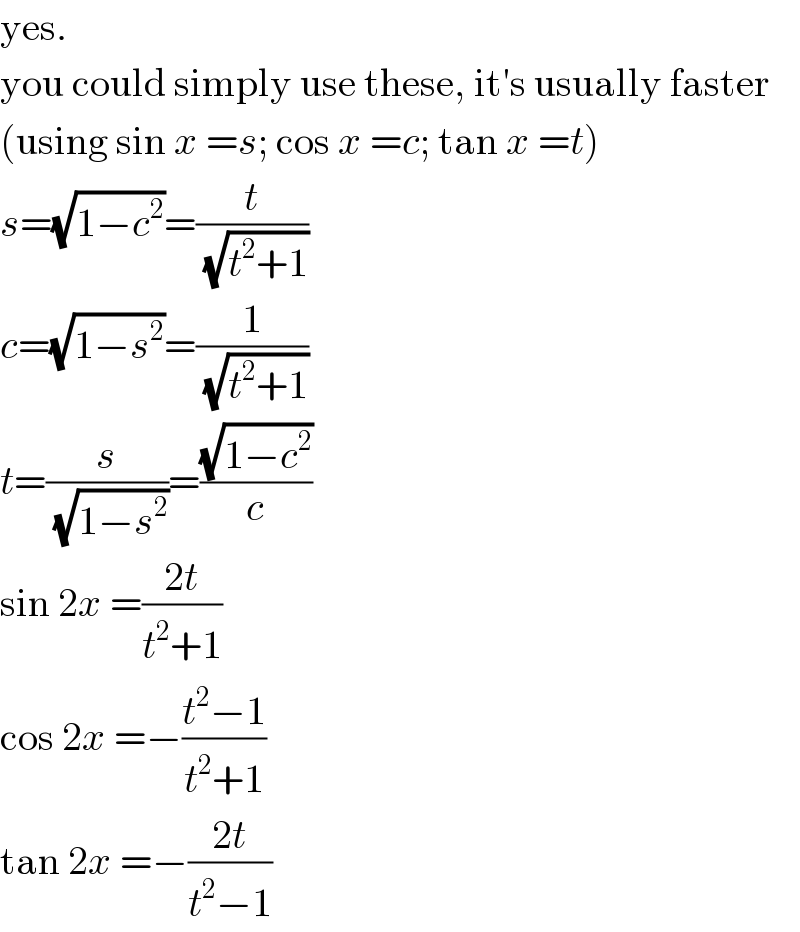
yes. you could simply use these, it′s usually faster (using sin x =s; cos x =c; tan x =t) s=(√(1−c^2 ))=(t/( (√(t^2 +1)))) c=(√(1−s^2 ))=(1/( (√(t^2 +1)))) t=(s/( (√(1−s^2 ))))=((√(1−c^2 ))/c) sin 2x =((2t)/(t^2 +1)) cos 2x =−((t^2 −1)/(t^2 +1)) tan 2x =−((2t)/(t^2 −1))

$$\mathrm{yes}. \\ $$$$\mathrm{you}\:\mathrm{could}\:\mathrm{simply}\:\mathrm{use}\:\mathrm{these},\:\mathrm{it}'\mathrm{s}\:\mathrm{usually}\:\mathrm{faster} \\ $$$$\left(\mathrm{using}\:\mathrm{sin}\:{x}\:={s};\:\mathrm{cos}\:{x}\:={c};\:\mathrm{tan}\:{x}\:={t}\right) \\ $$$${s}=\sqrt{\mathrm{1}−{c}^{\mathrm{2}} }=\frac{{t}}{\:\sqrt{{t}^{\mathrm{2}} +\mathrm{1}}} \\ $$$${c}=\sqrt{\mathrm{1}−{s}^{\mathrm{2}} }=\frac{\mathrm{1}}{\:\sqrt{{t}^{\mathrm{2}} +\mathrm{1}}} \\ $$$${t}=\frac{{s}}{\:\sqrt{\mathrm{1}−{s}^{\mathrm{2}} }}=\frac{\sqrt{\mathrm{1}−{c}^{\mathrm{2}} }}{{c}} \\ $$$$\mathrm{sin}\:\mathrm{2}{x}\:=\frac{\mathrm{2}{t}}{{t}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{cos}\:\mathrm{2}{x}\:=−\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{tan}\:\mathrm{2}{x}\:=−\frac{\mathrm{2}{t}}{{t}^{\mathrm{2}} −\mathrm{1}} \\ $$

Commented by benjo_mathlover last updated on 09/Nov/20

thank you

$$\mathrm{thank}\:\mathrm{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com