
Question Number 18891 by Tinkutara last updated on 01/Aug/17
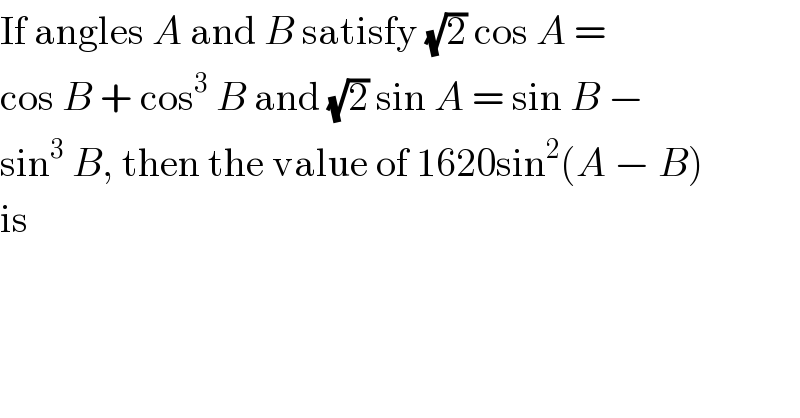
$$\mathrm{If}\:\mathrm{angles}\:{A}\:\mathrm{and}\:{B}\:\mathrm{satisfy}\:\sqrt{\mathrm{2}}\:\mathrm{cos}\:{A}\:= \\ $$$$\mathrm{cos}\:{B}\:+\:\mathrm{cos}^{\mathrm{3}} \:{B}\:\mathrm{and}\:\sqrt{\mathrm{2}}\:\mathrm{sin}\:{A}\:=\:\mathrm{sin}\:{B}\:− \\ $$$$\mathrm{sin}^{\mathrm{3}} \:{B},\:\mathrm{then}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{1620sin}^{\mathrm{2}} \left({A}\:−\:{B}\right) \\ $$$$\mathrm{is} \\ $$
Answered by behi.8.3.4.1.7@gmail.com last updated on 02/Aug/17
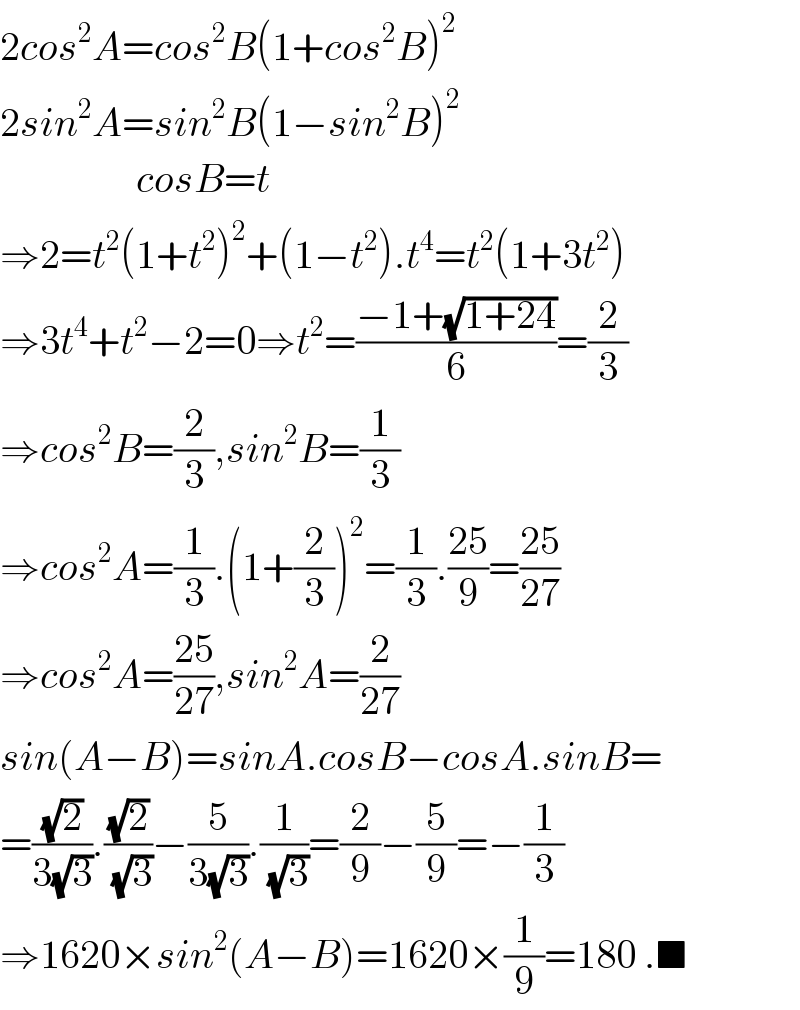
$$\mathrm{2}{cos}^{\mathrm{2}} {A}={cos}^{\mathrm{2}} {B}\left(\mathrm{1}+{cos}^{\mathrm{2}} {B}\right)^{\mathrm{2}} \\ $$$$\mathrm{2}{sin}^{\mathrm{2}} {A}={sin}^{\mathrm{2}} {B}\left(\mathrm{1}−{sin}^{\mathrm{2}} {B}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{cosB}={t} \\ $$$$\Rightarrow\mathrm{2}={t}^{\mathrm{2}} \left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} +\left(\mathrm{1}−{t}^{\mathrm{2}} \right).{t}^{\mathrm{4}} ={t}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{3}{t}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\mathrm{3}{t}^{\mathrm{4}} +{t}^{\mathrm{2}} −\mathrm{2}=\mathrm{0}\Rightarrow{t}^{\mathrm{2}} =\frac{−\mathrm{1}+\sqrt{\mathrm{1}+\mathrm{24}}}{\mathrm{6}}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\Rightarrow{cos}^{\mathrm{2}} {B}=\frac{\mathrm{2}}{\mathrm{3}},{sin}^{\mathrm{2}} {B}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\Rightarrow{cos}^{\mathrm{2}} {A}=\frac{\mathrm{1}}{\mathrm{3}}.\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{3}}.\frac{\mathrm{25}}{\mathrm{9}}=\frac{\mathrm{25}}{\mathrm{27}} \\ $$$$\Rightarrow{cos}^{\mathrm{2}} {A}=\frac{\mathrm{25}}{\mathrm{27}},{sin}^{\mathrm{2}} {A}=\frac{\mathrm{2}}{\mathrm{27}} \\ $$$${sin}\left({A}−{B}\right)={sinA}.{cosB}−{cosA}.{sinB}= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}\sqrt{\mathrm{3}}}.\frac{\sqrt{\mathrm{2}}}{\sqrt{\mathrm{3}}}−\frac{\mathrm{5}}{\mathrm{3}\sqrt{\mathrm{3}}}.\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}=\frac{\mathrm{2}}{\mathrm{9}}−\frac{\mathrm{5}}{\mathrm{9}}=−\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{1620}×{sin}^{\mathrm{2}} \left({A}−{B}\right)=\mathrm{1620}×\frac{\mathrm{1}}{\mathrm{9}}=\mathrm{180}\:.\blacksquare \\ $$
Answered by ajfour last updated on 01/Aug/17

$$\sqrt{\mathrm{2}}\mathrm{sin}\:\left(\mathrm{A}−\mathrm{B}\right)=\sqrt{\mathrm{2}}\mathrm{sin}\:\mathrm{Acos}\:\mathrm{B}−\sqrt{\mathrm{2}}\mathrm{cos}\:\mathrm{Asin}\:\mathrm{B} \\ $$$$\:\:=\left(\mathrm{sin}\:\mathrm{B}−\mathrm{sin}\:^{\mathrm{3}} \mathrm{B}\right)\mathrm{cos}\:\mathrm{B} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\left(\mathrm{cos}\:\mathrm{B}+\mathrm{cos}\:^{\mathrm{3}} \mathrm{B}\right)\mathrm{sin}\:\mathrm{B} \\ $$$$\:\:=−\left(\mathrm{sin}\:\mathrm{Bcos}\:\mathrm{B}\right)\left(\mathrm{1}\right) \\ $$$$\Rightarrow\mathrm{2sin}\:^{\mathrm{2}} \left(\mathrm{A}−\mathrm{B}\right)=\mathrm{sin}\:^{\mathrm{2}} \mathrm{Bcos}\:^{\mathrm{2}} \mathrm{B}\:\:..\left(\mathrm{i}\right) \\ $$$$\:\:\:\mathrm{As}\:\:\sqrt{\mathrm{2}}\mathrm{cos}\:\mathrm{A}=\mathrm{cos}\:\mathrm{B}\left(\mathrm{1}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}\right) \\ $$$$\mathrm{and}\:\:\:\sqrt{\mathrm{2}}\mathrm{sin}\:\mathrm{A}=\mathrm{sin}\:\mathrm{B}\left(\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{B}\right) \\ $$$$\mathrm{squaring}\:\mathrm{and}\:\mathrm{adding}\:\mathrm{above}\:\mathrm{two}\:\mathrm{eqns}: \\ $$$$\:\:\mathrm{2}=\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}\left(\mathrm{1}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}\right)^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}\right)\left(\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}\right)^{\mathrm{2}} \\ $$$$\:\:\mathrm{2}=\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}+\mathrm{2cos}\:^{\mathrm{4}} \mathrm{B}+\mathrm{cos}\:^{\mathrm{6}} \mathrm{B} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{cos}\:^{\mathrm{4}} \mathrm{B}−\mathrm{cos}\:^{\mathrm{6}} \mathrm{B} \\ $$$$\Rightarrow\:\:\:\mathrm{3cos}\:^{\mathrm{4}} \mathrm{B}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}−\mathrm{2}=\mathrm{0} \\ $$$$\:\mathrm{3cos}\:^{\mathrm{4}} \mathrm{B}+\mathrm{3cos}\:^{\mathrm{2}} \mathrm{B}−\mathrm{2cos}\:^{\mathrm{2}} \mathrm{B}−\mathrm{2}=\mathrm{0} \\ $$$$\Rightarrow\:\:\left(\mathrm{3cos}\:^{\mathrm{2}} \mathrm{B}−\mathrm{2}\right)\left(\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\Rightarrow\:\:\:\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{B}=\frac{\mathrm{2}}{\mathrm{3}}\:\:\:\mathrm{and}\:\:\:\mathrm{sin}\:^{\mathrm{2}} \mathrm{B}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\mathrm{Using}\:\mathrm{in}\:\left(\mathrm{i}\right): \\ $$$$\:\:\:\:\mathrm{2sin}\:^{\mathrm{2}} \left(\mathrm{A}−\mathrm{B}\right)=\mathrm{sin}\:^{\mathrm{2}} \mathrm{Bcos}\:^{\mathrm{2}} \mathrm{B} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{3}}×\frac{\mathrm{2}}{\mathrm{3}}\:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\:\:\mathrm{So}\:\:\mathrm{1620sin}\:^{\mathrm{2}} \left(\mathrm{A}−\mathrm{B}\right)=\mathrm{810}×\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{1620sin}\:^{\mathrm{2}} \left(\mathrm{A}−\mathrm{B}\right)=\mathrm{180}\:. \\ $$
Commented by behi.8.3.4.1.7@gmail.com last updated on 02/Aug/17
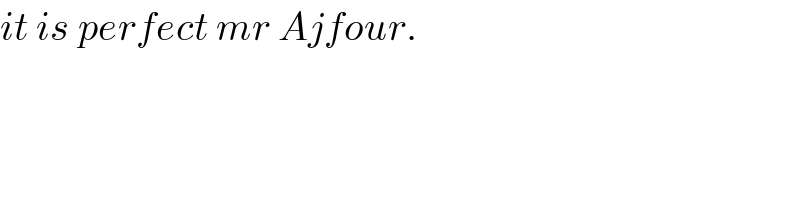
$${it}\:{is}\:{perfect}\:{mr}\:{Ajfour}. \\ $$
Commented by ajfour last updated on 01/Aug/17

$$\mathrm{Is}\:\mathrm{this}\:\mathrm{correct}? \\ $$
Commented by Tinkutara last updated on 01/Aug/17

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{ajfour}\:\mathrm{Sir}! \\ $$
Commented by ajfour last updated on 01/Aug/17

$$\mathrm{thanks}\:\mathrm{for}\:\mathrm{confirming}. \\ $$
