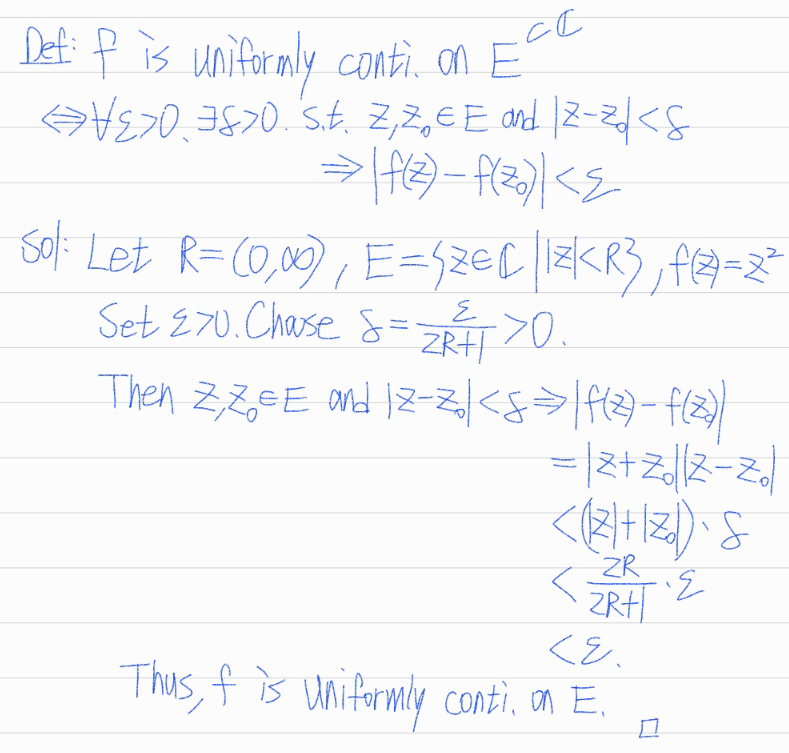Question Number 185245 by Mastermind last updated on 19/Jan/23
$$\mathrm{Show}\:\mathrm{that}\:\mathrm{f}\left(\mathrm{z}\right)=\mathrm{z}^{\mathrm{2}} \:\mathrm{is}\:\mathrm{uniformly} \\ $$ $$\mathrm{continous}\:\mathrm{in}\:\mathrm{the}\:\mathrm{region}\:\mid\mathrm{z}\mid<\mathrm{R} \\ $$ $$\mathrm{where}\:\mathrm{0}<\mathrm{R}<\infty. \\ $$ $$ \\ $$ $$ \\ $$ $$\mathrm{Help}! \\ $$
Commented byFrix last updated on 19/Jan/23

$$\mathrm{What}\:\mathrm{does}\:\natural\mathrm{uniformly}\:\mathrm{continous}\varepsilon\:\mathrm{mean}? \\ $$ $$\mathrm{The}\:\mathrm{answer}\:\mathrm{to}\:\mathrm{your}\:\mathrm{question}\:\mathrm{is}\:\mathrm{easy}\:\mathrm{once} \\ $$ $$\mathrm{you}\:\mathrm{understood}\:\mathrm{the}\:\mathrm{definition}. \\ $$
Answered by 123564 last updated on 20/Jan/23