Question and Answers Forum

All Question   Topic List

Question Number 184287 by Tawa11 last updated on 04/Jan/23

If the area enclosed between the curves y=x² and the line y = 2x is rotated round the x-axis through 4 right angles, find the volume of the solid generated\\n

Answered by mr W last updated on 04/Jan/23

y_1 =2x y_2 =x^2 2x=x^2 ⇒x=0, x=2 V=∫_0 ^2 π(y_1 ^2 −y_2 ^2 )dx =∫_0 ^2 π(4x^2 −x^4 )dx =π[((4x^3 )/3)−(x^5 /5)]_0 ^2 =π(((4×8)/3)−((32)/5)) =((64π)/(15))

$${y}_{\mathrm{1}} =\mathrm{2}{x} \\ $$ $${y}_{\mathrm{2}} ={x}^{\mathrm{2}} \\ $$ $$\mathrm{2}{x}={x}^{\mathrm{2}} \:\Rightarrow{x}=\mathrm{0},\:{x}=\mathrm{2} \\ $$ $${V}=\int_{\mathrm{0}} ^{\mathrm{2}} \pi\left({y}_{\mathrm{1}} ^{\mathrm{2}} −{y}_{\mathrm{2}} ^{\mathrm{2}} \right){dx} \\ $$ $$\:\:\:=\int_{\mathrm{0}} ^{\mathrm{2}} \pi\left(\mathrm{4}{x}^{\mathrm{2}} −{x}^{\mathrm{4}} \right){dx} \\ $$ $$\:\:\:=\pi\left[\frac{\mathrm{4}{x}^{\mathrm{3}} }{\mathrm{3}}−\frac{{x}^{\mathrm{5}} }{\mathrm{5}}\right]_{\mathrm{0}} ^{\mathrm{2}} \\ $$ $$\:\:\:=\pi\left(\frac{\mathrm{4}×\mathrm{8}}{\mathrm{3}}−\frac{\mathrm{32}}{\mathrm{5}}\right) \\ $$ $$\:\:\:=\frac{\mathrm{64}\pi}{\mathrm{15}} \\ $$

Commented byTawa11 last updated on 04/Jan/23

I appreciate sir.

$$\mathrm{I}\:\mathrm{appreciate}\:\mathrm{sir}. \\ $$

Answered by mr W last updated on 04/Jan/23

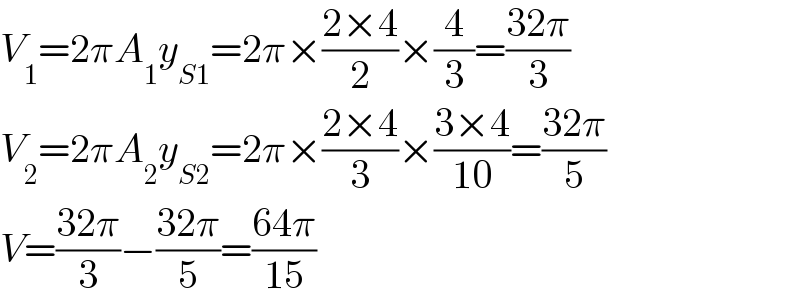
V_1 =2πA_1 y_(S1) =2π×((2×4)/2)×(4/3)=((32π)/3) V_2 =2πA_2 y_(S2) =2π×((2×4)/3)×((3×4)/(10))=((32π)/5) V=((32π)/3)−((32π)/5)=((64π)/(15))

$${V}_{\mathrm{1}} =\mathrm{2}\pi{A}_{\mathrm{1}} {y}_{{S}\mathrm{1}} =\mathrm{2}\pi×\frac{\mathrm{2}×\mathrm{4}}{\mathrm{2}}×\frac{\mathrm{4}}{\mathrm{3}}=\frac{\mathrm{32}\pi}{\mathrm{3}} \\ $$ $${V}_{\mathrm{2}} =\mathrm{2}\pi{A}_{\mathrm{2}} {y}_{{S}\mathrm{2}} =\mathrm{2}\pi×\frac{\mathrm{2}×\mathrm{4}}{\mathrm{3}}×\frac{\mathrm{3}×\mathrm{4}}{\mathrm{10}}=\frac{\mathrm{32}\pi}{\mathrm{5}} \\ $$ $${V}=\frac{\mathrm{32}\pi}{\mathrm{3}}−\frac{\mathrm{32}\pi}{\mathrm{5}}=\frac{\mathrm{64}\pi}{\mathrm{15}} \\ $$

Commented byTawa11 last updated on 05/Jan/23

Thanks for your time sir. God bless you.

$$\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time}\:\mathrm{sir}. \\ $$ $$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$

Commented byTawa11 last updated on 05/Jan/23

Can I see the diagram sir?

$$\mathrm{Can}\:\mathrm{I}\:\mathrm{see}\:\mathrm{the}\:\mathrm{diagram}\:\mathrm{sir}? \\ $$

Commented bymr W last updated on 05/Jan/23

diagram for what?

$${diagram}\:{for}\:{what}? \\ $$

Commented byTawa11 last updated on 05/Jan/23

Graph I mean sir. For the volume as the area rotate. Is ut possible sir.

$$\mathrm{Graph}\:\mathrm{I}\:\mathrm{mean}\:\mathrm{sir}. \\ $$ $$\mathrm{For}\:\mathrm{the}\:\mathrm{volume}\:\mathrm{as}\:\mathrm{the}\:\mathrm{area}\:\mathrm{rotate}. \\ $$ $$\mathrm{Is}\:\mathrm{ut}\:\mathrm{possible}\:\mathrm{sir}. \\ $$

Commented bymr W last updated on 05/Jan/23

i assumed you know how the graph for y=2x and y=x^2 is.

$${i}\:{assumed}\:{you}\:{know}\:{how}\:{the}\:{graph} \\ $$ $${for}\:{y}=\mathrm{2}{x}\:{and}\:{y}={x}^{\mathrm{2}} \:{is}. \\ $$

Commented byTawa11 last updated on 05/Jan/23

Yes sir. Very well.

$$\mathrm{Yes}\:\mathrm{sir}.\:\mathrm{Very}\:\mathrm{well}. \\ $$

Commented bymr W last updated on 05/Jan/23

see Q158209 which you have read.

$${see}\:{Q}\mathrm{158209}\:{which}\:{you}\:{have}\:{read}. \\ $$

Commented byTawa11 last updated on 05/Jan/23

Seen sir, I understand now. God bless you sir.

$$\mathrm{Seen}\:\mathrm{sir},\:\mathrm{I}\:\mathrm{understand}\:\mathrm{now}. \\ $$ $$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com