
Question Number 181602 by ali009 last updated on 27/Nov/22
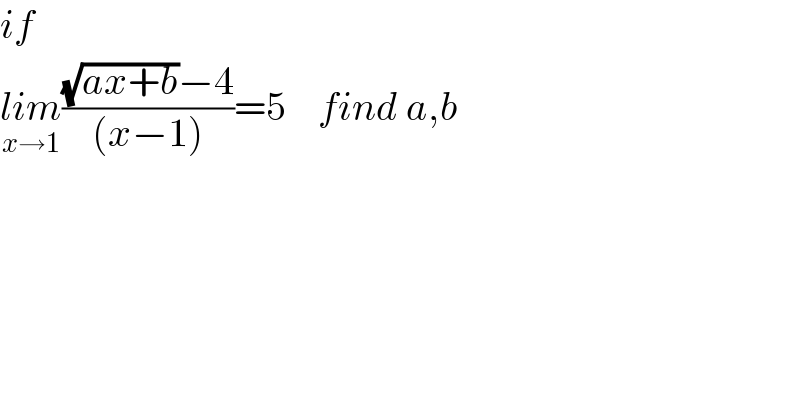
$${if}\: \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {{lim}}\frac{\sqrt{{ax}+{b}}−\mathrm{4}}{\left({x}−\mathrm{1}\right)}=\mathrm{5}\:\:\:\:{find}\:{a},{b} \\ $$
Answered by aleks041103 last updated on 27/Nov/22
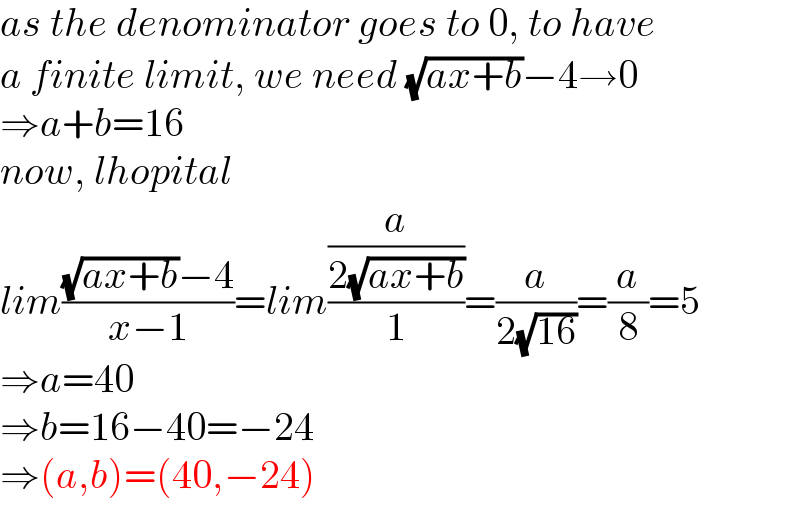
$${as}\:{the}\:{denominator}\:{goes}\:{to}\:\mathrm{0},\:{to}\:{have} \\ $$$${a}\:{finite}\:{limit},\:{we}\:{need}\:\sqrt{{ax}+{b}}−\mathrm{4}\rightarrow\mathrm{0} \\ $$$$\Rightarrow{a}+{b}=\mathrm{16} \\ $$$${now},\:{lhopital} \\ $$$${lim}\frac{\sqrt{{ax}+{b}}−\mathrm{4}}{{x}−\mathrm{1}}={lim}\frac{\frac{{a}}{\mathrm{2}\sqrt{{ax}+{b}}}}{\mathrm{1}}=\frac{{a}}{\mathrm{2}\sqrt{\mathrm{16}}}=\frac{{a}}{\mathrm{8}}=\mathrm{5} \\ $$$$\Rightarrow{a}=\mathrm{40} \\ $$$$\Rightarrow{b}=\mathrm{16}−\mathrm{40}=−\mathrm{24} \\ $$$$\Rightarrow\left({a},{b}\right)=\left(\mathrm{40},−\mathrm{24}\right) \\ $$
Answered by cortano1 last updated on 27/Nov/22
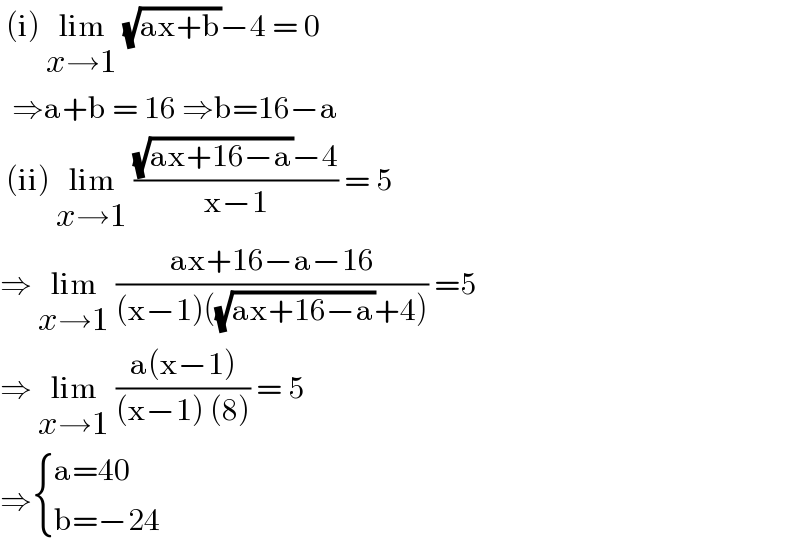
$$\:\left(\mathrm{i}\right)\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\sqrt{\mathrm{ax}+\mathrm{b}}−\mathrm{4}\:=\:\mathrm{0} \\ $$$$\:\:\Rightarrow\mathrm{a}+\mathrm{b}\:=\:\mathrm{16}\:\Rightarrow\mathrm{b}=\mathrm{16}−\mathrm{a} \\ $$$$\:\left(\mathrm{ii}\right)\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{ax}+\mathrm{16}−\mathrm{a}}−\mathrm{4}}{\mathrm{x}−\mathrm{1}}\:=\:\mathrm{5} \\ $$$$\Rightarrow\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\mathrm{ax}+\mathrm{16}−\mathrm{a}−\mathrm{16}}{\left(\mathrm{x}−\mathrm{1}\right)\left(\sqrt{\mathrm{ax}+\mathrm{16}−\mathrm{a}}+\mathrm{4}\right)}\:=\mathrm{5} \\ $$$$\Rightarrow\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\mathrm{a}\left(\mathrm{x}−\mathrm{1}\right)}{\left(\mathrm{x}−\mathrm{1}\right)\:\left(\mathrm{8}\right)}\:=\:\mathrm{5} \\ $$$$\Rightarrow\begin{cases}{\mathrm{a}=\mathrm{40}}\\{\mathrm{b}=−\mathrm{24}}\end{cases} \\ $$
