
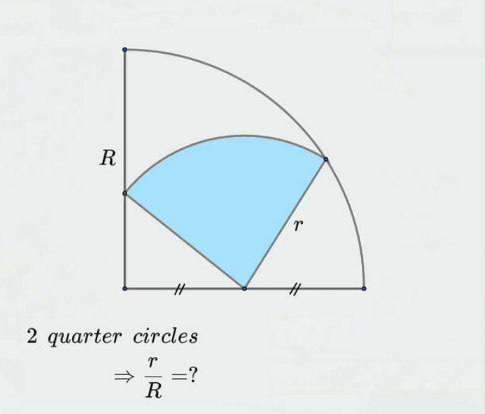
Question Number 177784 by mnjuly1970 last updated on 09/Oct/22
Answered by aleks041103 last updated on 09/Oct/22
Commented by aleks041103 last updated on 09/Oct/22

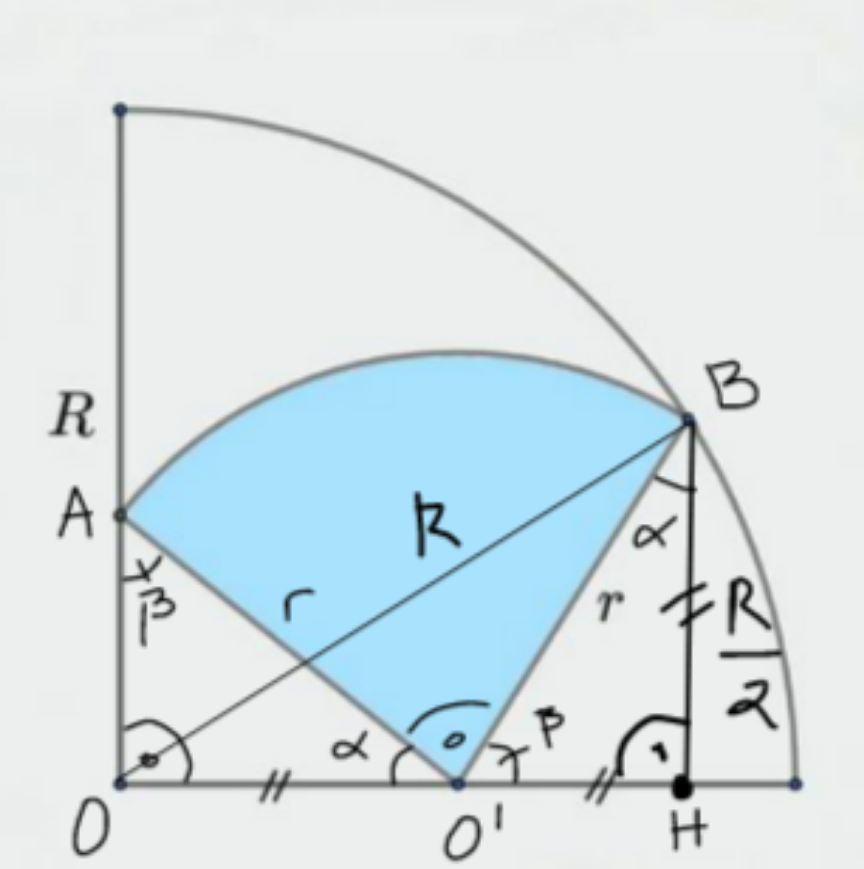
$${construct} \\ $$$${H}\in{OO}',\:{s}.{t}.\:{BH}\bot{OO}' \\ $$$$\Delta{OO}'{A}\left(\measuredangle{O}=\mathrm{90}°\right)\Rightarrow\measuredangle{O}'+\measuredangle{A}=\mathrm{90}° \\ $$$$\Rightarrow\alpha+\beta=\mathrm{90}° \\ $$$${But}\:{AOB}\:{is}\:{a}\:{quarter}\:{circle} \\ $$$$\Rightarrow\measuredangle{AO}'{B}=\mathrm{90}° \\ $$$${But} \\ $$$$\measuredangle{OO}'{A}+\measuredangle{AO}'{B}+\measuredangle{BO}'{H}=\mathrm{180}° \\ $$$$\Rightarrow\alpha+\measuredangle{BO}'{H}=\mathrm{90}° \\ $$$$\Rightarrow\measuredangle{BO}'{H}=\beta \\ $$$${But}\:{by}\:{construction}\:{for}\:\Delta{HO}'{B}\left(\measuredangle{H}=\mathrm{90}°\right) \\ $$$$\Rightarrow\measuredangle{HO}'{B}+\measuredangle{O}'{BH}=\mathrm{90}° \\ $$$$\Rightarrow\measuredangle{O}'{BH}=\alpha \\ $$$$\Rightarrow\Delta{O}'{OA}=\Delta{BHO}' \\ $$$${since}: \\ $$$$\mathrm{1}.\:{both}\:{are}\:{right}\:\Delta \\ $$$$\mathrm{2}.\:\measuredangle{O}'{BH}=\measuredangle{OO}'{A} \\ $$$$\mathrm{3}.\:{AO}'={O}'{B}={r} \\ $$$$\Rightarrow{BH}={OO}'=\frac{{R}}{\mathrm{2}} \\ $$$$\measuredangle{OHB}=\mathrm{90}°\:{and}\:{OB}={R} \\ $$$$\Rightarrow{OH}=\sqrt{{R}^{\mathrm{2}} −\left(\frac{{R}}{\mathrm{2}}\right)^{\mathrm{2}} }=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{R} \\ $$$$\Rightarrow{O}'{H}={OH}−{OO}'=\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{2}}{R} \\ $$$$\Rightarrow{O}'{B}=\sqrt{\left(\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{2}}{R}\right)^{\mathrm{2}} +\left(\frac{{R}}{\mathrm{2}}\right)^{\mathrm{2}} }= \\ $$$$=\frac{{R}}{\mathrm{2}}\sqrt{\left(\sqrt{\mathrm{3}}−\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}}=\frac{\sqrt{\mathrm{5}−\mathrm{2}\sqrt{\mathrm{3}}}}{\mathrm{2}}{R}={r} \\ $$$$\Rightarrow\frac{{r}}{{R}}=\frac{\sqrt{\mathrm{5}−\mathrm{2}\sqrt{\mathrm{3}}}}{\mathrm{2}} \\ $$
Commented by Tawa11 last updated on 09/Oct/22

$$\mathrm{Great}\:\mathrm{sir}. \\ $$
