
Question Number 171614 by Tawa11 last updated on 18/Jun/22

A particle is moving along a straight line such that it's position from a fixed point is S = ( 12 - 15t² + 5t³ )m where t is in seconds. Determine: A. Total distance travelled by the particle from t = 1sec to t = 3sec B. The average speed of the particle during this time.\\n
Commented byTawa11 last updated on 18/Jun/22
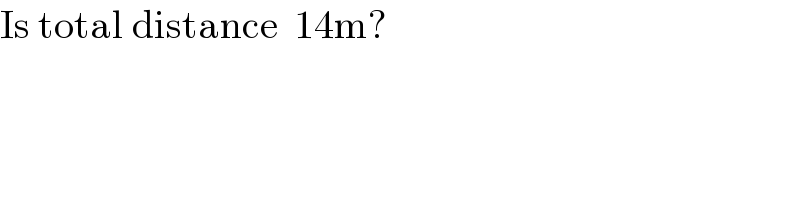
$$\mathrm{Is}\:\mathrm{total}\:\mathrm{distance}\:\:\mathrm{14m}? \\ $$
Commented byBeginner last updated on 18/Jun/22
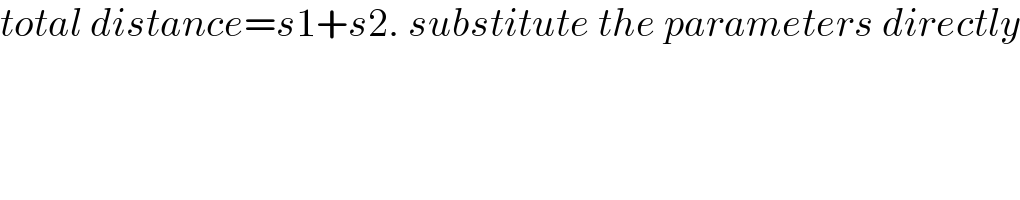
$${total}\:{distance}={s}\mathrm{1}+{s}\mathrm{2}.\:{substitute}\:{the}\:{parameters}\:{directly} \\ $$ $$ \\ $$
Commented bymr W last updated on 18/Jun/22

$$\mathrm{30}\:{m} \\ $$
Commented bymr W last updated on 19/Jun/22

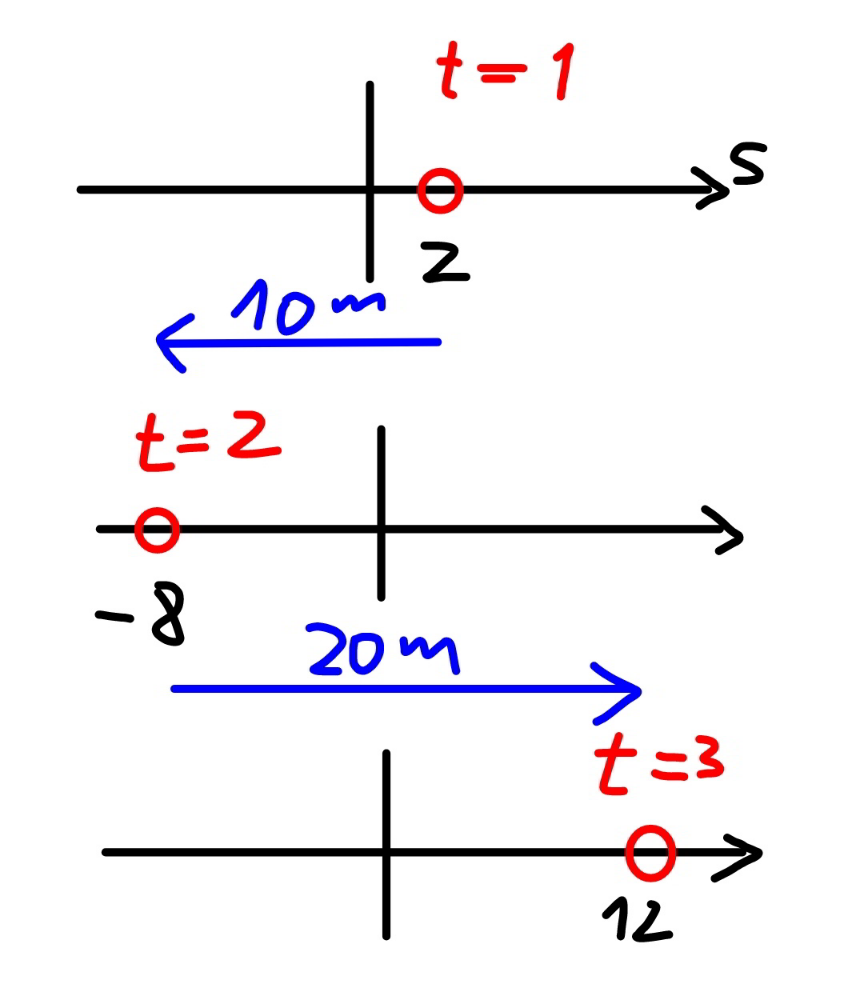
$${at}\:\mathrm{1}\:{o}'{clock}\:{you}\:{are}\:\mathrm{2}\:{km}\:{easterly}\: \\ $$ $${apart}\:{from}\:{your}\:{house}, \\ $$ $${at}\:\mathrm{2}\:{o}'{clock}\:{you}\:{are}\:\mathrm{8}\:{km}\:{westerly}\: \\ $$ $${apart}\:{from}\:{your}\:{house}, \\ $$ $${that}\:{means}\:{from}\:\mathrm{1}\:{o}'{clock}\:{to}\:\mathrm{2}\:{o}'{clock} \\ $$ $${you}\:{walked}\:\mathrm{10}\:{km}\:{in}\:{direction} \\ $$ $${from}\:{east}\:{to}\:{west}. \\ $$ $$ \\ $$ $${at}\:\mathrm{3}\:{o}'{clock}\:{you}\:{are}\:{again}\:\mathrm{12}\:{km}\:{easterly}\: \\ $$ $${apart}\:{from}\:{your}\:{house}, \\ $$ $${that}\:{means}\:{from}\:\mathrm{2}\:{o}'{clock}\:{to}\:\mathrm{3}\:{o}'{clock} \\ $$ $${you}\:{walked}\:\mathrm{20}\:{km}\:{in}\:{direction} \\ $$ $${from}\:{west}\:{to}\:{east}. \\ $$ $$ \\ $$ $${that}\:{means}\:{from}\:\mathrm{1}\:{o}'{clock}\:{to}\:\mathrm{3}\:{o}'{clock} \\ $$ $${you}\:{walked}\:{totally}\:\mathrm{30}\:{km}. \\ $$
Commented bymr W last updated on 19/Jun/22
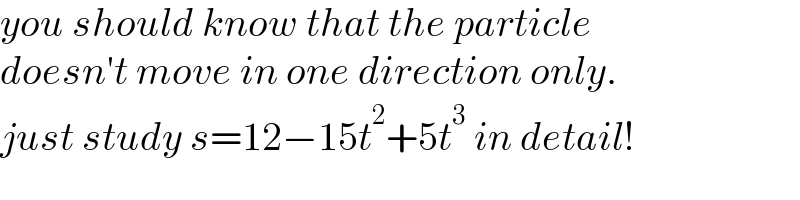
$${you}\:{should}\:{know}\:{that}\:{the}\:{particle} \\ $$ $${doesn}'{t}\:{move}\:{in}\:{one}\:{direction}\:{only}. \\ $$ $${just}\:{study}\:{s}=\mathrm{12}−\mathrm{15}{t}^{\mathrm{2}} +\mathrm{5}{t}^{\mathrm{3}} \:{in}\:{detail}! \\ $$
Commented bymr W last updated on 19/Jun/22
Commented byTawa11 last updated on 19/Jun/22
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sirs} \\ $$
Commented bymr W last updated on 19/Jun/22

$$\mathrm{\color{mathred}{D}\color{mathred}{i}\color{mathred}{s}\color{mathred}{t}\color{mathred}{a}\color{mathred}{n}\color{mathred}{c}\color{mathred}{e}}\color{mathred}{\:}\mathrm{\color{mathred}{t}\color{mathred}{r}\color{mathred}{a}\color{mathred}{v}\color{mathred}{e}\color{mathred}{l}\color{mathred}{e}\color{mathred}{d}}\:\mathrm{is}\:\mathrm{the}\:\mathrm{total}\:\mathrm{length}\: \\ $$ $$\mathrm{of}\:\mathrm{the}\:\mathrm{path}\:\mathrm{traveled}\:\mathrm{between}\:\mathrm{two} \\ $$ $$\mathrm{position}{s}. \\ $$ $${In}\:{current}\:{example}\:{the}\:{particle}\:{traveled} \\ $$ $${a}\:{path}\:{of}\:\mathrm{30}{m}\:{length}\:{in}\:{the}\:{second} \\ $$ $${and}\:{third}\:{second}.\:{so}\:{the}\:{distance} \\ $$ $${traveled}\:{is}\:\mathrm{30}{m}. \\ $$
Commented bymr W last updated on 19/Jun/22
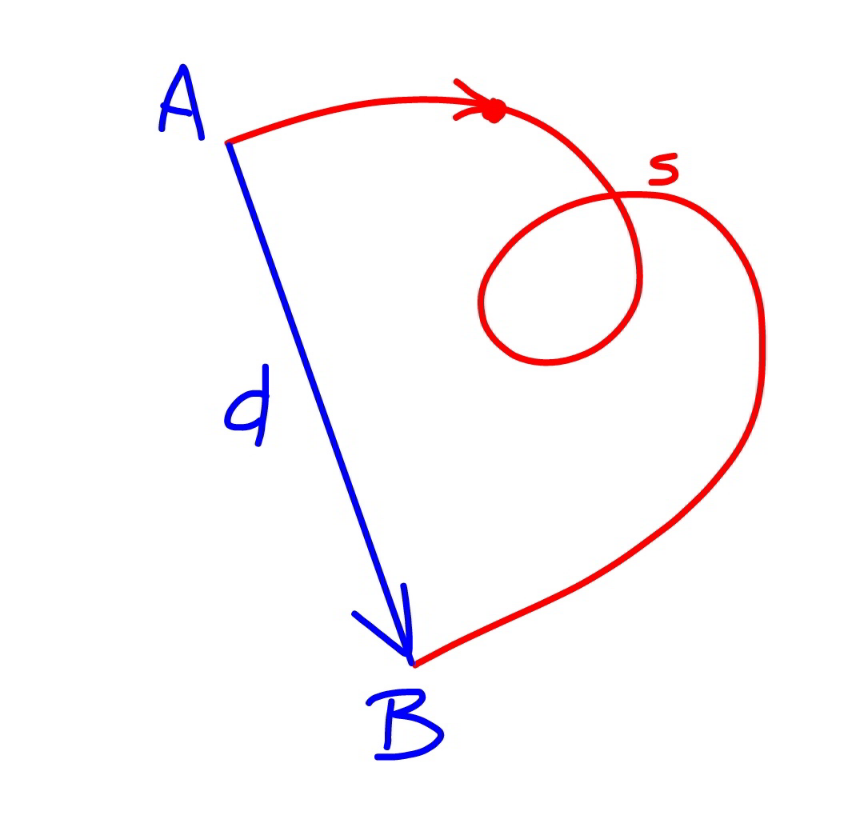
Commented bymr W last updated on 19/Jun/22

$${an}\:{object}\:{moved}\:{from}\:{A}\:{to}\:{B}\: \\ $$ $${following}\:{the}\:{red}\:{path}\:{with}\:{length}\:{s}. \\ $$ $${displacement}=\overset{\rightarrow} {{AB}} \\ $$ $${distance}=\mid\overset{\rightarrow} {{AB}}\mid={d} \\ $$ $${distance}\:{traveled}={length}\:{of}\:{path}={s} \\ $$
Commented byTawa11 last updated on 21/Jun/22
![Wow, I just finished studying your concept sir. Here displacement still end up in 10m That is (− 8 − 2) + [12 − (− 8)] = 10m. And Distance = 8 + 2 + 20 = 30m](Q171797.png)
$$\mathrm{Wow},\:\mathrm{I}\:\mathrm{just}\:\mathrm{finished}\:\mathrm{studying}\:\mathrm{your}\:\mathrm{concept}\:\mathrm{sir}. \\ $$ $$\mathrm{Here}\:\mathrm{displacement}\:\mathrm{still}\:\mathrm{end}\:\mathrm{up}\:\mathrm{in}\:\:\mathrm{10m} \\ $$ $$\mathrm{That}\:\mathrm{is}\:\:\:\:\:\left(−\:\:\:\mathrm{8}\:\:\:−\:\:\:\mathrm{2}\right)\:\:+\:\:\left[\mathrm{12}\:\:\:−\:\:\left(−\:\:\mathrm{8}\right)\right]\:\:\:=\:\:\:\mathrm{10m}. \\ $$ $$\mathrm{And} \\ $$ $$\mathrm{Distance}\:\:\:=\:\:\:\mathrm{8}\:\:+\:\:\mathrm{2}\:\:+\:\:\mathrm{20}\:\:\:=\:\:\:\mathrm{30m} \\ $$
