
Question Number 171642 by infinityaction last updated on 19/Jun/22
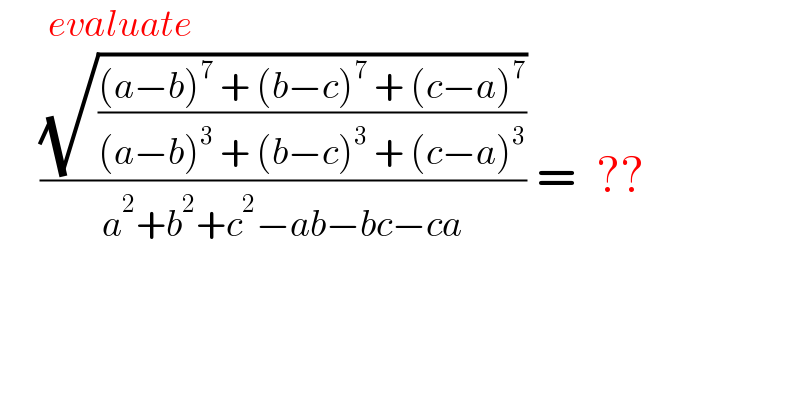
$$\:\:\:\:\:\:\:\:{evaluate}\:\:\: \\ $$$$\:\:\:\:\frac{\sqrt{\frac{\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} }{\left({a}−{b}\right)^{\mathrm{3}} \:+\:\left({b}−{c}\right)^{\mathrm{3}} \:+\:\left({c}−{a}\right)^{\mathrm{3}} }}}{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}}\:=\:\:?? \\ $$
Commented by infinityaction last updated on 19/Jun/22
![let a−b = 𝛂 , b−c = 𝛃 , c−a = 𝛄 𝛂+𝛃+𝛄 = Σα = 0 𝛂^3 +𝛃^3 +𝛄^3 = Σα^3 = 3𝛂𝛃𝛄 then x^3 +(𝛂𝛃+𝛃𝛄+𝛄𝛂)x−𝛂𝛃𝛄 = 0 this equations has roots 𝛂 ,𝛃 ,𝛄 αβγ = P and αβ+βγ+γα = Q x^3 = P− Qx x^7 = Px.x^3 − Qx^2 .x^3 x^7 = Px(P−Qx)−x^2 Q(P−Qx) x^7 = P^2 x − PQx^2 −PQx^2 +Q^2 x^3 x^7 = P^2 x−2PQx^2 +Q^2 x^3 𝛂^(7 ) +𝛃^7 +𝛄^7 = P 𝚺𝛂 −2PQ 𝚺𝛂^2 + Q^2 𝚺𝛂^3 𝚺𝛂^7 = 3PQ^(2 ) −2PQ{ (𝚺𝛂)^2 −2𝚺𝛂𝛃 } 𝚺𝛂^7 = 3PQ^2 −2PQ(−2Q) 𝚺𝛂^(7 ) = 7PQ^2 and 𝚺𝛂^3 = 3P ((𝚺𝛂^7 )/(𝚺𝛂^3 )) = (7/3)Q a^2 +b^2 +c^2 −ab−bc−ca = (1/2)[(a−b)^2 +(b−c)^2 +(c−a)^2 ] a^2 +b^2 +c^2 −ab−bc−ca = (1/2){𝛂^2 +𝛃^2 +𝛄^2 } a^2 +b^2 +c^2 −ab−bc−ca = (1/2){−2Q}= −Q so ∣ ((√((7/3)Q^2 ))/(−Q)) ∣= ∣(((√((7/3) ))Q)/(−Q))∣ = (√(7/3))](Q171651.png)
$$\:\:\:\boldsymbol{{let}}\:\boldsymbol{{a}}−\boldsymbol{{b}}\:=\:\boldsymbol{\alpha}\:,\:\boldsymbol{{b}}−\boldsymbol{{c}}\:=\:\boldsymbol{\beta}\:,\:\boldsymbol{{c}}−\boldsymbol{{a}}\:=\:\boldsymbol{\gamma} \\ $$$$\:\:\:\boldsymbol{\alpha}+\boldsymbol{\beta}+\boldsymbol{\gamma}\:=\:\Sigma\alpha\:=\:\mathrm{0}\:\: \\ $$$$\:\:\:\boldsymbol{\alpha}^{\mathrm{3}} +\boldsymbol{\beta}^{\mathrm{3}} +\boldsymbol{\gamma}^{\mathrm{3}} \:=\:\Sigma\alpha^{\mathrm{3}} \:=\:\:\mathrm{3}\boldsymbol{\alpha\beta\gamma} \\ $$$$\:\:\:\:\:\:\:\:{then} \\ $$$$\:\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{3}} +\left(\boldsymbol{\alpha\beta}+\boldsymbol{\beta\gamma}+\boldsymbol{\gamma\alpha}\right)\boldsymbol{{x}}−\boldsymbol{\alpha\beta\gamma}\:=\:\mathrm{0} \\ $$$$\:\:\:\:\boldsymbol{{this}}\:\boldsymbol{{equations}}\:\boldsymbol{{has}}\:\boldsymbol{{roots}}\:\boldsymbol{\alpha}\:,\boldsymbol{\beta}\:,\boldsymbol{\gamma} \\ $$$$\:\:\:\:\:\:\alpha\beta\gamma\:=\:{P}\:\:\:{and}\:\alpha\beta+\beta\gamma+\gamma\alpha\:=\:{Q} \\ $$$$\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{3}} \:=\:{P}−\:{Qx} \\ $$$$\:\:\:\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{7}} \:=\:\boldsymbol{{Px}}.\boldsymbol{{x}}^{\mathrm{3}} \:−\:\boldsymbol{{Qx}}^{\mathrm{2}} .\boldsymbol{{x}}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{7}} \:=\:\boldsymbol{{Px}}\left(\boldsymbol{{P}}−\boldsymbol{{Qx}}\right)−\boldsymbol{{x}}^{\mathrm{2}} \boldsymbol{{Q}}\left(\boldsymbol{{P}}−\boldsymbol{{Qx}}\right) \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{7}} \:=\:\boldsymbol{{P}}^{\mathrm{2}} \boldsymbol{{x}}\:−\:\boldsymbol{{PQx}}^{\mathrm{2}} −\boldsymbol{{PQx}}^{\mathrm{2}} +\boldsymbol{{Q}}^{\mathrm{2}} \boldsymbol{{x}}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\boldsymbol{{x}}^{\mathrm{7}} \:=\:\boldsymbol{{P}}^{\mathrm{2}} \boldsymbol{{x}}−\mathrm{2}\boldsymbol{{PQx}}^{\mathrm{2}} +\boldsymbol{{Q}}^{\mathrm{2}} \boldsymbol{{x}}^{\mathrm{3}} \\ $$$$\:\:\boldsymbol{\alpha}^{\mathrm{7}\:} +\boldsymbol{\beta}^{\mathrm{7}} +\boldsymbol{\gamma}^{\mathrm{7}} \:=\:\boldsymbol{{P}}\:\boldsymbol{\Sigma\alpha}\:−\mathrm{2}\boldsymbol{{PQ}}\:\boldsymbol{\Sigma\alpha}^{\mathrm{2}} \:+\:\boldsymbol{{Q}}^{\mathrm{2}} \boldsymbol{\Sigma\alpha}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\boldsymbol{\Sigma\alpha}^{\mathrm{7}} \:=\:\mathrm{3}\boldsymbol{{PQ}}^{\mathrm{2}\:} −\mathrm{2}\boldsymbol{{PQ}}\left\{\:\left(\boldsymbol{\Sigma\alpha}\right)^{\mathrm{2}} −\mathrm{2}\boldsymbol{\Sigma\alpha\beta}\:\right\} \\ $$$$\:\:\:\:\:\:\boldsymbol{\Sigma\alpha}^{\mathrm{7}} \:=\:\mathrm{3}\boldsymbol{{PQ}}^{\mathrm{2}} −\mathrm{2}\boldsymbol{{PQ}}\left(−\mathrm{2}\boldsymbol{{Q}}\right) \\ $$$$\:\:\:\:\:\:\boldsymbol{\Sigma\alpha}^{\mathrm{7}\:} =\:\mathrm{7}\boldsymbol{{PQ}}^{\mathrm{2}} \:\:\boldsymbol{{and}}\:\:\boldsymbol{\Sigma\alpha}^{\mathrm{3}} \:=\:\mathrm{3}\boldsymbol{{P}} \\ $$$$\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{\Sigma\alpha}^{\mathrm{7}} }{\boldsymbol{\Sigma\alpha}^{\mathrm{3}} }\:\:=\:\:\frac{\mathrm{7}}{\mathrm{3}}\boldsymbol{{Q}} \\ $$$$\:\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{c}}^{\mathrm{2}} −\boldsymbol{{ab}}−\boldsymbol{{bc}}−\boldsymbol{{ca}}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left[\left(\boldsymbol{{a}}−\boldsymbol{{b}}\right)^{\mathrm{2}} +\left(\boldsymbol{{b}}−\boldsymbol{{c}}\right)^{\mathrm{2}} +\left(\boldsymbol{{c}}−\boldsymbol{{a}}\right)^{\mathrm{2}} \right] \\ $$$$\:\:\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{c}}^{\mathrm{2}} −\boldsymbol{{ab}}−\boldsymbol{{bc}}−\boldsymbol{{ca}}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left\{\boldsymbol{\alpha}^{\mathrm{2}} +\boldsymbol{\beta}^{\mathrm{2}} +\boldsymbol{\gamma}^{\mathrm{2}} \right\} \\ $$$$\:\:\:\:\:\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{c}}^{\mathrm{2}} −\boldsymbol{{ab}}−\boldsymbol{{bc}}−\boldsymbol{{ca}}\:=\:\:\frac{\mathrm{1}}{\mathrm{2}}\left\{−\mathrm{2}\boldsymbol{{Q}}\right\}=\:−\boldsymbol{{Q}} \\ $$$$\:\:\:{so} \\ $$$$\:\:\:\:\:\:\:\mid\:\:\frac{\sqrt{\frac{\mathrm{7}}{\mathrm{3}}\boldsymbol{{Q}}^{\mathrm{2}} }}{−\boldsymbol{{Q}}}\:\:\mid=\:\:\mid\frac{\sqrt{\frac{\mathrm{7}}{\mathrm{3}}\:}\boldsymbol{{Q}}}{−\boldsymbol{{Q}}}\mid\:=\:\sqrt{\frac{\mathrm{7}}{\mathrm{3}}} \\ $$
Commented by Rasheed.Sindhi last updated on 19/Jun/22
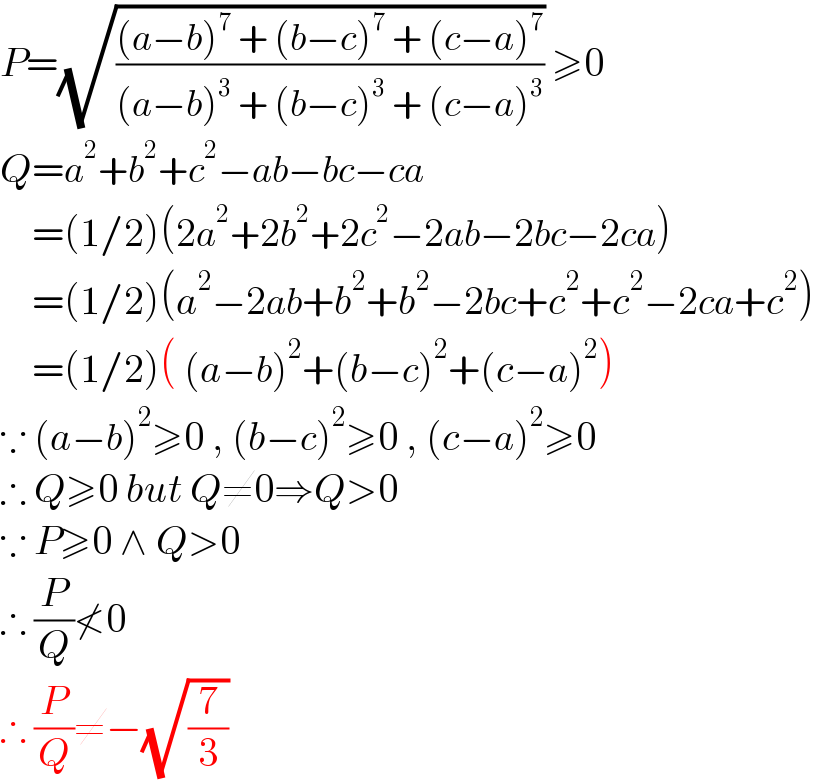
$${P}=\sqrt{\frac{\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} }{\left({a}−{b}\right)^{\mathrm{3}} \:+\:\left({b}−{c}\right)^{\mathrm{3}} \:+\:\left({c}−{a}\right)^{\mathrm{3}} }}\:\geqslant\mathrm{0} \\ $$$${Q}={a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca} \\ $$$$\:\:\:\:=\left(\mathrm{1}/\mathrm{2}\right)\left(\mathrm{2}{a}^{\mathrm{2}} +\mathrm{2}{b}^{\mathrm{2}} +\mathrm{2}{c}^{\mathrm{2}} −\mathrm{2}{ab}−\mathrm{2}{bc}−\mathrm{2}{ca}\right) \\ $$$$\:\:\:\:=\left(\mathrm{1}/\mathrm{2}\right)\left({a}^{\mathrm{2}} −\mathrm{2}{ab}+{b}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}{bc}+{c}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{2}{ca}+{c}^{\mathrm{2}} \right) \\ $$$$\:\:\:\:=\left(\mathrm{1}/\mathrm{2}\right)\left(\:\left({a}−{b}\right)^{\mathrm{2}} +\left({b}−{c}\right)^{\mathrm{2}} +\left({c}−{a}\right)^{\mathrm{2}} \right) \\ $$$$\because\:\left({a}−{b}\right)^{\mathrm{2}} \geqslant\mathrm{0}\:,\:\left({b}−{c}\right)^{\mathrm{2}} \geqslant\mathrm{0}\:,\:\left({c}−{a}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$$$\therefore\:{Q}\geqslant\mathrm{0}\:{but}\:{Q}\neq\mathrm{0}\Rightarrow{Q}>\mathrm{0} \\ $$$$\because\:{P}\geqslant\mathrm{0}\:\wedge\:{Q}>\mathrm{0} \\ $$$$\therefore\:\frac{{P}}{{Q}}\nless\mathrm{0} \\ $$$$\therefore\:\frac{{P}}{{Q}}\neq−\sqrt{\frac{\mathrm{7}}{\mathrm{3}}} \\ $$
Commented by infinityaction last updated on 19/Jun/22

$${got}\:{it}\:{sir} \\ $$$${thanks} \\ $$
Commented by Rasheed.Sindhi last updated on 19/Jun/22
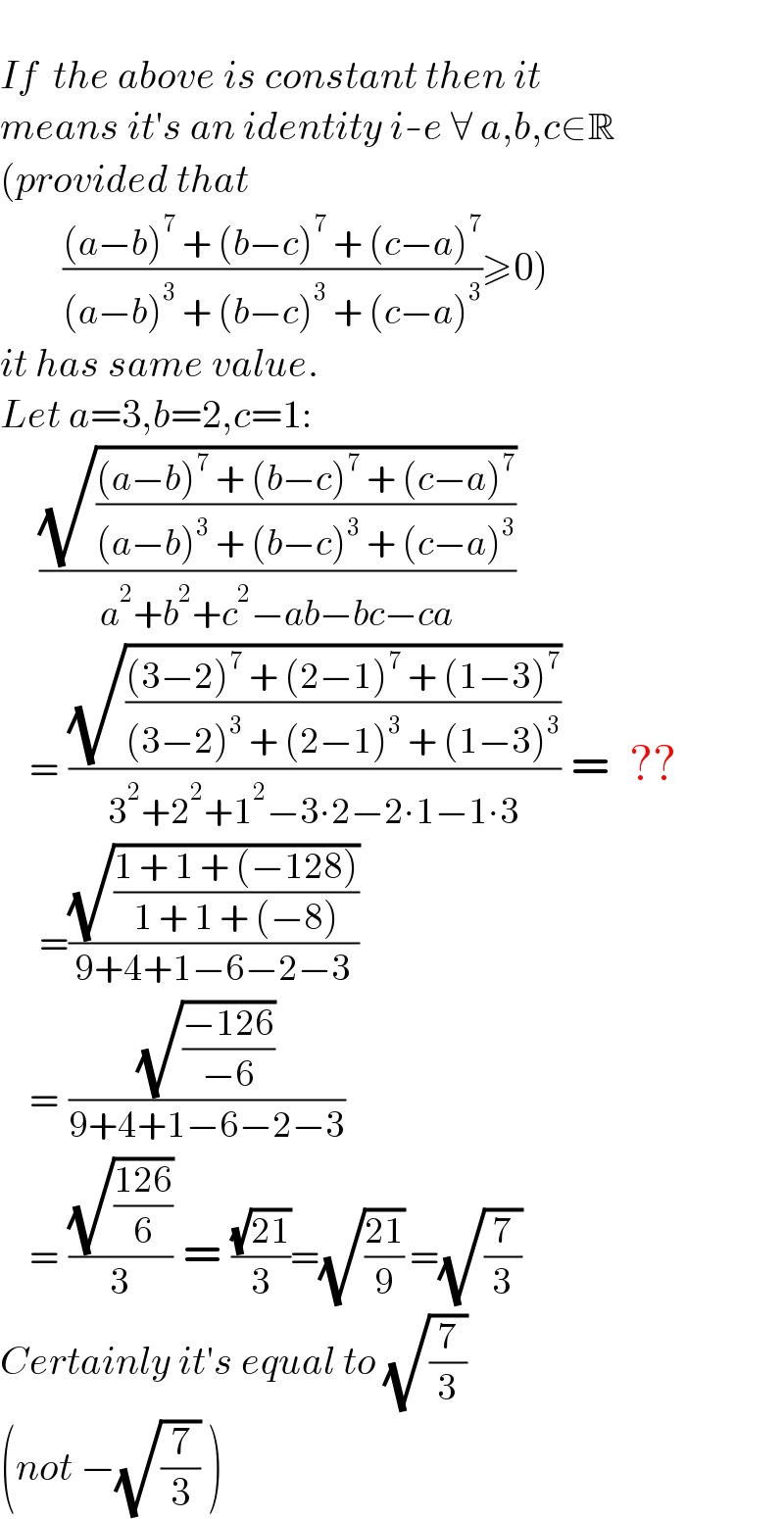
$$ \\ $$$${If}\:\:{the}\:{above}\:{is}\:{constant}\:{then}\:{it} \\ $$$${means}\:{it}'{s}\:{an}\:{identity}\:{i}-{e}\:\forall\:{a},{b},{c}\in\mathbb{R} \\ $$$$\left({provided}\:{that}\right. \\ $$$$\left.\:\:\:\:\:\:\:\:\frac{\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} }{\left({a}−{b}\right)^{\mathrm{3}} \:+\:\left({b}−{c}\right)^{\mathrm{3}} \:+\:\left({c}−{a}\right)^{\mathrm{3}} }\geqslant\mathrm{0}\right) \\ $$$${it}\:{has}\:{same}\:{value}. \\ $$$${Let}\:{a}=\mathrm{3},{b}=\mathrm{2},{c}=\mathrm{1}: \\ $$$$\:\:\:\:\frac{\sqrt{\frac{\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} }{\left({a}−{b}\right)^{\mathrm{3}} \:+\:\left({b}−{c}\right)^{\mathrm{3}} \:+\:\left({c}−{a}\right)^{\mathrm{3}} }}}{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}}\: \\ $$$$\:\:\:=\:\frac{\sqrt{\frac{\left(\mathrm{3}−\mathrm{2}\right)^{\mathrm{7}} \:+\:\left(\mathrm{2}−\mathrm{1}\right)^{\mathrm{7}} \:+\:\left(\mathrm{1}−\mathrm{3}\right)^{\mathrm{7}} }{\left(\mathrm{3}−\mathrm{2}\right)^{\mathrm{3}} \:+\:\left(\mathrm{2}−\mathrm{1}\right)^{\mathrm{3}} \:+\:\left(\mathrm{1}−\mathrm{3}\right)^{\mathrm{3}} }}}{\mathrm{3}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{1}^{\mathrm{2}} −\mathrm{3}\centerdot\mathrm{2}−\mathrm{2}\centerdot\mathrm{1}−\mathrm{1}\centerdot\mathrm{3}}\:=\:\:?? \\ $$$$\:\:\:\:=\frac{\sqrt{\frac{\mathrm{1}\:+\:\mathrm{1}\:+\:\left(−\mathrm{128}\right)}{\mathrm{1}\:+\:\mathrm{1}\:+\:\left(−\mathrm{8}\right)}}}{\mathrm{9}+\mathrm{4}+\mathrm{1}−\mathrm{6}−\mathrm{2}−\mathrm{3}}\: \\ $$$$\:\:\:=\:\frac{\sqrt{\frac{−\mathrm{126}}{−\mathrm{6}}}}{\mathrm{9}+\mathrm{4}+\mathrm{1}−\mathrm{6}−\mathrm{2}−\mathrm{3}}\: \\ $$$$\:\:\:=\:\frac{\sqrt{\frac{\mathrm{126}}{\mathrm{6}}}}{\mathrm{3}}\:=\:\frac{\sqrt{\mathrm{21}}}{\mathrm{3}}=\sqrt{\frac{\mathrm{21}}{\mathrm{9}}}\:=\sqrt{\frac{\mathrm{7}}{\mathrm{3}}} \\ $$$${Certainly}\:{it}'{s}\:{equal}\:{to}\:\sqrt{\frac{\mathrm{7}}{\mathrm{3}}} \\ $$$$\left({not}\:−\sqrt{\frac{\mathrm{7}}{\mathrm{3}}}\:\right) \\ $$
Commented by Tawa11 last updated on 25/Jun/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
Answered by Rasheed.Sindhi last updated on 19/Jun/22
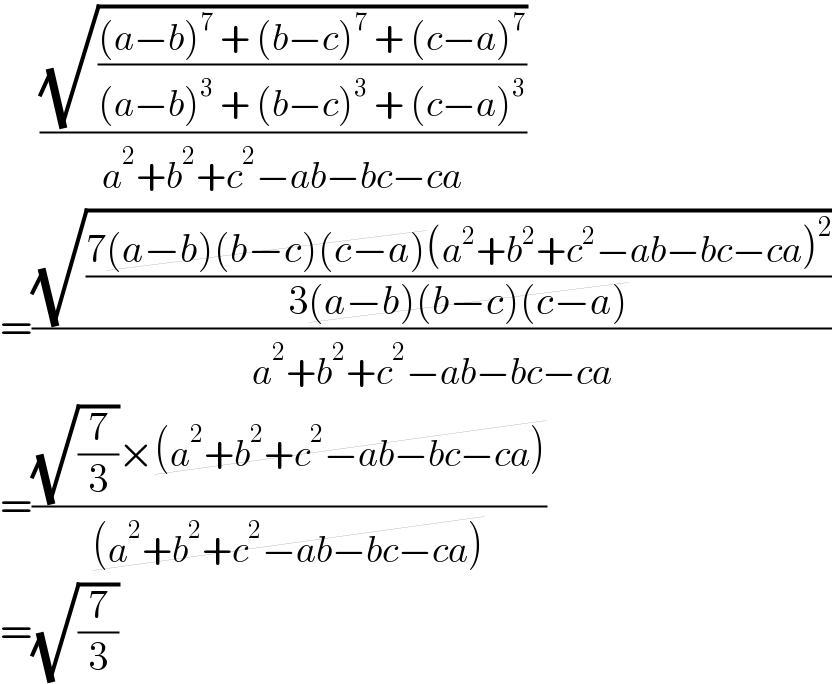
$$\:\:\:\:\frac{\sqrt{\frac{\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} }{\left({a}−{b}\right)^{\mathrm{3}} \:+\:\left({b}−{c}\right)^{\mathrm{3}} \:+\:\left({c}−{a}\right)^{\mathrm{3}} }}}{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}} \\ $$$$=\frac{\sqrt{\frac{\mathrm{7}\cancel{\left({a}−{b}\right)\left({b}−{c}\right)\left({c}−{a}\right)}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}\right)^{\mathrm{2}} }{\mathrm{3}\cancel{\left({a}−{b}\right)\left({b}−{c}\right)\left({c}−{a}\right)}}}}{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}} \\ $$$$=\frac{\sqrt{\frac{\mathrm{7}}{\mathrm{3}}}×\cancel{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}\right)}}{\cancel{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}\right)}} \\ $$$$=\sqrt{\frac{\mathrm{7}}{\mathrm{3}}} \\ $$
Commented by infinityaction last updated on 19/Jun/22

$${sir}\:{can}\:{you}\:{tell}\:{me}\:{factor}\:{of} \\ $$$$\left({a}−{b}\right)^{\mathrm{7}} +\left({b}−{c}\right)^{\mathrm{7}} +\left({c}−{a}\right)^{\mathrm{7}} \\ $$
Commented by Rasheed.Sindhi last updated on 19/Jun/22
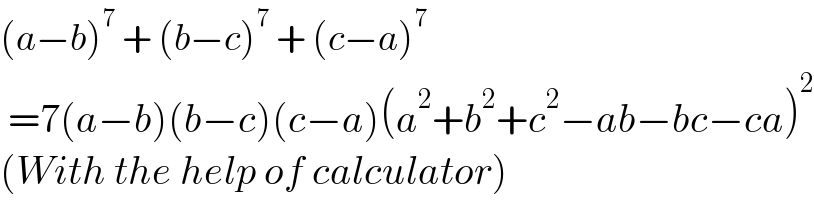
$$\left({a}−{b}\right)^{\mathrm{7}} \:+\:\left({b}−{c}\right)^{\mathrm{7}} \:+\:\left({c}−{a}\right)^{\mathrm{7}} \\ $$$$\:=\mathrm{7}\left({a}−{b}\right)\left({b}−{c}\right)\left({c}−{a}\right)\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{ab}−{bc}−{ca}\right)^{\mathrm{2}} \\ $$$$\left({With}\:{the}\:{help}\:{of}\:{calculator}\right) \\ $$
