
Question Number 168040 by cortano1 last updated on 01/Apr/22
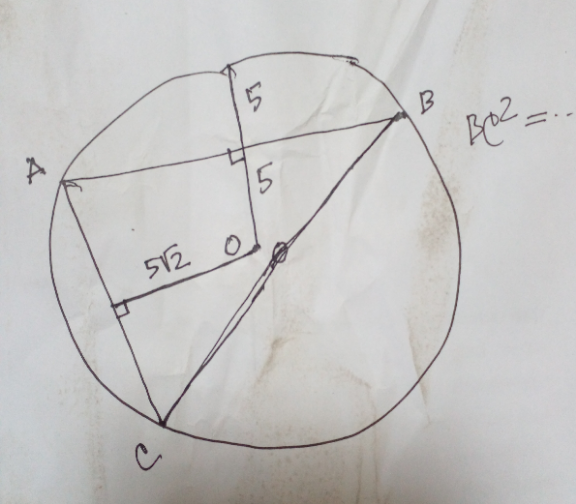
Commented by cortano1 last updated on 01/Apr/22
$${O}\:{is}\:{center}\:{point}\:{of}\:{circle} \\ $$
Commented by som(math1967) last updated on 01/Apr/22

$${BC}^{\mathrm{2}} =\mathrm{400}{sin}^{\mathrm{2}} \mathrm{75} \\ $$
Commented by cortano1 last updated on 01/Apr/22

$${i}\:{got}\:\mathrm{100}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right) \\ $$
Commented by som(math1967) last updated on 01/Apr/22

$${sin}\mathrm{75}=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$$=\frac{\left(\sqrt{\mathrm{3}}+\mathrm{1}\right)}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$\mathrm{400}{sin}^{\mathrm{2}} \mathrm{75} \\ $$$$=\mathrm{400}×\frac{\left(\sqrt{\mathrm{3}}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{8}} \\ $$$$=\mathrm{400}×\frac{\mathrm{2}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)}{\mathrm{8}}=\mathrm{100}\left(\mathrm{2}+\sqrt{\left.\mathrm{3}\right)}\right. \\ $$
