
Question Number 167682 by bounhome last updated on 22/Mar/22
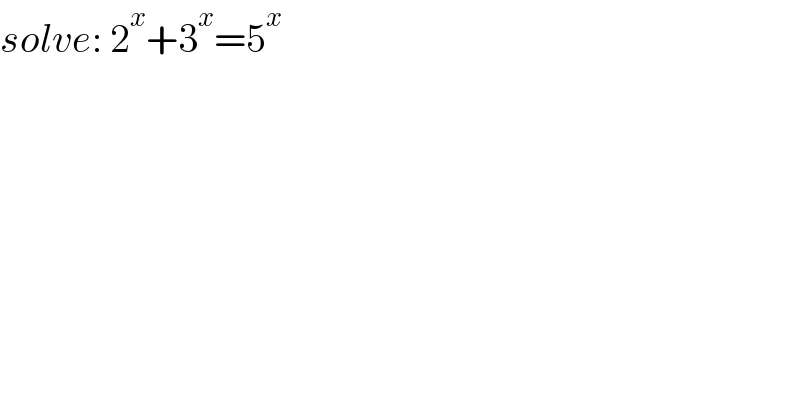
$${solve}:\:\mathrm{2}^{{x}} +\mathrm{3}^{{x}} =\mathrm{5}^{{x}} \\ $$
Commented by mr W last updated on 22/Mar/22
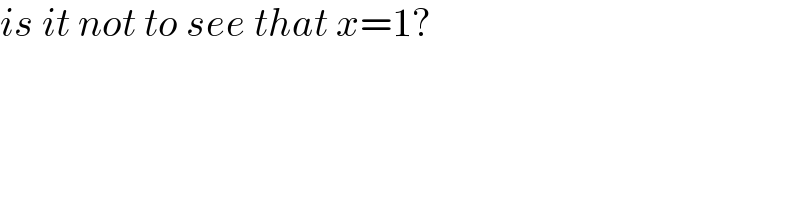
$${is}\:{it}\:{not}\:{to}\:{see}\:{that}\:{x}=\mathrm{1}? \\ $$
Answered by HeferH last updated on 22/Mar/22
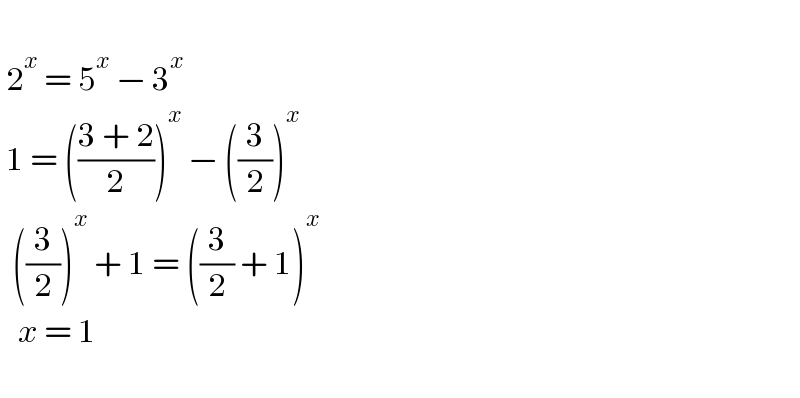
$$\: \\ $$$$\:\mathrm{2}^{{x}} \:=\:\mathrm{5}^{{x}} \:−\:\mathrm{3}^{{x}} \\ $$$$\:\mathrm{1}\:=\:\left(\frac{\mathrm{3}\:+\:\mathrm{2}}{\mathrm{2}}\right)^{{x}} \:−\:\left(\frac{\mathrm{3}}{\mathrm{2}}\right)^{{x}} \\ $$$$\:\:\left(\frac{\mathrm{3}}{\mathrm{2}}\right)^{{x}} \:+\:\mathrm{1}\:=\:\left(\frac{\mathrm{3}}{\mathrm{2}}\:+\:\mathrm{1}\right)^{{x}} \: \\ $$$$\:\:\:{x}\:=\:\mathrm{1} \\ $$$$\:\:\: \\ $$
Commented by Rasheed.Sindhi last updated on 23/Mar/22

$$\mathcal{G}{ood}\:{saying}! \\ $$
Commented by mr W last updated on 23/Mar/22

$${it}'{s}\:{easier}\:{to}\:{make}\:{easy}\:{things} \\ $$$${complex}\:{than}\:{to}\:{make}\:{complex} \\ $$$${things}\:{easy}. \\ $$
