
Question Number 16699 by mrW1 last updated on 25/Jun/17
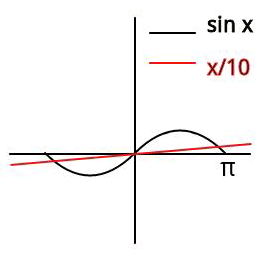
![Related to Q16675: Find the number of intersection points of graph sin x=(x/(10)). Let′s see sin x = (x/n) with n>1. For n≤1 there is one intersection point. Let x=2kπ+t with k∈N ∧ t∈[0,2π] sin x=sin t cos x=cos t we find the point on f(x)=sin x where its tangent is g(x)=(x/n). f′(x)=cos x=cos t g′(x)=(1/n) cos t=(1/n) t=cos^(−1) (1/n) sin t=(n/(√(n^2 +1))) so that f(x) intersects with g(x), ((sin x)/x)≥(1/n) ⇒n sin x≥x ⇒n sin t≥2kπ+t ⇒k≤((n sin t −t)/(2π))=(((n^2 /(√(n^2 +1)))−cos^(−1) (1/n))/(2π)) k_(max) =⌊(((n^2 /(√(n^2 +1)))−cos^(−1) (1/n))/(2π))⌋ number of intersecting points is m=2×2(k_(max) +1)−1=4k_(max) +3 for n=10 k_(max) =⌊(((n^2 /(√(n^2 +1)))−cos^(−1) (1/n))/(2π))⌋ =⌊((((10^2 )/(√(10^2 +1)))−cos^(−1) (1/(10)))/(2π))⌋=⌊1.35⌋=1 ⇒m=4×1+3=7 for n=20 k_(max) =⌊((((20^2 )/(√(20^2 +1)))−cos^(−1) (1/(20)))/(2π))⌋=⌊2.94⌋=2 ⇒m=4×2+3=11](Q16699.png)
$$\mathrm{Related}\:\mathrm{to}\:\mathrm{Q16675}: \\ $$ $$ \\ $$ $$\mathrm{Find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{intersection}\:\mathrm{points} \\ $$ $$\mathrm{of}\:\mathrm{graph}\:\mathrm{sin}\:\mathrm{x}=\frac{\mathrm{x}}{\mathrm{10}}. \\ $$ $$ \\ $$ $$\mathrm{Let}'\mathrm{s}\:\mathrm{see}\:\mathrm{sin}\:\mathrm{x}\:=\:\frac{\mathrm{x}}{\mathrm{n}}\:\mathrm{with}\:\mathrm{n}>\mathrm{1}. \\ $$ $$\mathrm{For}\:\mathrm{n}\leqslant\mathrm{1}\:\mathrm{there}\:\mathrm{is}\:\mathrm{one}\:\mathrm{intersection}\:\mathrm{point}. \\ $$ $$ \\ $$ $$\mathrm{Let}\:\mathrm{x}=\mathrm{2k}\pi+\mathrm{t}\:\mathrm{with}\:\mathrm{k}\in\mathbb{N}\:\wedge\:\mathrm{t}\in\left[\mathrm{0},\mathrm{2}\pi\right] \\ $$ $$\mathrm{sin}\:\mathrm{x}=\mathrm{sin}\:\mathrm{t} \\ $$ $$\mathrm{cos}\:\mathrm{x}=\mathrm{cos}\:\mathrm{t} \\ $$ $$ \\ $$ $$\mathrm{we}\:\mathrm{find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{on}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{sin}\:\mathrm{x}\:\mathrm{where}\:\mathrm{its} \\ $$ $$\mathrm{tangent}\:\mathrm{is}\:\mathrm{g}\left(\mathrm{x}\right)=\frac{\mathrm{x}}{\mathrm{n}}. \\ $$ $$\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{cos}\:\mathrm{x}=\mathrm{cos}\:\mathrm{t} \\ $$ $$\mathrm{g}'\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{n}} \\ $$ $$\mathrm{cos}\:\mathrm{t}=\frac{\mathrm{1}}{\mathrm{n}} \\ $$ $$\mathrm{t}=\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{n}} \\ $$ $$\mathrm{sin}\:\mathrm{t}=\frac{\mathrm{n}}{\sqrt{\mathrm{n}^{\mathrm{2}} +\mathrm{1}}} \\ $$ $$ \\ $$ $$\mathrm{so}\:\mathrm{that}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{intersects}\:\mathrm{with}\:\mathrm{g}\left(\mathrm{x}\right), \\ $$ $$\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\geqslant\frac{\mathrm{1}}{\mathrm{n}} \\ $$ $$\Rightarrow\mathrm{n}\:\mathrm{sin}\:\mathrm{x}\geqslant\mathrm{x} \\ $$ $$\Rightarrow\mathrm{n}\:\mathrm{sin}\:\mathrm{t}\geqslant\mathrm{2k}\pi+\mathrm{t} \\ $$ $$\Rightarrow\mathrm{k}\leqslant\frac{\mathrm{n}\:\mathrm{sin}\:\mathrm{t}\:−\mathrm{t}}{\mathrm{2}\pi}=\frac{\frac{\mathrm{n}^{\mathrm{2}} }{\sqrt{\mathrm{n}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{n}}}{\mathrm{2}\pi} \\ $$ $$\mathrm{k}_{\mathrm{max}} =\lfloor\frac{\frac{\mathrm{n}^{\mathrm{2}} }{\sqrt{\mathrm{n}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{n}}}{\mathrm{2}\pi}\rfloor \\ $$ $$ \\ $$ $$\mathrm{number}\:\mathrm{of}\:\mathrm{intersecting}\:\mathrm{points}\:\mathrm{is} \\ $$ $$\mathrm{m}=\mathrm{2}×\mathrm{2}\left(\mathrm{k}_{\mathrm{max}} +\mathrm{1}\right)−\mathrm{1}=\mathrm{4k}_{\mathrm{max}} +\mathrm{3} \\ $$ $$ \\ $$ $$\mathrm{for}\:\mathrm{n}=\mathrm{10} \\ $$ $$\mathrm{k}_{\mathrm{max}} =\lfloor\frac{\frac{\mathrm{n}^{\mathrm{2}} }{\sqrt{\mathrm{n}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{n}}}{\mathrm{2}\pi}\rfloor \\ $$ $$=\lfloor\frac{\frac{\mathrm{10}^{\mathrm{2}} }{\sqrt{\mathrm{10}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{10}}}{\mathrm{2}\pi}\rfloor=\lfloor\mathrm{1}.\mathrm{35}\rfloor=\mathrm{1} \\ $$ $$\Rightarrow\mathrm{m}=\mathrm{4}×\mathrm{1}+\mathrm{3}=\mathrm{7} \\ $$ $$ \\ $$ $$\mathrm{for}\:\mathrm{n}=\mathrm{20} \\ $$ $$\mathrm{k}_{\mathrm{max}} =\lfloor\frac{\frac{\mathrm{20}^{\mathrm{2}} }{\sqrt{\mathrm{20}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{20}}}{\mathrm{2}\pi}\rfloor=\lfloor\mathrm{2}.\mathrm{94}\rfloor=\mathrm{2} \\ $$ $$\Rightarrow\mathrm{m}=\mathrm{4}×\mathrm{2}+\mathrm{3}=\mathrm{11} \\ $$
Commented bymrW1 last updated on 25/Jun/17
Commented byajfour last updated on 25/Jun/17
$$\mathrm{good}\:\mathrm{Sir},\:\mathrm{and}\:\mathrm{thank}\:\mathrm{you}. \\ $$
Commented byTinkutara last updated on 25/Jun/17
$$\mathrm{Thanks}\:\mathrm{Sir}!\:\mathrm{Can}\:\mathrm{you}\:\mathrm{give}\:\mathrm{me}\:\mathrm{your} \\ $$ $$\mathrm{Geometry}\:\mathrm{Expressions}\:\mathrm{for}\:\mathrm{PC}? \\ $$
Commented bymrW1 last updated on 25/Jun/17

$$\mathrm{As}\:\mathrm{I}\:\mathrm{have}\:\mathrm{told}\:\mathrm{you},\:\mathrm{you}\:\mathrm{can}\:\mathrm{download} \\ $$ $$\mathrm{the}\:\mathrm{software}\:\mathrm{in}\:\mathrm{internet}\:\mathrm{by}\:\mathrm{yourself}.\:\mathrm{E}.\mathrm{g}. \\ $$ $$\mathrm{https}://\mathrm{dfiles}.\mathrm{eu}/\mathrm{files}/\mathrm{kd976lf6v} \\ $$ $$\mathrm{It}\:\mathrm{is}\:\mathrm{Russian},\:\mathrm{but}\:\mathrm{you}\:\mathrm{can}\:\mathrm{switch}\:\mathrm{into} \\ $$ $$\mathrm{English}.\:\mathrm{I}\:\mathrm{downloaded}\:\mathrm{it}\:\mathrm{also}\:\mathrm{here}. \\ $$
Commented byTinkutara last updated on 25/Jun/17

$$\mathrm{Sir},\:\mathrm{this}\:\mathrm{is}\:\mathrm{a}\:.\mathrm{rar}\:\mathrm{file}\:\mathrm{and}\:\mathrm{after} \\ $$ $$\mathrm{decompressing}\:\mathrm{it}\:\mathrm{I}\:\mathrm{cannot}\:\mathrm{find}\:\mathrm{the}\:.\mathrm{exe} \\ $$ $$\mathrm{file}\:\mathrm{to}\:\mathrm{be}\:\mathrm{run}\:\mathrm{on}\:\mathrm{PC}.\:\mathrm{What}\:\mathrm{to}\:\mathrm{do}\:\mathrm{after} \\ $$ $$\mathrm{downloading}?\:\mathrm{Will}\:\mathrm{the}\:\mathrm{application}\:\mathrm{not} \\ $$ $$\mathrm{ask}\:\mathrm{for}\:\mathrm{any}\:\mathrm{type}\:\mathrm{of}\:\mathrm{license}\:\mathrm{or}\:\mathrm{key}? \\ $$
Commented bymrW1 last updated on 25/Jun/17

$$\mathrm{In}\:\mathrm{the}\:\mathrm{rar}\:\mathrm{file}\:\mathrm{there}\:\mathrm{are}\:\mathrm{2}\:\mathrm{files}:\:\mathrm{a}\:.\mathrm{exe} \\ $$ $$\mathrm{and}\:\mathrm{a}\:.\mathrm{url}\:\mathrm{file}.\:\mathrm{You}\:\mathrm{can}\:\mathrm{run}\:\mathrm{the}\:\mathrm{file} \\ $$ $$\mathrm{geometryexpressions}.\mathrm{exe}\:\mathrm{directly}. \\ $$
Commented bymrW1 last updated on 25/Jun/17

$$\mathrm{You}\:\mathrm{asked}\:\mathrm{for}\:\mathrm{a}\:\mathrm{version}\:\mathrm{which}\:\mathrm{runs} \\ $$ $$\mathrm{without}\:\mathrm{key}.\:\mathrm{This}\:\mathrm{is}\:\mathrm{such}\:\mathrm{a}\:\mathrm{version}. \\ $$
Commented byTinkutara last updated on 25/Jun/17

$$\mathrm{Thanks}\:\mathrm{a}\:\mathrm{lot}\:\mathrm{Sir}! \\ $$
