
Question Number 16600 by tawa tawa last updated on 24/Jun/17

$$\mathrm{Solve}\:\mathrm{simultaneously} \\ $$$$\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{y}^{\mathrm{2}} \:=\:\mathrm{61}\:\:\:\:\:\:\:\:\:..............\:\mathrm{equation}\:\left(\mathrm{i}\right) \\ $$$$\mathrm{x}^{\mathrm{3}} \:−\:\mathrm{y}^{\mathrm{3}} \:=\:\mathrm{91}\:\:\:\:\:\:\:\:\:..............\:\mathrm{equation}\:\left(\mathrm{ii}\right) \\ $$
Commented by prakash jain last updated on 24/Jun/17

$${x}=\sqrt{\mathrm{61}}\mathrm{cos}\:{u},{y}=\sqrt{\mathrm{61}}\mathrm{sin}\:{u} \\ $$$$\mathrm{61}^{\mathrm{3}/\mathrm{2}} \mathrm{cos}^{\mathrm{3}} {u}−\mathrm{61}^{\mathrm{3}/\mathrm{2}} \mathrm{sin}^{\mathrm{3}} {u}=\mathrm{91} \\ $$$$\left(\mathrm{cos}\:{u}−\mathrm{sin}\:{u}\right)\left(\mathrm{cos}^{\mathrm{2}} {u}+\mathrm{sin}^{\mathrm{2}} {u}−\mathrm{cos}\:{u}\mathrm{sin}\:{u}\right) \\ $$$$\:\:\:\:\:\:\:=\frac{\mathrm{91}}{\mathrm{61}^{\mathrm{3}/\mathrm{2}} } \\ $$$$\left(\mathrm{cos}\:{u}−\mathrm{sin}\:{u}\right)\left(\mathrm{1}−\mathrm{cos}\:{u}\mathrm{sin}\:{u}\right)=.\mathrm{191} \\ $$$$\mathrm{cos}\:\:\left(\frac{\pi}{\mathrm{4}}−{u}\right)\left(\mathrm{2}−\mathrm{sin}\:\mathrm{2}{u}\right)=.\mathrm{191}×\mathrm{2}\sqrt{\mathrm{2}}=.\mathrm{54} \\ $$$$\mathrm{2cos}\:\left(\frac{\pi}{\mathrm{4}}−{u}\right)−\mathrm{cos}\:\left(\frac{\pi}{\mathrm{4}}−{u}\right)\mathrm{sin}\:\mathrm{2}{u}=.\mathrm{54} \\ $$$${continue} \\ $$
Commented by tawa tawa last updated on 24/Jun/17

$$\mathrm{Am}\:\mathrm{with}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by Tinkutara last updated on 24/Jun/17

$$\mathrm{Hit}\:\mathrm{and}\:\mathrm{trial}\:\mathrm{method}: \\ $$$${y}^{\mathrm{2}} \:=\:\mathrm{61}\:−\:{x}^{\mathrm{2}} \\ $$$$\mathrm{Let}\:{x},\:{y}\:\in\:{N}. \\ $$$$\mathrm{Then}\:{y}^{\mathrm{2}} \:=\:\mathrm{60},\:\mathrm{57},\:\mathrm{52},\:\mathrm{45},\:\mathrm{36},\:\mathrm{25},\:\mathrm{12}. \\ $$$$\mathrm{But}\:{x}\:>\:{y}.\:\mathrm{So}\:{x}\:=\:\mathrm{6}\:\mathrm{and}\:{y}\:=\:\mathrm{5}. \\ $$
Commented by tawa tawa last updated on 24/Jun/17

$$\mathrm{Am}\:\mathrm{with}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 26/Jun/17

Commented by mrW1 last updated on 26/Jun/17

$$\mathrm{geogebra}\:\mathrm{is}\:\mathrm{very}\:\mathrm{powerful}! \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 26/Jun/17

$${please}\:{correct}\:{your}\:{final}\:{answer} \\ $$$${from}\::\left(\mathrm{6},−\mathrm{5}\right)\Rightarrow\left(\mathrm{6},\mathrm{5}\right).{cxcuse}\:{me}. \\ $$
Commented by mrW1 last updated on 26/Jun/17

$$\mathrm{certainly}\:\mathrm{you}\:\mathrm{are}\:\mathrm{right}.\:\mathrm{thanks}! \\ $$
Answered by mrW1 last updated on 26/Jun/17
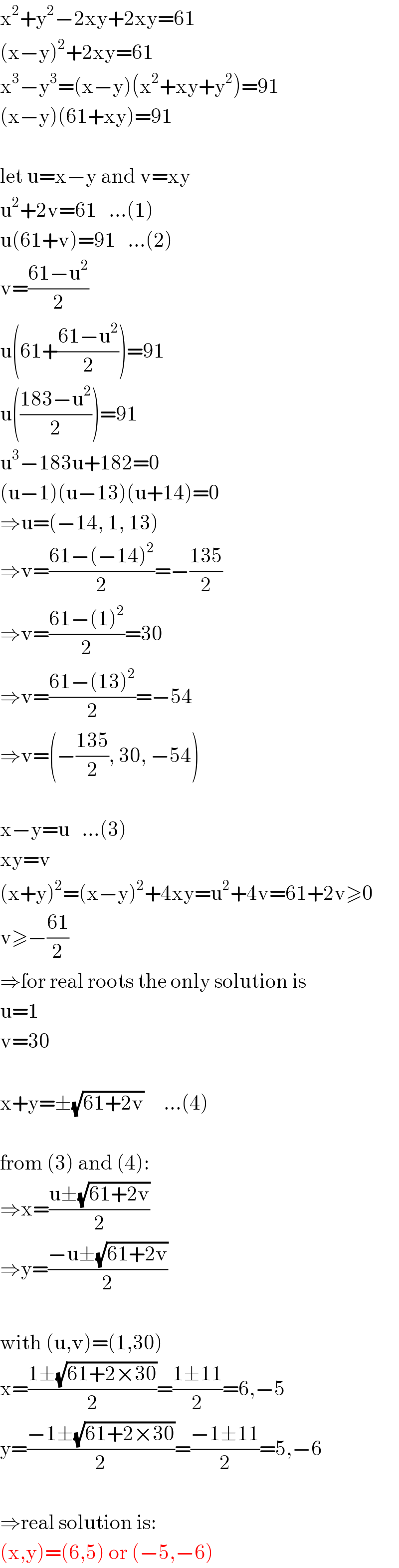
$$\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} −\mathrm{2xy}+\mathrm{2xy}=\mathrm{61} \\ $$$$\left(\mathrm{x}−\mathrm{y}\right)^{\mathrm{2}} +\mathrm{2xy}=\mathrm{61} \\ $$$$\mathrm{x}^{\mathrm{3}} −\mathrm{y}^{\mathrm{3}} =\left(\mathrm{x}−\mathrm{y}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{xy}+\mathrm{y}^{\mathrm{2}} \right)=\mathrm{91} \\ $$$$\left(\mathrm{x}−\mathrm{y}\right)\left(\mathrm{61}+\mathrm{xy}\right)=\mathrm{91} \\ $$$$ \\ $$$$\mathrm{let}\:\mathrm{u}=\mathrm{x}−\mathrm{y}\:\mathrm{and}\:\mathrm{v}=\mathrm{xy} \\ $$$$\mathrm{u}^{\mathrm{2}} +\mathrm{2v}=\mathrm{61}\:\:\:...\left(\mathrm{1}\right) \\ $$$$\mathrm{u}\left(\mathrm{61}+\mathrm{v}\right)=\mathrm{91}\:\:\:...\left(\mathrm{2}\right) \\ $$$$\mathrm{v}=\frac{\mathrm{61}−\mathrm{u}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\mathrm{u}\left(\mathrm{61}+\frac{\mathrm{61}−\mathrm{u}^{\mathrm{2}} }{\mathrm{2}}\right)=\mathrm{91} \\ $$$$\mathrm{u}\left(\frac{\mathrm{183}−\mathrm{u}^{\mathrm{2}} }{\mathrm{2}}\right)=\mathrm{91} \\ $$$$\mathrm{u}^{\mathrm{3}} −\mathrm{183u}+\mathrm{182}=\mathrm{0} \\ $$$$\left(\mathrm{u}−\mathrm{1}\right)\left(\mathrm{u}−\mathrm{13}\right)\left(\mathrm{u}+\mathrm{14}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{u}=\left(−\mathrm{14},\:\mathrm{1},\:\mathrm{13}\right) \\ $$$$\Rightarrow\mathrm{v}=\frac{\mathrm{61}−\left(−\mathrm{14}\right)^{\mathrm{2}} }{\mathrm{2}}=−\frac{\mathrm{135}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{v}=\frac{\mathrm{61}−\left(\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}}=\mathrm{30} \\ $$$$\Rightarrow\mathrm{v}=\frac{\mathrm{61}−\left(\mathrm{13}\right)^{\mathrm{2}} }{\mathrm{2}}=−\mathrm{54} \\ $$$$\Rightarrow\mathrm{v}=\left(−\frac{\mathrm{135}}{\mathrm{2}},\:\mathrm{30},\:−\mathrm{54}\right) \\ $$$$ \\ $$$$\mathrm{x}−\mathrm{y}=\mathrm{u}\:\:\:...\left(\mathrm{3}\right) \\ $$$$\mathrm{xy}=\mathrm{v} \\ $$$$\left(\mathrm{x}+\mathrm{y}\right)^{\mathrm{2}} =\left(\mathrm{x}−\mathrm{y}\right)^{\mathrm{2}} +\mathrm{4xy}=\mathrm{u}^{\mathrm{2}} +\mathrm{4v}=\mathrm{61}+\mathrm{2v}\geqslant\mathrm{0} \\ $$$$\mathrm{v}\geqslant−\frac{\mathrm{61}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{for}\:\mathrm{real}\:\mathrm{roots}\:\mathrm{the}\:\mathrm{only}\:\mathrm{solution}\:\mathrm{is}\: \\ $$$$\mathrm{u}=\mathrm{1} \\ $$$$\mathrm{v}=\mathrm{30} \\ $$$$ \\ $$$$\mathrm{x}+\mathrm{y}=\pm\sqrt{\mathrm{61}+\mathrm{2v}}\:\:\:\:\:...\left(\mathrm{4}\right) \\ $$$$ \\ $$$$\mathrm{from}\:\left(\mathrm{3}\right)\:\mathrm{and}\:\left(\mathrm{4}\right): \\ $$$$\Rightarrow\mathrm{x}=\frac{\mathrm{u}\pm\sqrt{\mathrm{61}+\mathrm{2v}}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{y}=\frac{−\mathrm{u}\pm\sqrt{\mathrm{61}+\mathrm{2v}}}{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{with}\:\left(\mathrm{u},\mathrm{v}\right)=\left(\mathrm{1},\mathrm{30}\right) \\ $$$$\mathrm{x}=\frac{\mathrm{1}\pm\sqrt{\mathrm{61}+\mathrm{2}×\mathrm{30}}}{\mathrm{2}}=\frac{\mathrm{1}\pm\mathrm{11}}{\mathrm{2}}=\mathrm{6},−\mathrm{5} \\ $$$$\mathrm{y}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{61}+\mathrm{2}×\mathrm{30}}}{\mathrm{2}}=\frac{−\mathrm{1}\pm\mathrm{11}}{\mathrm{2}}=\mathrm{5},−\mathrm{6} \\ $$$$ \\ $$$$\Rightarrow\mathrm{real}\:\mathrm{solution}\:\mathrm{is}: \\ $$$$\left(\mathrm{x},\mathrm{y}\right)=\left(\mathrm{6},\mathrm{5}\right)\:\mathrm{or}\:\left(−\mathrm{5},−\mathrm{6}\right) \\ $$
Commented by tawa tawa last updated on 24/Jun/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}.\: \\ $$
Commented by tawa tawa last updated on 24/Jun/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}.\: \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 25/Jun/17
$${perfect}!\:\&\:{cxcellent}. \\ $$
Commented by RasheedSoomro last updated on 25/Jun/17

$$\mathrm{e}^{\mathrm{x}} \mathrm{cellent}! \\ $$
