
Question Number 158143 by alcohol last updated on 31/Oct/21

Commented by TheHoneyCat last updated on 31/Oct/21
![G_3 and G_2 are clearly isomorphical buy looking at their respectiv tables. we just need to map as follow: { (1,⇆,1),(5,⇆,3),(7,⇆,5),((11),⇆,7) :} G_1 cannot be isomorphic to either one because 3×_(10) 3=9≢1 [10] while ∀n∈{1,5,7,11} n×_(12) n=1 and ∀n∈{1,3,5,7} n×_8 n=1](Q158168.png)
$$\mathrm{G}_{\mathrm{3}} \:\mathrm{and}\:\mathrm{G}_{\mathrm{2}} \:\mathrm{are}\:\mathrm{clearly}\:\mathrm{isomorphical}\:\mathrm{buy}\:\mathrm{looking} \\ $$$$\mathrm{at}\:\mathrm{their}\:\mathrm{respectiv}\:\mathrm{tables}. \\ $$$$\mathrm{we}\:\mathrm{just}\:\mathrm{need}\:\mathrm{to}\:\mathrm{map}\:\mathrm{as}\:\mathrm{follow}: \\ $$$$\begin{cases}{\mathrm{1}}&{\leftrightarrows}&{\mathrm{1}}\\{\mathrm{5}}&{\leftrightarrows}&{\mathrm{3}}\\{\mathrm{7}}&{\leftrightarrows}&{\mathrm{5}}\\{\mathrm{11}}&{\leftrightarrows}&{\mathrm{7}}\end{cases} \\ $$$$ \\ $$$$\mathrm{G}_{\mathrm{1}} \:\mathrm{cannot}\:\mathrm{be}\:\mathrm{isomorphic}\:\mathrm{to}\:\mathrm{either}\:\mathrm{one}\:\mathrm{because} \\ $$$$\mathrm{3}×_{\mathrm{10}} \mathrm{3}=\mathrm{9}≢\mathrm{1}\:\left[\mathrm{10}\right] \\ $$$$\mathrm{while}\:\forall{n}\in\left\{\mathrm{1},\mathrm{5},\mathrm{7},\mathrm{11}\right\}\:{n}×_{\mathrm{12}} {n}=\mathrm{1} \\ $$$$\mathrm{and}\:\forall{n}\in\left\{\mathrm{1},\mathrm{3},\mathrm{5},\mathrm{7}\right\}\:{n}×_{\mathrm{8}} {n}=\mathrm{1} \\ $$
Commented by TheHoneyCat last updated on 31/Oct/21
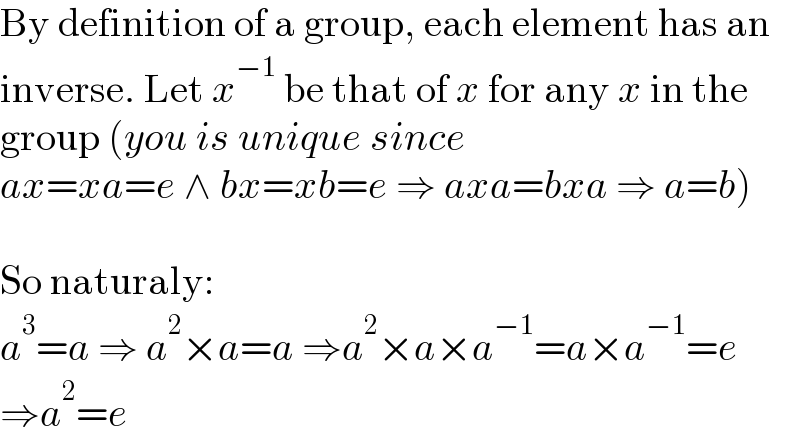
$$\mathrm{By}\:\mathrm{definition}\:\mathrm{of}\:\mathrm{a}\:\mathrm{group},\:\mathrm{each}\:\mathrm{element}\:\mathrm{has}\:\mathrm{an} \\ $$$$\mathrm{inverse}.\:\mathrm{Let}\:{x}^{−\mathrm{1}} \:\mathrm{be}\:\mathrm{that}\:\mathrm{of}\:{x}\:\mathrm{for}\:\mathrm{any}\:{x}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{group}\:\left({you}\:{is}\:{unique}\:{since}\right. \\ $$$$\left.{ax}={xa}={e}\:\wedge\:{bx}={xb}={e}\:\Rightarrow\:{axa}={bxa}\:\Rightarrow\:{a}={b}\right) \\ $$$$ \\ $$$$\mathrm{So}\:\mathrm{naturaly}:\: \\ $$$${a}^{\mathrm{3}} ={a}\:\Rightarrow\:{a}^{\mathrm{2}} ×{a}={a}\:\Rightarrow{a}^{\mathrm{2}} ×{a}×{a}^{−\mathrm{1}} ={a}×{a}^{−\mathrm{1}} ={e} \\ $$$$\Rightarrow{a}^{\mathrm{2}} ={e} \\ $$
Commented by TheHoneyCat last updated on 31/Oct/21
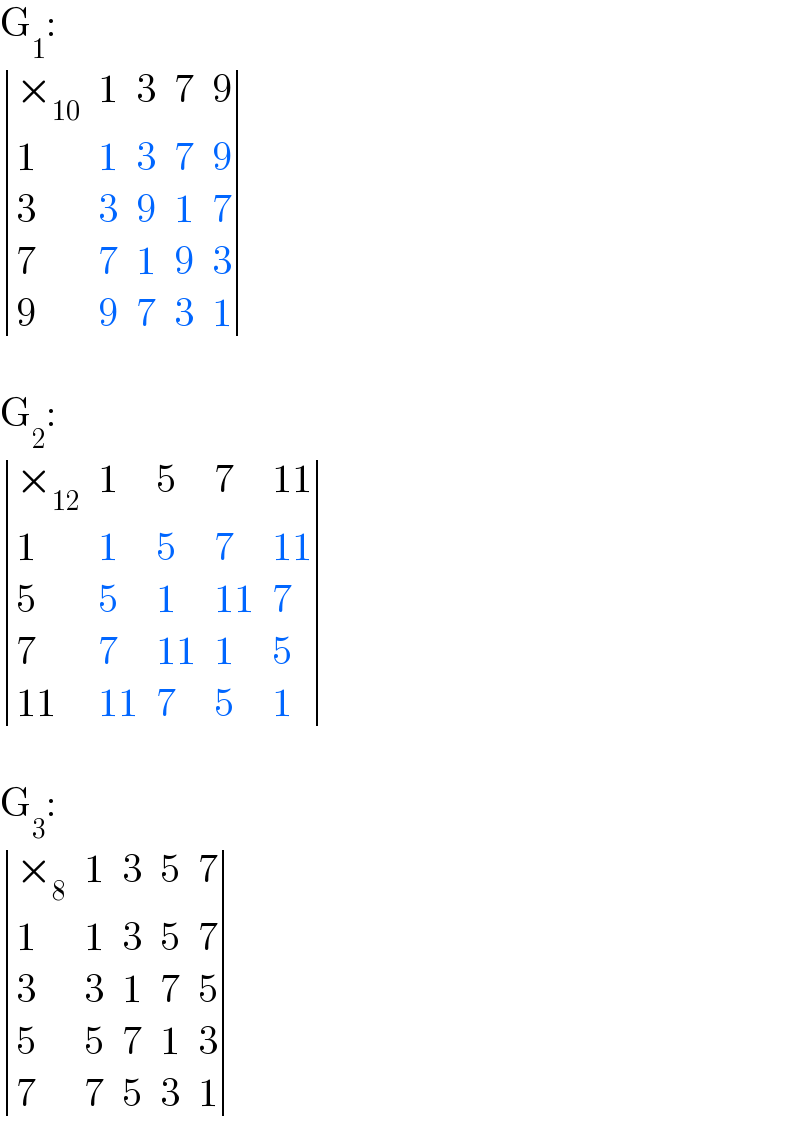
$$\mathrm{G}_{\mathrm{1}} : \\ $$$$\begin{vmatrix}{×_{\mathrm{10}} }&{\mathrm{1}}&{\mathrm{3}}&{\mathrm{7}}&{\mathrm{9}}\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{3}}&{\mathrm{7}}&{\mathrm{9}}\\{\mathrm{3}}&{\mathrm{3}}&{\mathrm{9}}&{\mathrm{1}}&{\mathrm{7}}\\{\mathrm{7}}&{\mathrm{7}}&{\mathrm{1}}&{\mathrm{9}}&{\mathrm{3}}\\{\mathrm{9}}&{\mathrm{9}}&{\mathrm{7}}&{\mathrm{3}}&{\mathrm{1}}\end{vmatrix} \\ $$$$ \\ $$$$\mathrm{G}_{\mathrm{2}} : \\ $$$$\begin{vmatrix}{×_{\mathrm{12}} }&{\mathrm{1}}&{\mathrm{5}}&{\mathrm{7}}&{\mathrm{11}}\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{5}}&{\mathrm{7}}&{\mathrm{11}}\\{\mathrm{5}}&{\mathrm{5}}&{\mathrm{1}}&{\mathrm{11}}&{\mathrm{7}}\\{\mathrm{7}}&{\mathrm{7}}&{\mathrm{11}}&{\mathrm{1}}&{\mathrm{5}}\\{\mathrm{11}}&{\mathrm{11}}&{\mathrm{7}}&{\mathrm{5}}&{\mathrm{1}}\end{vmatrix} \\ $$$$ \\ $$$$\mathrm{G}_{\mathrm{3}} : \\ $$$$\begin{vmatrix}{×_{\mathrm{8}} }&{\mathrm{1}}&{\mathrm{3}}&{\mathrm{5}}&{\mathrm{7}}\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{3}}&{\mathrm{5}}&{\mathrm{7}}\\{\mathrm{3}}&{\mathrm{3}}&{\mathrm{1}}&{\mathrm{7}}&{\mathrm{5}}\\{\mathrm{5}}&{\mathrm{5}}&{\mathrm{7}}&{\mathrm{1}}&{\mathrm{3}}\\{\mathrm{7}}&{\mathrm{7}}&{\mathrm{5}}&{\mathrm{3}}&{\mathrm{1}}\end{vmatrix} \\ $$
Commented by TheHoneyCat last updated on 31/Oct/21
oups, I forgot to put the last one in blue...
Commented by phanphuoc last updated on 31/Oct/21
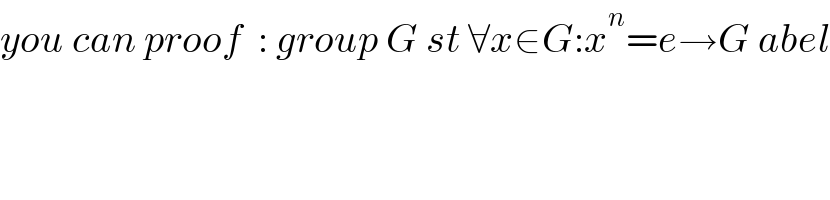
$${you}\:{can}\:{proof}\:\::\:{group}\:{G}\:{st}\:\forall{x}\in{G}:{x}^{{n}} ={e}\rightarrow{G}\:{abel} \\ $$
Commented by TheHoneyCat last updated on 31/Oct/21
it did say "∀x" but "∃a" so I just did not assume anything
Answered by TheHoneyCat last updated on 31/Oct/21
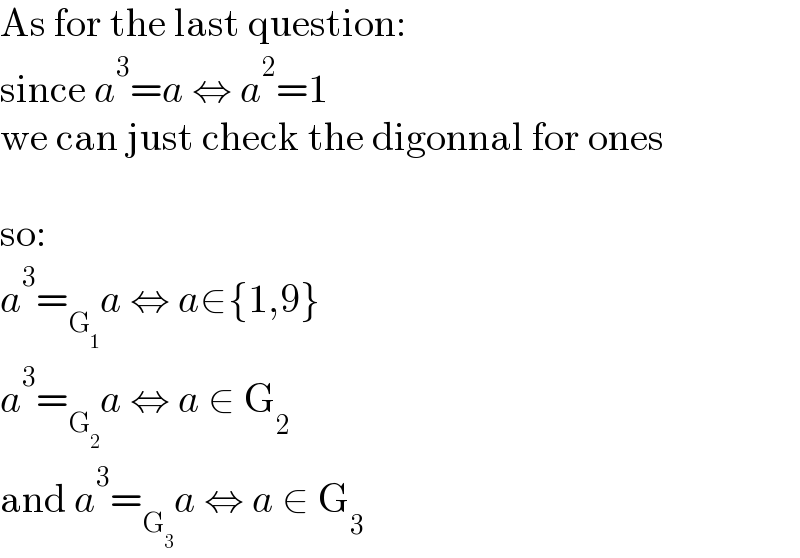
$$\mathrm{As}\:\mathrm{for}\:\mathrm{the}\:\mathrm{last}\:\mathrm{question}: \\ $$$$\mathrm{since}\:{a}^{\mathrm{3}} ={a}\:\Leftrightarrow\:{a}^{\mathrm{2}} =\mathrm{1} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{just}\:\mathrm{check}\:\mathrm{the}\:\mathrm{digonnal}\:\mathrm{for}\:\mathrm{ones} \\ $$$$ \\ $$$$\mathrm{so}: \\ $$$${a}^{\mathrm{3}} =_{\mathrm{G}_{\mathrm{1}} } {a}\:\Leftrightarrow\:{a}\in\left\{\mathrm{1},\mathrm{9}\right\} \\ $$$${a}^{\mathrm{3}} =_{\mathrm{G}_{\mathrm{2}} } {a}\:\Leftrightarrow\:{a}\:\in\:\mathrm{G}_{\mathrm{2}} \\ $$$$\mathrm{and}\:{a}^{\mathrm{3}} =_{\mathrm{G}_{\mathrm{3}} } {a}\:\Leftrightarrow\:{a}\:\in\:\mathrm{G}_{\mathrm{3}} \\ $$
