
Question Number 154476 by SANOGO last updated on 18/Sep/21
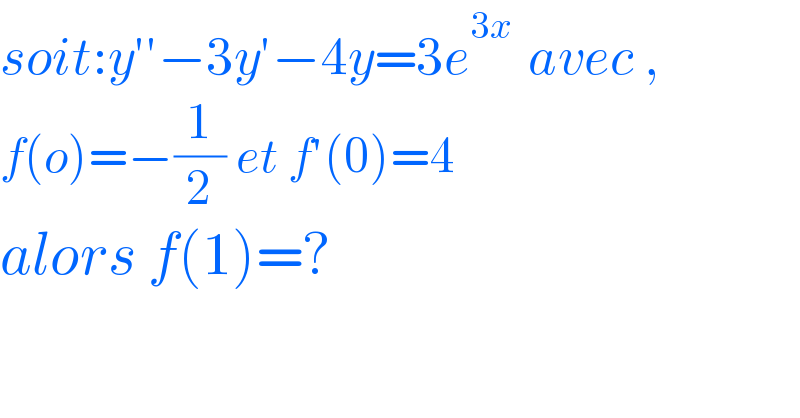
$${soit}:{y}''−\mathrm{3}{y}'−\mathrm{4}{y}=\mathrm{3}{e}^{\mathrm{3}{x}\:} \:{avec}\:, \\ $$$${f}\left({o}\right)=−\frac{\mathrm{1}}{\mathrm{2}}\:{et}\:{f}'\left(\mathrm{0}\right)=\mathrm{4} \\ $$$${alors}\:{f}\left(\mathrm{1}\right)=? \\ $$$$ \\ $$
Answered by ARUNG_Brandon_MBU last updated on 18/Sep/21
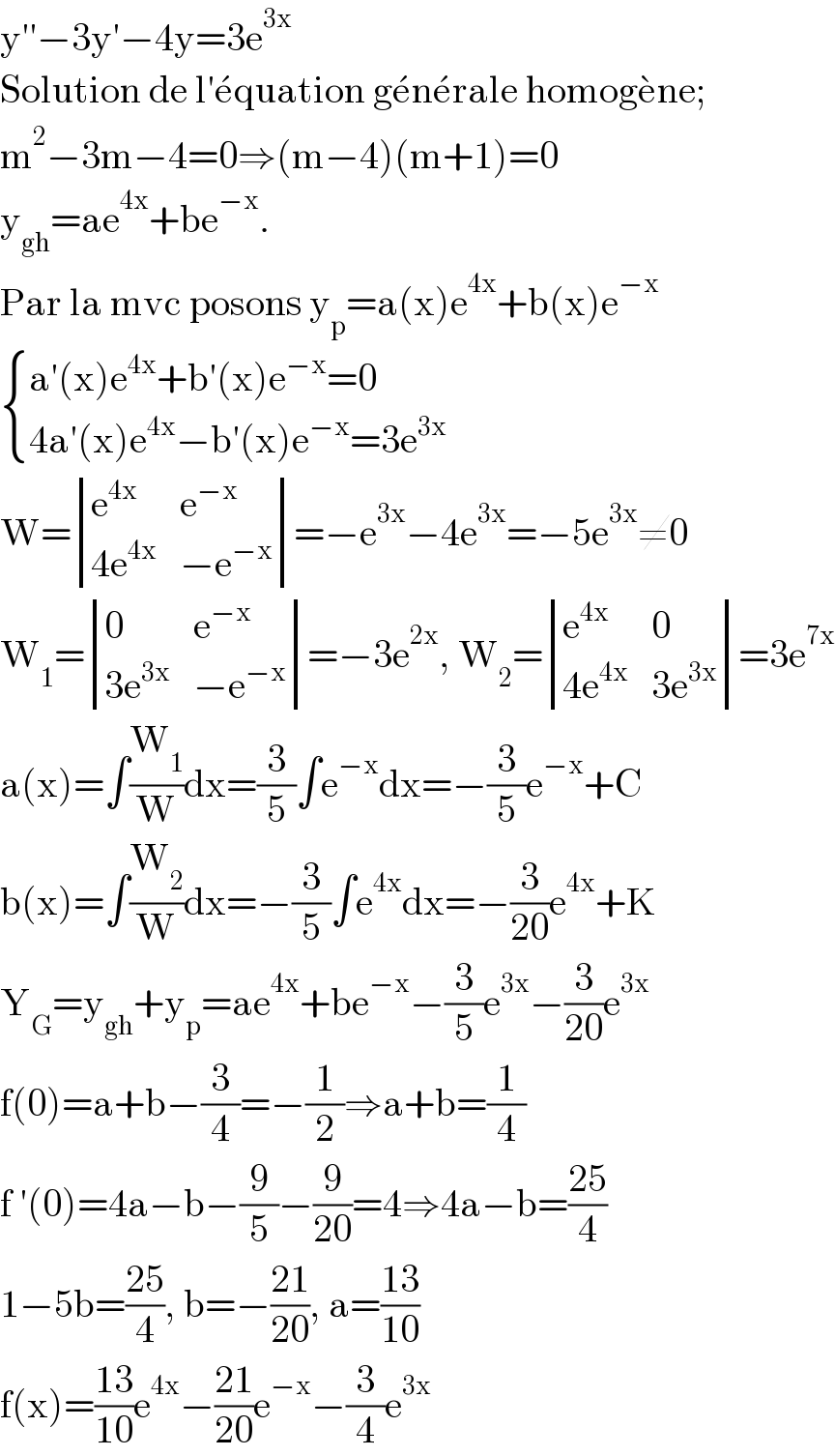
$$\mathrm{y}''−\mathrm{3y}'−\mathrm{4y}=\mathrm{3e}^{\mathrm{3x}} \\ $$$$\mathrm{Solution}\:\mathrm{de}\:\mathrm{l}'\acute {\mathrm{e}quation}\:\mathrm{g}\acute {\mathrm{e}n}\acute {\mathrm{e}rale}\:\mathrm{homog}\grave {\mathrm{e}ne}; \\ $$$$\mathrm{m}^{\mathrm{2}} −\mathrm{3m}−\mathrm{4}=\mathrm{0}\Rightarrow\left(\mathrm{m}−\mathrm{4}\right)\left(\mathrm{m}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\mathrm{y}_{\mathrm{gh}} =\mathrm{ae}^{\mathrm{4x}} +\mathrm{be}^{−\mathrm{x}} . \\ $$$$\mathrm{Par}\:\mathrm{la}\:\mathrm{mvc}\:\mathrm{posons}\:\mathrm{y}_{\mathrm{p}} =\mathrm{a}\left(\mathrm{x}\right)\mathrm{e}^{\mathrm{4x}} +\mathrm{b}\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{x}} \\ $$$$\begin{cases}{\mathrm{a}'\left(\mathrm{x}\right)\mathrm{e}^{\mathrm{4x}} +\mathrm{b}'\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{x}} =\mathrm{0}}\\{\mathrm{4a}'\left(\mathrm{x}\right)\mathrm{e}^{\mathrm{4x}} −\mathrm{b}'\left(\mathrm{x}\right)\mathrm{e}^{−\mathrm{x}} =\mathrm{3e}^{\mathrm{3x}} }\end{cases} \\ $$$$\mathrm{W}=\begin{vmatrix}{\mathrm{e}^{\mathrm{4x}} }&{\mathrm{e}^{−\mathrm{x}} }\\{\mathrm{4e}^{\mathrm{4x}} }&{−\mathrm{e}^{−\mathrm{x}} }\end{vmatrix}=−\mathrm{e}^{\mathrm{3x}} −\mathrm{4e}^{\mathrm{3x}} =−\mathrm{5e}^{\mathrm{3x}} \neq\mathrm{0} \\ $$$$\mathrm{W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{0}}&{\mathrm{e}^{−\mathrm{x}} }\\{\mathrm{3e}^{\mathrm{3x}} }&{−\mathrm{e}^{−\mathrm{x}} }\end{vmatrix}=−\mathrm{3e}^{\mathrm{2x}} ,\:\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{e}^{\mathrm{4x}} }&{\mathrm{0}}\\{\mathrm{4e}^{\mathrm{4x}} }&{\mathrm{3e}^{\mathrm{3x}} }\end{vmatrix}=\mathrm{3e}^{\mathrm{7x}} \\ $$$$\mathrm{a}\left(\mathrm{x}\right)=\int\frac{\mathrm{W}_{\mathrm{1}} }{\mathrm{W}}\mathrm{dx}=\frac{\mathrm{3}}{\mathrm{5}}\int\mathrm{e}^{−\mathrm{x}} \mathrm{dx}=−\frac{\mathrm{3}}{\mathrm{5}}\mathrm{e}^{−\mathrm{x}} +\mathrm{C} \\ $$$$\mathrm{b}\left(\mathrm{x}\right)=\int\frac{\mathrm{W}_{\mathrm{2}} }{\mathrm{W}}\mathrm{dx}=−\frac{\mathrm{3}}{\mathrm{5}}\int\mathrm{e}^{\mathrm{4x}} \mathrm{dx}=−\frac{\mathrm{3}}{\mathrm{20}}\mathrm{e}^{\mathrm{4x}} +\mathrm{K} \\ $$$$\mathrm{Y}_{\mathrm{G}} =\mathrm{y}_{\mathrm{gh}} +\mathrm{y}_{\mathrm{p}} =\mathrm{ae}^{\mathrm{4x}} +\mathrm{be}^{−\mathrm{x}} −\frac{\mathrm{3}}{\mathrm{5}}\mathrm{e}^{\mathrm{3x}} −\frac{\mathrm{3}}{\mathrm{20}}\mathrm{e}^{\mathrm{3x}} \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=\mathrm{a}+\mathrm{b}−\frac{\mathrm{3}}{\mathrm{4}}=−\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\mathrm{a}+\mathrm{b}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\mathrm{f}\:'\left(\mathrm{0}\right)=\mathrm{4a}−\mathrm{b}−\frac{\mathrm{9}}{\mathrm{5}}−\frac{\mathrm{9}}{\mathrm{20}}=\mathrm{4}\Rightarrow\mathrm{4a}−\mathrm{b}=\frac{\mathrm{25}}{\mathrm{4}} \\ $$$$\mathrm{1}−\mathrm{5b}=\frac{\mathrm{25}}{\mathrm{4}},\:\mathrm{b}=−\frac{\mathrm{21}}{\mathrm{20}},\:\mathrm{a}=\frac{\mathrm{13}}{\mathrm{10}} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{13}}{\mathrm{10}}\mathrm{e}^{\mathrm{4x}} −\frac{\mathrm{21}}{\mathrm{20}}\mathrm{e}^{−\mathrm{x}} −\frac{\mathrm{3}}{\mathrm{4}}\mathrm{e}^{\mathrm{3x}} \\ $$
Commented by SANOGO last updated on 18/Sep/21
$${merci}\:{bien}\:{le}\:{dur} \\ $$
Commented by ARUNG_Brandon_MBU last updated on 18/Sep/21
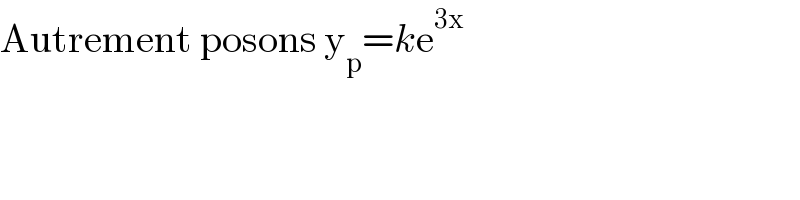
$$\mathrm{Autrement}\:\mathrm{posons}\:\mathrm{y}_{\mathrm{p}} ={k}\mathrm{e}^{\mathrm{3x}} \\ $$
Commented by SANOGO last updated on 18/Sep/21
$${ok}\:{daccord}\:{merci} \\ $$
Commented by Ar Brandon last updated on 18/Sep/21

$$\mathrm{Je}\:\mathrm{vous}\:\mathrm{en}\:\mathrm{prie} \\ $$
Answered by qaz last updated on 19/Sep/21
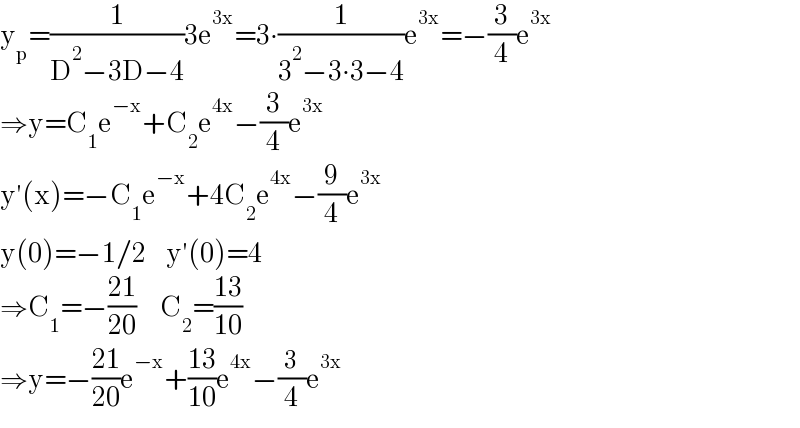
$$\mathrm{y}_{\mathrm{p}} =\frac{\mathrm{1}}{\mathrm{D}^{\mathrm{2}} −\mathrm{3D}−\mathrm{4}}\mathrm{3e}^{\mathrm{3x}} =\mathrm{3}\centerdot\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} −\mathrm{3}\centerdot\mathrm{3}−\mathrm{4}}\mathrm{e}^{\mathrm{3x}} =−\frac{\mathrm{3}}{\mathrm{4}}\mathrm{e}^{\mathrm{3x}} \\ $$$$\Rightarrow\mathrm{y}=\mathrm{C}_{\mathrm{1}} \mathrm{e}^{−\mathrm{x}} +\mathrm{C}_{\mathrm{2}} \mathrm{e}^{\mathrm{4x}} −\frac{\mathrm{3}}{\mathrm{4}}\mathrm{e}^{\mathrm{3x}} \\ $$$$\mathrm{y}'\left(\mathrm{x}\right)=−\mathrm{C}_{\mathrm{1}} \mathrm{e}^{−\mathrm{x}} +\mathrm{4C}_{\mathrm{2}} \mathrm{e}^{\mathrm{4x}} −\frac{\mathrm{9}}{\mathrm{4}}\mathrm{e}^{\mathrm{3x}} \\ $$$$\mathrm{y}\left(\mathrm{0}\right)=−\mathrm{1}/\mathrm{2}\:\:\:\:\:\mathrm{y}'\left(\mathrm{0}\right)=\mathrm{4} \\ $$$$\Rightarrow\mathrm{C}_{\mathrm{1}} =−\frac{\mathrm{21}}{\mathrm{20}}\:\:\:\:\:\:\mathrm{C}_{\mathrm{2}} =\frac{\mathrm{13}}{\mathrm{10}} \\ $$$$\Rightarrow\mathrm{y}=−\frac{\mathrm{21}}{\mathrm{20}}\mathrm{e}^{−\mathrm{x}} +\frac{\mathrm{13}}{\mathrm{10}}\mathrm{e}^{\mathrm{4x}} −\frac{\mathrm{3}}{\mathrm{4}}\mathrm{e}^{\mathrm{3x}} \\ $$
Answered by physicstutes last updated on 20/Sep/21
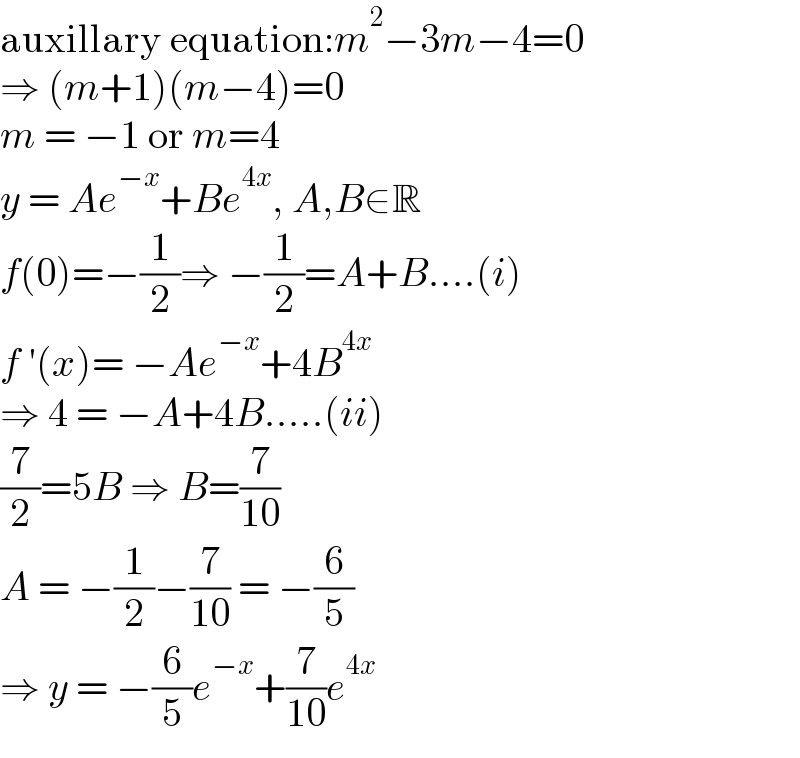
$$\mathrm{auxillary}\:\mathrm{equation}:{m}^{\mathrm{2}} −\mathrm{3}{m}−\mathrm{4}=\mathrm{0} \\ $$$$\Rightarrow\:\left({m}+\mathrm{1}\right)\left({m}−\mathrm{4}\right)=\mathrm{0} \\ $$$${m}\:=\:−\mathrm{1}\:\mathrm{or}\:{m}=\mathrm{4} \\ $$$${y}\:=\:{Ae}^{−{x}} +{Be}^{\mathrm{4}{x}} ,\:{A},{B}\in\mathbb{R} \\ $$$${f}\left(\mathrm{0}\right)=−\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\:−\frac{\mathrm{1}}{\mathrm{2}}={A}+{B}....\left({i}\right) \\ $$$${f}\:'\left({x}\right)=\:−{Ae}^{−{x}} +\mathrm{4}{B}^{\mathrm{4}{x}} \\ $$$$\Rightarrow\:\mathrm{4}\:=\:−{A}+\mathrm{4}{B}.....\left({ii}\right) \\ $$$$\frac{\mathrm{7}}{\mathrm{2}}=\mathrm{5}{B}\:\Rightarrow\:{B}=\frac{\mathrm{7}}{\mathrm{10}} \\ $$$${A}\:=\:−\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{7}}{\mathrm{10}}\:=\:−\frac{\mathrm{6}}{\mathrm{5}} \\ $$$$\Rightarrow\:{y}\:=\:−\frac{\mathrm{6}}{\mathrm{5}}{e}^{−{x}} +\frac{\mathrm{7}}{\mathrm{10}}{e}^{\mathrm{4}{x}} \\ $$
