
Question Number 154343 by Lekhraj last updated on 17/Sep/21
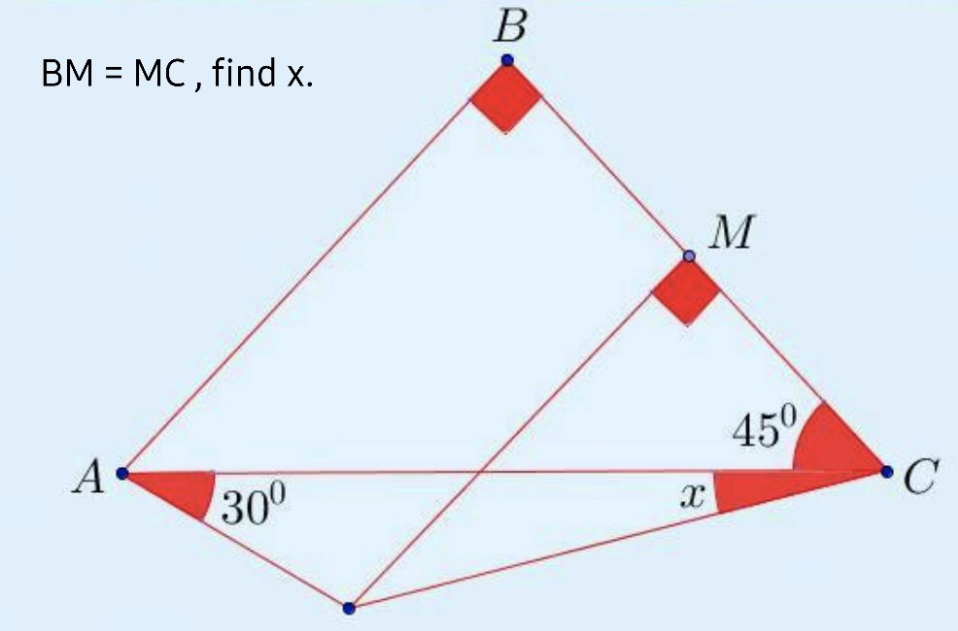
Answered by qaz last updated on 17/Sep/21

$$\frac{\mathrm{sin}\:\mathrm{x}}{\bullet\mathrm{O}}=\frac{\mathrm{sin}\:\angle\mathrm{M}\bullet\mathrm{C}}{\mathrm{OC}},\:\:\:\:\:\:\frac{\mathrm{sin}\:\mathrm{30}°}{\bullet\mathrm{O}}=\frac{\mathrm{sin}\:\mathrm{105}°}{\mathrm{OA}} \\ $$$$\because\:\:\mathrm{OA}=\mathrm{OC}\:\:\:\:\:\: \\ $$$$\therefore\:\:\mathrm{sin}\:\mathrm{x}=\frac{\mathrm{sin}\:\mathrm{30}°}{\mathrm{sin}\:\mathrm{105}°}\centerdot\mathrm{sin}\:\angle\mathrm{M}\bullet\mathrm{C}=\frac{\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{3}}+\mathrm{1}}\mathrm{sin}\:\angle\mathrm{M}\bullet\mathrm{C} \\ $$$$\because\:\:\:\angle\mathrm{M}\bullet\mathrm{C}=\mathrm{180}°−\mathrm{135}°−\mathrm{x}=\mathrm{45}°−\mathrm{x} \\ $$$$\therefore\:\:\:\mathrm{sin}\:\mathrm{x}=\frac{\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{3}}+\mathrm{1}}\mathrm{sin}\:\left(\mathrm{45}°−\mathrm{x}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}+\mathrm{1}}\left(\mathrm{cos}\:\mathrm{x}−\mathrm{sin}\:\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{tan}\:\mathrm{x}=\mathrm{2}−\sqrt{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}−\sqrt{\mathrm{3}}\right) \\ $$
Commented by Lekhraj last updated on 17/Sep/21
$$\:\mathrm{Thank}\:\mathrm{you}\:.\:\mathrm{You}\:\mathrm{does}\:\mathrm{not}\:\mathrm{mark}\:\mathrm{in}\:\mathrm{the}\:\mathrm{figure}\:\mathrm{which}\:\mathrm{point}\:\mathrm{is}\:\mathrm{the}\:\mathrm{blue}\:\mathrm{dot} \\ $$$$\mathrm{and}\:\mathrm{which}\:\mathrm{point}\:\mathrm{is}\:\mathrm{o}. \\ $$
