
Question Number 154318 by liberty last updated on 17/Sep/21
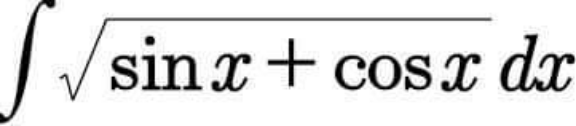
Answered by MJS_new last updated on 17/Sep/21
![sin x +cos x =(√2)sin ((4x+π)/4) = [sin θ =1−2sin^2 ((2θ−π)/4)] =(√2)(1−2sin^2 ((4x−π)/8)) ∫(√(sin x +cos x))dx= [t=((4x−π)/8) → dx=2dt] =2^(5/4) ∫(√(1−2sin^2 t))dt=2^(5/4) E (t∣2) = =2^(5/4) E ((x/2)−(π/8)∣2) +C](Q154333.png)
$$\mathrm{sin}\:{x}\:+\mathrm{cos}\:{x}\:=\sqrt{\mathrm{2}}\mathrm{sin}\:\frac{\mathrm{4}{x}+\pi}{\mathrm{4}}\:= \\ $$$$\:\:\:\:\:\left[\mathrm{sin}\:\theta\:=\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \:\frac{\mathrm{2}\theta−\pi}{\mathrm{4}}\right] \\ $$$$=\sqrt{\mathrm{2}}\left(\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \:\frac{\mathrm{4}{x}−\pi}{\mathrm{8}}\right) \\ $$$$\int\sqrt{\mathrm{sin}\:{x}\:+\mathrm{cos}\:{x}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\frac{\mathrm{4}{x}−\pi}{\mathrm{8}}\:\rightarrow\:{dx}=\mathrm{2}{dt}\right] \\ $$$$=\mathrm{2}^{\mathrm{5}/\mathrm{4}} \int\sqrt{\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \:{t}}{dt}=\mathrm{2}^{\mathrm{5}/\mathrm{4}} \mathrm{E}\:\left({t}\mid\mathrm{2}\right)\:= \\ $$$$=\mathrm{2}^{\mathrm{5}/\mathrm{4}} \mathrm{E}\:\left(\frac{{x}}{\mathrm{2}}−\frac{\pi}{\mathrm{8}}\mid\mathrm{2}\right)\:+{C} \\ $$
