
Question Number 1543 by 112358 last updated on 17/Aug/15
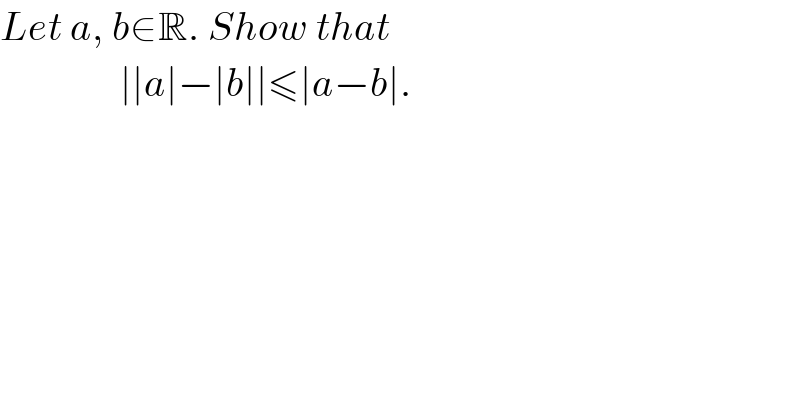
$${Let}\:{a},\:{b}\in\mathbb{R}.\:{Show}\:{that}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mid\mid{a}\mid−\mid{b}\mid\mid\leqslant\mid{a}−{b}\mid. \\ $$
Answered by Rasheed Soomro last updated on 18/Aug/15
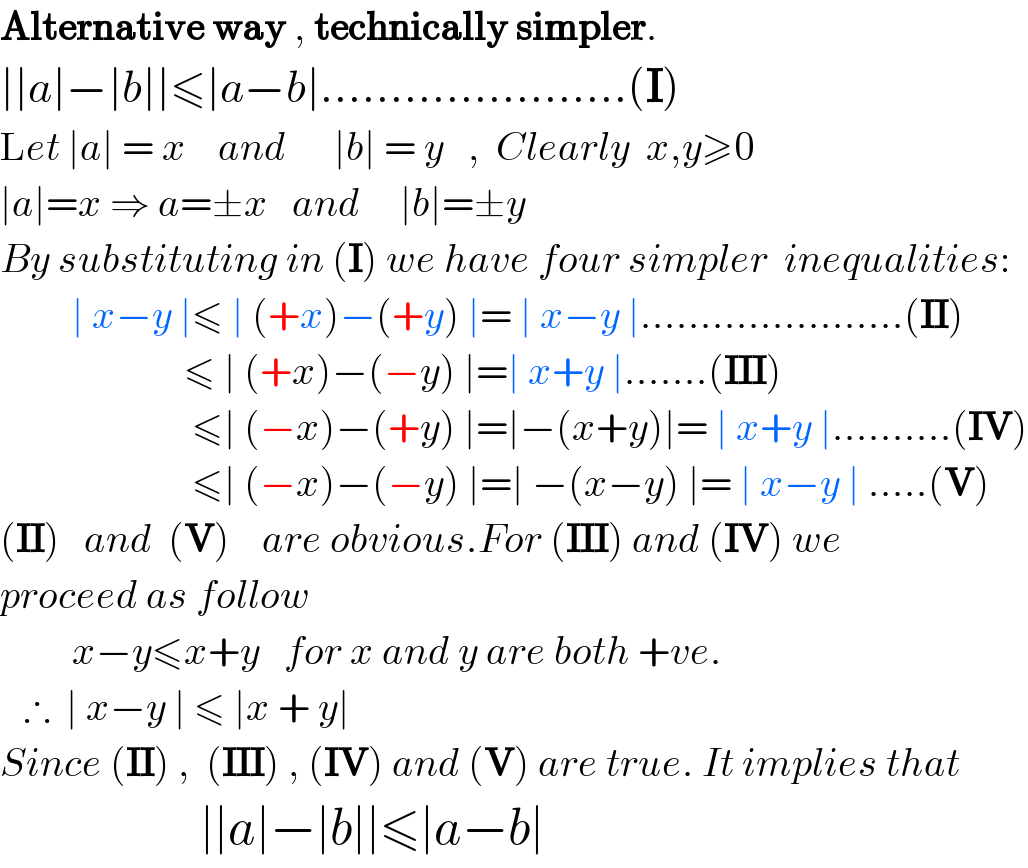
$$\boldsymbol{\mathrm{Alternative}}\:\boldsymbol{\mathrm{way}}\:,\:\boldsymbol{\mathrm{technically}}\:\boldsymbol{\mathrm{simpler}}. \\ $$$$\mid\mid{a}\mid−\mid{b}\mid\mid\leqslant\mid{a}−{b}\mid......................\left(\boldsymbol{\mathrm{I}}\right) \\ $$$$\mathrm{L}{et}\:\mid{a}\mid\:=\:{x}\:\:\:\:{and}\:\:\:\:\:\:\mid{b}\mid\:=\:{y}\:\:\:,\:\:{Clearly}\:\:{x},{y}\geqslant\mathrm{0} \\ $$$$\mid{a}\mid={x}\:\Rightarrow\:{a}=\pm{x}\:\:\:{and}\:\:\:\:\:\mid{b}\mid=\pm{y} \\ $$$${By}\:{substituting}\:{in}\:\left(\boldsymbol{\mathrm{I}}\right)\:{we}\:{have}\:{four}\:{simpler}\:\:{inequalities}: \\ $$$$\:\:\:\:\:\:\:\:\:\mid\:{x}−{y}\:\mid\leqslant\:\mid\:\left(+{x}\right)−\left(+{y}\right)\:\mid=\:\mid\:{x}−{y}\:\mid......................\left(\boldsymbol{\mathrm{II}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\leqslant\:\mid\:\left(+{x}\right)−\left(−{y}\right)\:\mid=\mid\:{x}+{y}\:\mid.......\left(\boldsymbol{\mathrm{III}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\leqslant\mid\:\left(−{x}\right)−\left(+{y}\right)\:\mid=\mid−\left({x}+{y}\right)\mid=\:\mid\:{x}+{y}\:\mid..........\left(\boldsymbol{\mathrm{IV}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\leqslant\mid\:\left(−{x}\right)−\left(−{y}\right)\:\mid=\mid\:−\left({x}−{y}\right)\:\mid=\:\mid\:{x}−{y}\:\mid\:.....\left(\boldsymbol{\mathrm{V}}\right) \\ $$$$\left(\boldsymbol{\mathrm{II}}\right)\:\:\:{and}\:\:\left(\boldsymbol{\mathrm{V}}\right)\:\:\:\:{are}\:{obvious}.{For}\:\left(\boldsymbol{\mathrm{III}}\right)\:{and}\:\left(\boldsymbol{\mathrm{IV}}\right)\:{we} \\ $$$${proceed}\:{as}\:{follow} \\ $$$$\:\:\:\:\:\:\:\:\:{x}−{y}\leqslant{x}+{y}\:\:\:{for}\:{x}\:{and}\:{y}\:{are}\:{both}\:+{ve}. \\ $$$$\:\:\:\therefore\:\:\mid\:{x}−{y}\:\mid\:\leqslant\:\mid{x}\:+\:{y}\mid \\ $$$${Since}\:\left(\boldsymbol{\mathrm{II}}\right)\:,\:\:\left(\boldsymbol{\mathrm{III}}\right)\:,\:\left(\boldsymbol{\mathrm{IV}}\right)\:{and}\:\left(\boldsymbol{\mathrm{V}}\right)\:{are}\:{true}.\:{It}\:{implies}\:{that} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mid\mid{a}\mid−\mid{b}\mid\mid\leqslant\mid{a}−{b}\mid \\ $$
Answered by 123456 last updated on 17/Aug/15
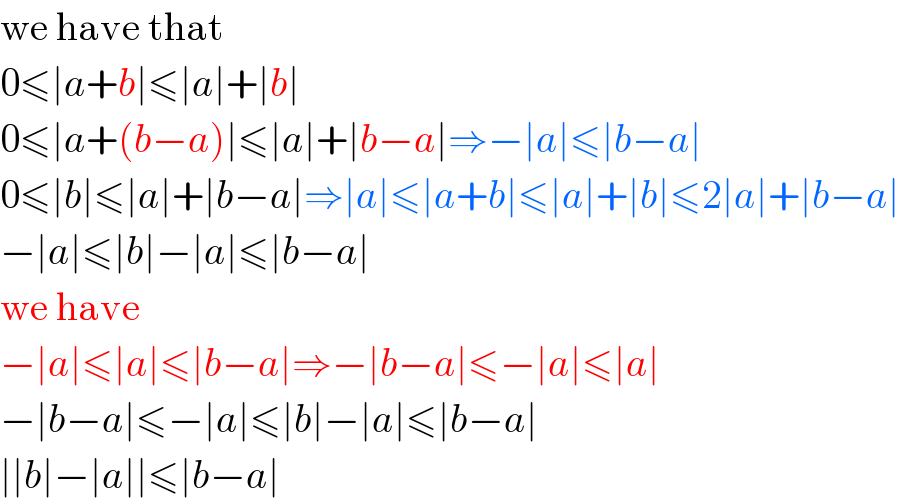
$$\mathrm{we}\:\mathrm{have}\:\mathrm{that} \\ $$$$\mathrm{0}\leqslant\mid{a}+{b}\mid\leqslant\mid{a}\mid+\mid{b}\mid \\ $$$$\mathrm{0}\leqslant\mid{a}+\left({b}−{a}\right)\mid\leqslant\mid{a}\mid+\mid{b}−{a}\mid\Rightarrow−\mid{a}\mid\leqslant\mid{b}−{a}\mid \\ $$$$\mathrm{0}\leqslant\mid{b}\mid\leqslant\mid{a}\mid+\mid{b}−{a}\mid\Rightarrow\mid{a}\mid\leqslant\mid{a}+{b}\mid\leqslant\mid{a}\mid+\mid{b}\mid\leqslant\mathrm{2}\mid{a}\mid+\mid{b}−{a}\mid \\ $$$$−\mid{a}\mid\leqslant\mid{b}\mid−\mid{a}\mid\leqslant\mid{b}−{a}\mid \\ $$$$\mathrm{we}\:\mathrm{have} \\ $$$$−\mid{a}\mid\leqslant\mid{a}\mid\leqslant\mid{b}−{a}\mid\Rightarrow−\mid{b}−{a}\mid\leqslant−\mid{a}\mid\leqslant\mid{a}\mid \\ $$$$−\mid{b}−{a}\mid\leqslant−\mid{a}\mid\leqslant\mid{b}\mid−\mid{a}\mid\leqslant\mid{b}−{a}\mid \\ $$$$\mid\mid{b}\mid−\mid{a}\mid\mid\leqslant\mid{b}−{a}\mid \\ $$
