
Question Number 152907 by mathdanisur last updated on 03/Sep/21

$$\mathrm{Find}\:\mathrm{a}\:\mathrm{closed}\:\mathrm{form}: \\ $$$$\Omega=\left(\underset{\:\mathrm{0}} {\overset{\:\mathrm{1}} {\int}}\:\frac{\mathrm{x}^{\mathrm{29}} −\mathrm{x}^{\mathrm{9}} }{\mathrm{x}^{\mathrm{40}} +\mathrm{1}}\:\mathrm{dx}\right)\left(\underset{\:\mathrm{0}} {\overset{\:\mathrm{1}} {\int}}\:\frac{\mathrm{x}^{\mathrm{29}} −\mathrm{2x}^{\mathrm{9}} }{\mathrm{x}^{\mathrm{40}} +\mathrm{4}}\mathrm{dx}\right) \\ $$
Answered by mindispower last updated on 03/Sep/21
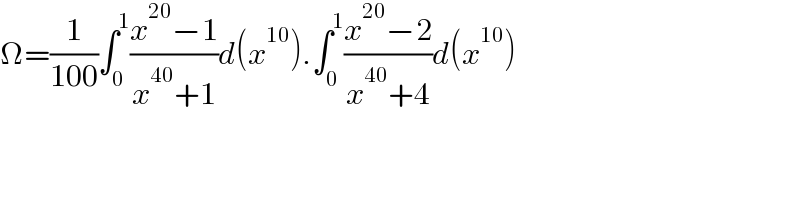
$$\Omega=\frac{\mathrm{1}}{\mathrm{100}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{20}} −\mathrm{1}}{{x}^{\mathrm{40}} +\mathrm{1}}{d}\left({x}^{\mathrm{10}} \right).\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{20}} −\mathrm{2}}{{x}^{\mathrm{40}} +\mathrm{4}}{d}\left({x}^{\mathrm{10}} \right) \\ $$$$ \\ $$
