
Question Number 15284 by ajfour last updated on 09/Jun/17
Commented by ajfour last updated on 09/Jun/17
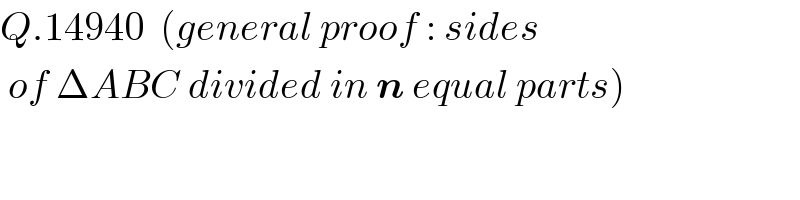
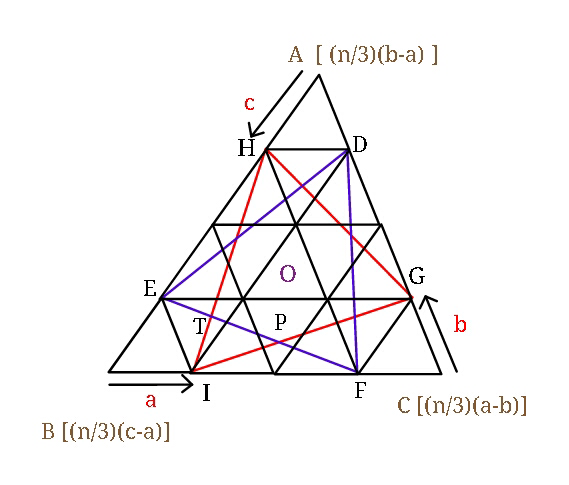
$${Q}.\mathrm{14940}\:\:\left({general}\:{proof}\::\:{sides}\:\right. \\ $$$$\left.\:{of}\:\Delta{ABC}\:{divided}\:{in}\:\boldsymbol{{n}}\:{equal}\:{parts}\right) \\ $$
Commented by RasheedSoomro last updated on 09/Jun/17

$$\mathrm{Sir}\:\mathrm{you}\:\mathrm{have}\:\mathrm{told}\:\mathrm{elsewhere}\:\mathrm{that}\:\mathrm{you} \\ $$$$\mathrm{use}\:\:{Lekh}\:{diagram}. \\ $$$${C}\mathrm{an}\:\mathrm{we}\:\mathrm{draw}\:\mathrm{scaled}\:\mathrm{figures}\:\mathrm{with}\:\mathrm{it}\:\mathrm{like} \\ $$$$\mathrm{geogebra}? \\ $$
Commented by ajfour last updated on 10/Jun/17

$${it}\:{is}\:{nothing}\:{compared}\:{to}\:{geogebra}, \\ $$$${i}\:{use}\:{these}\:{other}\:{apps}\:{coz}\:{my} \\ $$$${old}\:{and}\:{simple}\:{device}\:{dont}\:{support} \\ $$$${geogebra}\:{and}\:{many}\:{others}.. \\ $$
Answered by ajfour last updated on 09/Jun/17
![Origin: centroid of ΔABC of sides AB=nc, BC=na, AC=nb a+b+c=0 (vector sum) a×a=b×b=c×c=0 a×b=b×c=c×a=2t_0 ∀ t_0 is area of triangle witb sides a, b, and c . position vector of point B : r_B ^� =((1/2))((2/3))(−na)+((1/3))(nc) =(n/3)(c−a) . Eqn of line IG : r^� = OI+λ(IG) = r_B ^� +a+λ[(n−2)a−c] ...(i) Eqn of line EF : r^� = OE+μ(EF) = r_B ^� −c+μ[c+(n−1)a] ...(ii) P is intersection of IG and EF equating (ii) and (i) for point P : a[1+λ(n−2)−μ(n−1)]+ c[−λ+1−μ] =0 ⇒ λ(n−2)−μ(n−1)=−1 and λ+μ=1 or λ(n−1)+μ(n−1)=n−1 adding to get λ =((n−2)/(2n−3)) = ((IP)/(IG)) μ=1−(((n−2))/(2n−3)) = ((n−1)/(2n−3)) = ((ET)/(EF)) Eqn of HT : r^� = OI−ρ(HI) = (n/3)(c−a)+a−ρ[(n−1)c+a] Eqn of EF : r^� = (n/3)(c−a)−c+κ[(c+(n−1)a] equating them for point T : a[1−ρ−κ(n−1)]+c[−ρ(n−1)+1−κ]=0 ⇒ ρ+κ(n−1)=1 ....(iii) and ρ(n−1)+κ =1 or ρ(n−1)^2 +κ(n−1)=n−1 ..(iv) (iv)−(iii) gives: ρ=((n−2)/((n−1)^2 −1))=(1/n) =((TI)/(HI)) κ=1−ρ(n−1)=1−(((n−1))/n)=(1/n) =((ET)/(EF)) . Area(ΔTIP)=(IP×IT)/2 =((λ(IG)×(−ρ)(HI))/2) =((1/(2n)))(((n−2)/(2n−3)))[(n−2)a−c]×[−(n−1)c−a] =(((n−2))/(2n(2n−3)))[(n−1)(n−2)+1](c×a) =(((n−2)(n^2 −3n+3))/(n(2n−3))) t_0 Area(ΔIGH)=((IG×IH)/2) =(([(n−2)a−c]×[−a−(n−1)c])/2) =(([(n−1)(n−2)+1])/2)(c×a) = (n^2 −3n+3)t_0 . Area(considered hexagon) = Area(ΔIGH)−3Area(ΔTIP) =(n^2 −3n+3)t_0 −((3(n−2)(n^2 −3n+3)t_0 )/(n(2n−3))) =(n^2 −3n+3)[1−((3(n−2))/(n(2n−3)))]t_0 =(n^2 −3n+3)(((2n^2 −6n+6))/(n(2n−3)))t_0 = ((2(n^2 −3n+3)^2 )/(n(2n−3))) t_0 . Area(ΔABC)=((nc×na)/2) (n^2 /2)(c×a)=(n^2 /2)(2t_0 )=n^2 t_0 ((Area(hexagon))/(Area(ΔABC)))=((2(n^2 −3n+3)^2 )/(n^3 (2n−3))) .](Q15286.png)
$${Origin}:\:{centroid}\:{of}\:\Delta{ABC}\:{of} \\ $$$${sides}\:{AB}={nc},\:{BC}={na},\:{AC}={nb} \\ $$$$\:\boldsymbol{{a}}+\boldsymbol{{b}}+\boldsymbol{{c}}=\mathrm{0}\:\:\left({vector}\:{sum}\right) \\ $$$$\boldsymbol{{a}}×\boldsymbol{{a}}=\boldsymbol{{b}}×\boldsymbol{{b}}=\boldsymbol{{c}}×\boldsymbol{{c}}=\mathrm{0} \\ $$$$\boldsymbol{{a}}×\boldsymbol{{b}}=\boldsymbol{{b}}×\boldsymbol{{c}}=\boldsymbol{{c}}×\boldsymbol{{a}}=\mathrm{2}\boldsymbol{{t}}_{\mathrm{0}} \\ $$$$\forall\:\:{t}_{\mathrm{0}} \:{is}\:{area}\:{of}\:{triangle}\:{witb}\:{sides} \\ $$$$\boldsymbol{{a}},\:\boldsymbol{{b}},\:{and}\:\boldsymbol{{c}}\:. \\ $$$${position}\:{vector}\:{of}\:{point}\:{B}\:: \\ $$$$\bar {{r}}_{{B}} =\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\frac{\mathrm{2}}{\mathrm{3}}\right)\left(−{n}\boldsymbol{{a}}\right)+\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left({n}\boldsymbol{{c}}\right) \\ $$$$\:\:\:\:=\frac{{n}}{\mathrm{3}}\left(\boldsymbol{{c}}−\boldsymbol{{a}}\right)\:. \\ $$$${Eqn}\:{of}\:{line}\:{IG}\:: \\ $$$$\:\:\bar {\boldsymbol{{r}}}=\:{OI}+\lambda\left({IG}\right) \\ $$$$\:\:\:\:=\:\bar {\boldsymbol{{r}}}_{{B}} +\boldsymbol{{a}}+\lambda\left[\left({n}−\mathrm{2}\right)\boldsymbol{{a}}−\boldsymbol{{c}}\right]\:\:\:...\left({i}\right) \\ $$$${Eqn}\:{of}\:{line}\:{EF}\:: \\ $$$$\:\:\bar {\boldsymbol{{r}}}=\:{OE}+\mu\left({EF}\right) \\ $$$$\:\:\:\:=\:\bar {\boldsymbol{{r}}}_{{B}} −\boldsymbol{{c}}+\mu\left[\boldsymbol{{c}}+\left({n}−\mathrm{1}\right)\boldsymbol{{a}}\right]\:\:\:\:\:...\left({ii}\right) \\ $$$$\:\boldsymbol{{P}}\:\:{is}\:{intersection}\:{of}\:{IG}\:{and}\:{EF} \\ $$$$\:{equating}\:\left({ii}\right)\:{and}\:\left({i}\right)\:{for}\:{point}\:{P}\:: \\ $$$$\:\:\boldsymbol{{a}}\left[\mathrm{1}+\lambda\left({n}−\mathrm{2}\right)−\mu\left({n}−\mathrm{1}\right)\right]+ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{c}}\left[−\lambda+\mathrm{1}−\mu\right]\:=\mathrm{0} \\ $$$$\Rightarrow\:\lambda\left({n}−\mathrm{2}\right)−\mu\left({n}−\mathrm{1}\right)=−\mathrm{1}\:\:{and} \\ $$$$\:\:\:\:\:\:\:\:\:\:\lambda+\mu=\mathrm{1} \\ $$$${or}\:\:\lambda\left({n}−\mathrm{1}\right)+\mu\left({n}−\mathrm{1}\right)={n}−\mathrm{1} \\ $$$${adding}\:{to}\:{get}\:\lambda\:=\frac{{n}−\mathrm{2}}{\mathrm{2}{n}−\mathrm{3}}\:=\:\frac{{IP}}{{IG}} \\ $$$$\:\:\:\mu=\mathrm{1}−\frac{\left({n}−\mathrm{2}\right)}{\mathrm{2}{n}−\mathrm{3}}\:=\:\frac{{n}−\mathrm{1}}{\mathrm{2}{n}−\mathrm{3}}\:=\:\frac{{ET}}{{EF}} \\ $$$${Eqn}\:{of}\:{HT}\:: \\ $$$$\:\:\bar {\boldsymbol{{r}}}=\:{OI}−\rho\left({HI}\right) \\ $$$$\:\:\:\:=\:\frac{{n}}{\mathrm{3}}\left(\boldsymbol{{c}}−\boldsymbol{{a}}\right)+\boldsymbol{{a}}−\rho\left[\left({n}−\mathrm{1}\right)\boldsymbol{{c}}+\boldsymbol{{a}}\right] \\ $$$$\:{Eqn}\:{of}\:{EF}\:: \\ $$$$\:\:\bar {\boldsymbol{{r}}}=\:\frac{{n}}{\mathrm{3}}\left(\boldsymbol{{c}}−\boldsymbol{{a}}\right)−\boldsymbol{{c}}+\kappa\left[\left(\boldsymbol{{c}}+\left({n}−\mathrm{1}\right)\boldsymbol{{a}}\right]\right. \\ $$$${equating}\:{them}\:{for}\:{point}\:{T}\:: \\ $$$$\:\boldsymbol{{a}}\left[\mathrm{1}−\rho−\kappa\left({n}−\mathrm{1}\right)\right]+\boldsymbol{{c}}\left[−\rho\left({n}−\mathrm{1}\right)+\mathrm{1}−\kappa\right]=\mathrm{0} \\ $$$$\Rightarrow\:\:\rho+\kappa\left({n}−\mathrm{1}\right)=\mathrm{1}\:\:\:\:\:\:....\left({iii}\right)\:{and} \\ $$$$\:\:\:\:\:\:\:\rho\left({n}−\mathrm{1}\right)+\kappa\:=\mathrm{1} \\ $$$${or}\:\:\rho\left({n}−\mathrm{1}\right)^{\mathrm{2}} +\kappa\left({n}−\mathrm{1}\right)={n}−\mathrm{1}\:\:\:..\left({iv}\right) \\ $$$$\:\:\left({iv}\right)−\left({iii}\right)\:{gives}: \\ $$$$\:\rho=\frac{{n}−\mathrm{2}}{\left({n}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{1}}=\frac{\mathrm{1}}{{n}}\:\:=\frac{{TI}}{{HI}} \\ $$$$\:\:\kappa=\mathrm{1}−\rho\left({n}−\mathrm{1}\right)=\mathrm{1}−\frac{\left({n}−\mathrm{1}\right)}{{n}}=\frac{\mathrm{1}}{{n}} \\ $$$$\:\:\:\:\:=\frac{{ET}}{{EF}}\:\:. \\ $$$${Area}\left(\Delta{TIP}\right)=\left({IP}×{IT}\right)/\mathrm{2} \\ $$$$\:\:\:=\frac{\lambda\left({IG}\right)×\left(−\rho\right)\left({HI}\right)}{\mathrm{2}} \\ $$$$\:\:\:=\left(\frac{\mathrm{1}}{\mathrm{2}{n}}\right)\left(\frac{{n}−\mathrm{2}}{\mathrm{2}{n}−\mathrm{3}}\right)\left[\left({n}−\mathrm{2}\right)\boldsymbol{{a}}−\boldsymbol{{c}}\right]×\left[−\left({n}−\mathrm{1}\right)\boldsymbol{{c}}−\boldsymbol{{a}}\right] \\ $$$$\:\:=\frac{\left({n}−\mathrm{2}\right)}{\mathrm{2}{n}\left(\mathrm{2}{n}−\mathrm{3}\right)}\left[\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)+\mathrm{1}\right]\left(\boldsymbol{{c}}×\boldsymbol{{a}}\right) \\ $$$$\:\:=\frac{\left({n}−\mathrm{2}\right)\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)}{{n}\left(\mathrm{2}{n}−\mathrm{3}\right)}\:\boldsymbol{{t}}_{\mathrm{0}} \\ $$$${Area}\left(\Delta{IGH}\right)=\frac{{IG}×{IH}}{\mathrm{2}} \\ $$$$\:\:\:\:=\frac{\left[\left({n}−\mathrm{2}\right)\boldsymbol{{a}}−\boldsymbol{{c}}\right]×\left[−\boldsymbol{{a}}−\left({n}−\mathrm{1}\right)\boldsymbol{{c}}\right]}{\mathrm{2}} \\ $$$$\:\:\:=\frac{\left[\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)+\mathrm{1}\right]}{\mathrm{2}}\left(\boldsymbol{{c}}×\boldsymbol{{a}}\right) \\ $$$$\:\:\:=\:\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)\boldsymbol{{t}}_{\mathrm{0}} \:. \\ $$$${Area}\left({considered}\:{hexagon}\right) \\ $$$$\:\:\:\:=\:{Area}\left(\Delta{IGH}\right)−\mathrm{3}{Area}\left(\Delta{TIP}\right) \\ $$$$\:\:\:\:=\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)\boldsymbol{{t}}_{\mathrm{0}} −\frac{\mathrm{3}\left({n}−\mathrm{2}\right)\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)\boldsymbol{{t}}_{\mathrm{0}} }{{n}\left(\mathrm{2}{n}−\mathrm{3}\right)} \\ $$$$\:\:\:\:=\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)\left[\mathrm{1}−\frac{\mathrm{3}\left({n}−\mathrm{2}\right)}{{n}\left(\mathrm{2}{n}−\mathrm{3}\right)}\right]\boldsymbol{{t}}_{\mathrm{0}} \\ $$$$\:\:\:=\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)\frac{\left(\mathrm{2}{n}^{\mathrm{2}} −\mathrm{6}{n}+\mathrm{6}\right)}{{n}\left(\mathrm{2}{n}−\mathrm{3}\right)}\boldsymbol{{t}}_{\mathrm{0}} \\ $$$$\:\:\:=\:\frac{\mathrm{2}\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)^{\mathrm{2}} }{{n}\left(\mathrm{2}{n}−\mathrm{3}\right)}\:\boldsymbol{{t}}_{\mathrm{0}} \:\:. \\ $$$${Area}\left(\Delta{ABC}\right)=\frac{{n}\boldsymbol{{c}}×{n}\boldsymbol{{a}}}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{{n}^{\mathrm{2}} }{\mathrm{2}}\left(\boldsymbol{{c}}×\boldsymbol{{a}}\right)=\frac{{n}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{2}\boldsymbol{{t}}_{\mathrm{0}} \right)={n}^{\mathrm{2}} \boldsymbol{{t}}_{\mathrm{0}} \\ $$$$\frac{{Area}\left({hexagon}\right)}{{Area}\left(\Delta{ABC}\right)}=\frac{\mathrm{2}\left({n}^{\mathrm{2}} −\mathrm{3}{n}+\mathrm{3}\right)^{\mathrm{2}} }{{n}^{\mathrm{3}} \left(\mathrm{2}{n}−\mathrm{3}\right)}\:. \\ $$
Commented by mrW1 last updated on 09/Jun/17

$$\mathrm{Very}\:\mathrm{beautiful}\:\mathrm{work}\:\mathrm{sir}!\:\mathrm{congratulation}! \\ $$$$\mathrm{Since}\:\mathrm{you}\:\mathrm{have}\:\mathrm{the}\:\mathrm{points}\:\mathrm{T}\:\mathrm{and}\:\mathrm{P},\: \\ $$$$\mathrm{I}\:\mathrm{have}\:\mathrm{expected},\:\mathrm{that}\:\mathrm{you}\:\mathrm{will}\:\mathrm{calculate} \\ $$$$\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{hexagon}\:\mathrm{directly}\:\mathrm{in}\:\mathrm{this}\:\mathrm{way}: \\ $$$$\mathrm{A}_{\mathrm{Hexagon}} =\mathrm{6}×\mathrm{A}_{\Delta\mathrm{OTP}} \\ $$$$\mathrm{Can}\:\mathrm{you}\:\mathrm{check}\:\mathrm{if}\:\mathrm{this}\:\mathrm{will}\:\mathrm{get}\:\mathrm{the}\:\mathrm{same} \\ $$$$\mathrm{result},\:\mathrm{but}\:\mathrm{the}\:\mathrm{process}\:\mathrm{will}\:\mathrm{be}\:\mathrm{shorter}. \\ $$
Commented by ajfour last updated on 09/Jun/17

$${yes}\:{Sir},\:{completely}\:{true}. \\ $$$${I}\:{checked}\:{it}\:{through},\:{seems}\:{tedious} \\ $$$${again}\:{but}\:{they}\:{factorize}\:{out}. \\ $$$${Thanks}\:{for}\:{the}\:{genuine}\:{suggestion}. \\ $$
