
Question Number 150299 by otchereabdullai@gmail.com last updated on 10/Aug/21
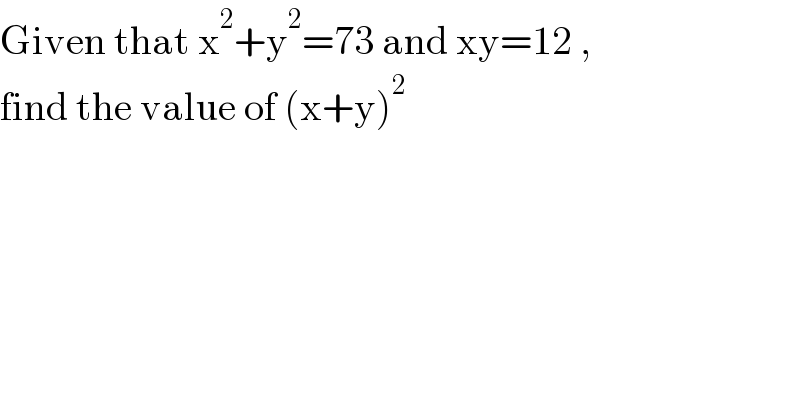
$$\mathrm{Given}\:\mathrm{that}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\mathrm{73}\:\mathrm{and}\:\mathrm{xy}=\mathrm{12}\:,\: \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\left(\mathrm{x}+\mathrm{y}\right)^{\mathrm{2}} \\ $$
Answered by cherokeesay last updated on 10/Aug/21

$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{2}{xy}=\left({x}+{y}\right)^{\mathrm{2}} =\mathrm{73}+\mathrm{24}=\mathrm{97} \\ $$
Commented by otchereabdullai@gmail.com last updated on 10/Aug/21

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}! \\ $$
Answered by puissant last updated on 11/Aug/21

$$\left(\mathrm{x}+\mathrm{y}\right)^{\mathrm{2}} =\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{2xy} \\ $$$$\Rightarrow\:\left(\mathrm{x}+\mathrm{y}\right)^{\mathrm{2}} =\mathrm{73}+\mathrm{24}=\mathrm{97}.. \\ $$
