
Question Number 144756 by mondlihk last updated on 28/Jun/21

Commented by Mathspace last updated on 28/Jun/21
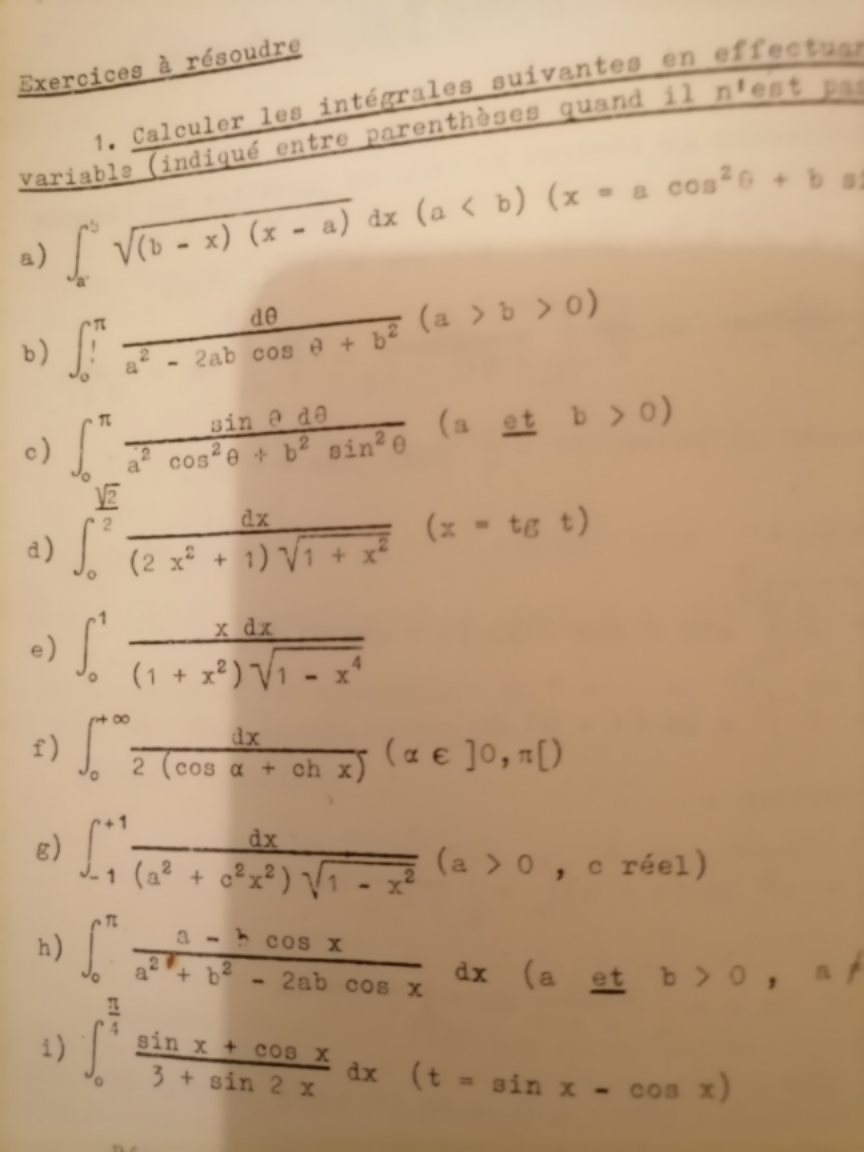
Answered by Olaf_Thorendsen last updated on 28/Jun/21
![Ω = ∫∫_(D = {x≥0, y≥0, x+y≤1}) xy dxdy Ω = ∫_0 ^1 ∫_0 ^(1−x) xy dxdy Ω = ∫_0 ^1 [x(y^2 /2)]_0 ^(1−x) dx Ω = ∫_0 ^1 x(((1−x)^2 )/2) dx Ω = [(x^4 /8)−(x^3 /3)+(x^2 /4)]_0 ^1 = ((3−8+6)/(24)) = (1/(24))](Q144758.png)
$$\Omega\:=\:\int\int_{\mathcal{D}\:=\:\left\{{x}\geqslant\mathrm{0},\:{y}\geqslant\mathrm{0},\:{x}+{y}\leqslant\mathrm{1}\right\}} {xy}\:{dxdy} \\ $$$$\Omega\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \int_{\mathrm{0}} ^{\mathrm{1}−{x}} {xy}\:{dxdy} \\ $$$$\Omega\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \left[{x}\frac{{y}^{\mathrm{2}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\mathrm{1}−{x}} \:{dx} \\ $$$$\Omega\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} {x}\frac{\left(\mathrm{1}−{x}\right)^{\mathrm{2}} }{\mathrm{2}}\:{dx} \\ $$$$\Omega\:=\:\left[\frac{{x}^{\mathrm{4}} }{\mathrm{8}}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{2}} }{\mathrm{4}}\right]_{\mathrm{0}} ^{\mathrm{1}} \:=\:\frac{\mathrm{3}−\mathrm{8}+\mathrm{6}}{\mathrm{24}}\:=\:\frac{\mathrm{1}}{\mathrm{24}} \\ $$
